2013E试卷(澳大利亚数学竞赛AMC-E：11-12年级中英文历年真题)

格式：pdf
大小：996.21 KB
文档页数：9

下载文档原格式

AMC Senior 2008(澳大利亚数学竞赛AMC-E：11-12年级中英文历年真题)

A u s t r A l i A n M At h e M At i c s c o M p e t i t i o na n a c t i v it y o f t h e a u s t r a l i a n m a t h e m a t i c s t r u s tT H U R S D AY 31 J U LY 2008SENIOR DIVISION COMPETITION PAPERINSTRUCTIO NS AND INFO RMATIO NGENERAL1. Do not open the booklet until told to do so by your teacher.2. NO calculators, slide rules, log tables, maths stencils, mobile phones or other calculating aids arepermitted. Scribbling paper, graph paper, ruler and compasses are permitted, but are not essential. 3. Diagrams are NOT drawn to scale. They are intended only as aids.4. There are 25 multiple-choice questions, each with 5 possible answers given and 5 questions thatrequire a whole number between 0 and 999. The questions generally get harder as you work through the paper. There is no penalty for an incorrect response.5. This is a competition not a test; do not expect to answer all questions. You are only competingagainst your own year in your own State or Region so different years doing the same paper are not compared.6. Read the instructions on the Answer Sheet carefully. Ensure your name, school name and schoolyear are filled in. It is your responsibility that the Answer Sheet is correctly coded. 7. When your teacher gives the signal, begin working on the problems.THE ANSWER SHEET 1. Use only lead pencil.2. Record your answers on the reverse of the Answer Sheet (not on the question paper) by FULLYcolouring the circle matching your answer.3. Your Answer Sheet will be read by a machine. The machine will see all markings even if they arein the wrong places, so please be careful not to doodle or write anything extra on the Answer Sheet. If you want to change an answer or remove any marks, use a plastic eraser and be sure to remove all marks and smudges.INTEGRITY OF THE COMPETITIONThe AMC reserves the right to re-examine students before deciding whether to grant official status to their score.A U S T R A L I A N S C H O O L Y E A R S 11 A N D 12T I M E A L L O W E D : 75 M I N U T E SSenior DivisionQuestions 1to 10,3marks each1.The value of 8002−2008is (A)200(B)8(C)6006(D)1060(E)59942.The diﬀerence between 120and 210is (A)0(B)110(C)35(D)310(E)3203.In the diagram,x equals................................................................................................................................................................................ (x)◦100◦110◦80◦(A)100(B)110(C)120(D)130(E)1404.The value of 200×8200÷8is(A)1(B)8(C)16(D)64(E)2005.The smallest value that x 2−4x +3can have is (A)−1(B)−3(C)1(D)3(E)26.$3is shared between two people.One gets 50cents more than the other.The ratio of the larger share to the smaller share is (A)6:1(B)7:5(C)4:3(D)5:3(E)7:4S 27.When 10002008is written as a numeral,the number of digits written is (A)2009(B)6024(C)6025(D)8032(E)20128.A semicircle is drawn on one side of an equilateral triangle.The ratio of the area of the semicircle to the area of the triangle is(A)1:1(B)π:2√3(C)π:√3(D)√3:π(E)3:π................................................................................................................................................................................................................................................................................................................................................................................................................................................................9.Given that cos x =0.5and 0◦<x <90◦,which of the following has the greatestvalue?(A)cos 2x(B)cos x(C)0.75(D)sin x(E)tan x10.A ﬁshtank with base 100cm by 200cm and depth 100cm contains water to a depthof 50cm.A solid metal rectangular prism with dimensions 80cm by 100cm by 60cm is then submerged in the tank with an 80cm by 100cm face on the bottom..................................................................................................................6.......................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................100100200501006080The depth of water,in centimetres,above the prism is then (A)12(B)14(C)16(D)18(E)20Questions 11to 20,4marks each11.Which of the following numbers is the largest?(A)2500(B)3400(C)4300(D)5200(E)610012.A normal die is thrown 100times.The sum of the numbers obtained will mostlikely be(A)200(B)250(C)300(D)350(E)400S 313.What is the smallest whole number which gives a square number when multipliedby 2008?(A)2(B)4(C)251(D)502(E)200814.A cross is made up of ﬁve squares,each with side length 1unit.Two cuts aremade,the ﬁrst from X to Y and the second from Z to T ,so that ZT X is a right angle.The three pieces are then arranged to form a rectangle...................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................ZYX T IIIIII.................................................................... (II)IIIIWhat is the ratio of the length to the width of the rectangle?(A)3:1(B)√10:1(C)2:1(D)2√3:1(E)5:215.A function is said to be a toggle function on (p,q,r )if f (p )=q ,f (q )=r andf (r )=p .The function f (x )=ax 2+bx +c is a toggle function on (1,2,3).What is the value of c ?(A)−2(B)0(C)3(D)9(E)1416.Two conical rollers with perpendicu-lar axes touch on a line that is 30◦to the axis of the smaller roller and 60◦to the axis of the larger roller.If the larger roller makes 1revolution per sec-ond and there is no slipping,how many revolutions per second does the smaller roller make?(A)12(B)1(C)√2(D)√3(E)2...................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................60◦30◦S 417.Consider the set X ={1,2,3,4,5,6}.How many subsets of X ,with at least one element,do not contain two consecutive integers?(A)16(B)18(C)20(D)21(E)2418.Farmer Taylor of Burra has two tanks.Water from the roof of his farmhouse iscollected in a 100kL tank and water from the roof of his barn is collected in a 25kL tank.The collecting area of his farmhouse roof is 200square metres while that of his barn is 80square metres.Currently,there are 35kL in the farmhouse tank and 13kL in the barn tank.Rain is forecast and he wants to collect as much water as possible.He should:(A)empty the barn tank into the farmhouse tank (B)ﬁll the barn tank from the farmhouse tank(C)pump 10kL from the farmhouse tank into the barn tank (D)pump 10kL from the barn tank into the farmhouse tank (E)do nothing19.A sequence {u 1,u 2,...,u n }of real numbers is deﬁned byu 1=√2,u 2=π,u n =u n −1−u n −2forn ≥3.What is u 2008?(A)−√2(B)2008(√2−2008π)(C)1003√2−1004π(D)π(E)√220.In the diagram,RU is equal in lengthto ST .What is the ratio of the area of QRU to the area of QST ?(A)√3:1(B)2:1(C)√6:1(D)√3:2(E)√6:2......................................................................................................................................................................................................................... (45)◦30◦UT Q RSQuestions 21to 25,5marks each21.P ,Q ,R ,S and T are consecutive vertices of a regular polygon.When extended,the lines P Q and T S meet at U with QUS =160◦.How many sides has the polygon?(A)36(B)42(C)48(D)52(E)54S522.How many numbers from1,2,3,4,...,2008have a cubic number other than1asa factor?(A)346(B)336(C)347(D)251(E)39323.The numbers828and313are3-digit palindromes where828−313=515,whichis also a palindrome.How many pairs(a,b)of3-digit palindromes are there witha>b and with a−b also a3-digit palindrome?(A)1972(B)1980(C)1988(D)1996(E)200824.The centres of all faces of a cube are joined to form an octahedron.The centresof all faces of this octahedron are now joined to form a smaller cube.What is the ratio of an edge of the smaller cube to an edge of the original cube?(A)1:√2(B)1:√3(C)1:2(D)1:3(E)1:425.In theﬁgure,all line segments are par-allel to one of the sides of the equi-lateral triangle P QR which has sidelength1unit.How long should P Xbe to maximise the smallest of the tenareas deﬁned?(A)13(B)4−√214(C)14(D)15(E)1√10.......................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................PQ RXFor questions26to30,shade the answer as an integer from0to999inthe space provided on the answer sheet.Question26is6marks,question27is7marks,question28is8marks, question29is9marks and question30is10marks.26.All possible straight lines joining the vertices of a cube with mid-points of its edgesare drawn.At how many points inside the cube do two or more of these lines meet?S 627.Let us call a sum of integers cool if the ﬁrst and last terms are 1and each termdiﬀers from its neighbours by at most 1.For example,the sum 1+2+3+4+3+2+3+3+3+2+3+3+2+1is cool.How many terms does it take to write 2008as a cool sum if we use no more terms than necessary?28.The positive integers x and y satisfy3x 2−8y 2+3x 2y 2=2008.What is the value of xy ?29.A point O is inside an equilateral triangleP QR and the perpendiculars OL ,OM and ON are drawn to the sides P Q ,QR and RP respectively.The ratios of lengths of the perpendiculars OL :OM :ON is 1:2:3.If area of LONP area of P QR =a b,where a and b are integers with no common factors,what is the value of a +b ?...............................................................................................................................................................................................................................................................................................................................................................................................................................................RPQLMNO.......................................................................................................................................................................................................................................................................................30.What is the smallest value that49+a 2−7√2a + a 2+b 2−√2ab +√50+b 2−10bcan have for positive real numbers a and b ?***Senior 2008 Answers Question Answer 1E2E3D4D5A6B7C8B9E10B11B12D13D14C15A16D17C18D19A20D21E22B23B24D25C26142789282829473013。

2013澳大利亚高考数学试题评分标准

3 ⌠ = 2π ⎡ 4e x ⎤ − 2π ⎮ xe x dx ⎣ ⎦1 ⌡ 1 3 3
3
Using integration by parts:
3 ⌠ 3 ⌠ x x xe dx = ⎡ xe ⎤ − ⎮ e x dx ⎮ ⎣ ⎦1 ⌡ ⎮ 1 ⌡1 3
(
)
= [ xe x − e x ]1
3
= ( 3e3 − e3 ) − ( e − e ) = 3e3 − e3 = 2e3 Hence V = 8π ( e3 − e ) − 2π 2e3 = 8π e3 − e − 4π e3 = 4π e3 − 8π e ∴ Volume is 4π e e 2 − 2 units3
z = x + iy ( x , y real ) z 2 + z 2 = ( x + iy ) + ( x − iy )
2 2
Marks 3 2 1
= x 2 + 2ixy − y 2 + x 2 − 2ixy − y 2 = 2 x 2 − 2 y2 = 2 x 2 − y2
(
)
x 2 y2 − ≤ 1. 4 4
= ⎮ cos2 θ (1 − cos2 θ ) sin θ dθ ⌡0 = ⎮ cos2 θ sin θ − cos4 θ sin θ dθ ⌡0 ⎡ cos3 θ = ⎢− + 3 ⎣
π 5 ⎤2 cos θ π ⌠2 π ⌠2 ππ Nhomakorabea5
⎥ ⎦0
⎡ 1 1⎤ = 0 − ⎢− + ⎥ ⎣ 3 5⎦ = 2 15
First use the t -substition, t = tan Then dx = 2

VCE考试数学2013试题1

Question 7 (6 marks) The probability distribution of a discrete random variable, X, is given by the table below. x Pr(X = x) a. 0 0.2 1 0.6p2 2 0.1 3 1-p 4 0.1 3 marks
b.
On the axes above, sketch the graph of g(x) = | f (x)| + 1, for x [-2, 2]. Label the end points with their coordinates.
2 marks
Question 9 – continued
6
Question 6 (3 marks) Let g: R → R, g(x) = (a – x)2, where a is a real constant. 31 The average value of g on the interval [–1, 1] is . 12 Find all possible values of a.
-x
y = f (x) P (x, f (x))
O
Q
x
a.
Find the area, A, of the triangle OQP in terms of x.
1 mark
Question 10 – continued
13
2013 MATHMETH (CAS) EXAM 1
b.
Find the maximum area of triangle OQP and the value of x for which the maximum occurs.

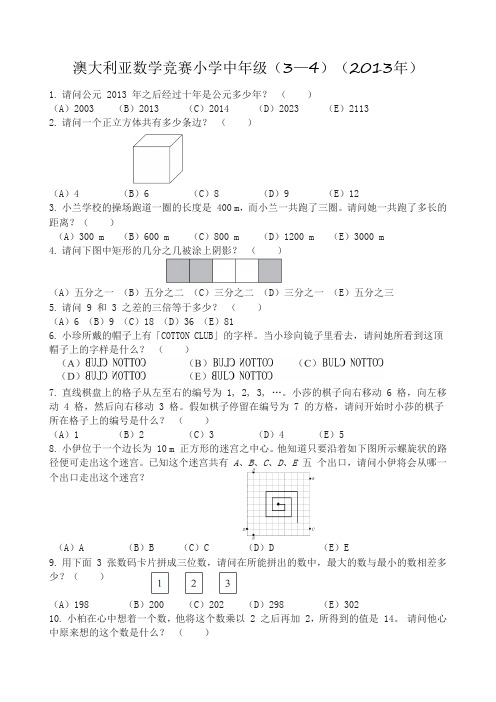
澳大利亚数学竞赛小学中年级(3--4年级)(2013年)

澳大利亚数学竞赛小学中年级（3—4）（2013年）1.请问公元 2013 年之后经过十年是公元多少年？（）（A）2003 （B）2013 （C）2014 （D）2023 （E）21132.请问一个正立方体共有多少条边？（）（A）4 （B）6 （C）8 （D）9 （E）123.小兰学校的操场跑道一圈的长度是 400 m，而小兰一共跑了三圈。

请问她一共跑了多长的距离？（）（A）300 m （B）600 m （C）800 m （D）1200 m （E）3000 m4.请问下图中矩形的几分之几被涂上阴影？（）（A）五分之一（B）五分之二（C）三分之二（D）三分之一（E）五分之三5.请问 9 和 3 之差的三倍等于多少？（）（A）6 （B）9 （C）18 （D）36 （E）816.小珍所戴的帽子上有「COTTON CLUB」的字样。

当小珍向镜子里看去，请问她所看到这顶帽子上的字样是什么？（）7.直线棋盘上的格子从左至右的编号为 1, 2, 3, …。

小莎的棋子向右移动 6 格，向左移动 4 格，然后向右移动 3 格。

假如棋子停留在编号为 7 的方格，请问开始时小莎的棋子所在格子上的编号是什么？（）（A）1 （B）2 （C）3 （D）4 （E）58.小伊位于一个边长为 10 m 正方形的迷宫之中心。

他知道只要沿着如下图所示螺旋状的路径便可走出这个迷宫。

已知这个迷宫共有A、B、C、D、E 五个出口，请问小伊将会从哪一个出口走出这个迷宫？（A）A （B）B （C）C （D）D （E）E9.用下面 3 张数码卡片拼成三位数，请问在所能拼出的数中，最大的数与最小的数相差多少？（）（A）198 （B）200 （C）202 （D）298 （E）30210.小柏在心中想着一个数，他将这个数乘以 2 之后再加 2，所得到的值是 14。

请问他心中原来想的这个数是什么？（）（A）6 （B）7 （C）8 （D）12 （E）3011.小艾有两枚 50 元硬币、三枚 20 元硬币与八枚 5 元硬币，小德有四枚 20 元硬币与六枚 10 元硬币。

2013年AMC8竞赛真题(英文版)

2013 AMC8 Problems1.Danica wants to arrange her model cars in rows with exactly 6 cars in each row. She now has 23 model cars. What is the smallest number of additional cars she must buy in order to be able to arrange all her cars this way?2.A sign at the fish market says, "50% off, today only: half-pound packages for just $3 perpackage." What is the regular price for a full pound of fish, in dollars?What is the value of?3.4.Eight friends ate at a restaurant and agreed to share the bill equally. Because Judi forgot her money, each of her seven friends paid an extra $2.50 to cover her portion of the total bill. What was the total bill? 5.Hammie is in thegrade and weighs 106 pounds. His quadruplet sisters are tiny babiesand weigh 5, 5, 6, and 8 pounds. Which is greater, the average (mean) weight of these five children or the median weight, and by how many pounds?6.The number in each box below is the product of the numbers in the two boxes that touch it in the row above. For example, . What is the missing number in the top row?7.Trey and his mom stopped at a railroad crossing to let a train pass. As the train began to pass, Trey counted 6 cars in the first 10 seconds. It took the train 2 minutes and 45 seconds to clear the crossing at a constant speed. Which of the following was the most likely number of cars in the train?8.A fair coin is tossed 3 times. What is the probability of at least two consecutive heads?9.The Incredible Hulk can double the distance he jumps with each succeeding jump. If his first jump is 1 meter, the second jump is 2 meters, the third jump is 4 meters, and so on, then on which jump will he first be able to jump more than 1 kilometer?10.What is the ratio of the least common multiple of 180 and 594 to the greatest common factor of 180 and 594?11.Ted's grandfather used his treadmill on 3 days this week. He went 2 miles each day. On Monday he jogged at a speed of 5 miles per hour. He walked at the rate of 3 miles per hour on Wednesday and at 4 miles per hour on Friday. If Grandfather had always walked at 4 miles per hour, he would have spent less time on the treadmill. How many minutes less?12.At the 2013 Winnebago County Fair a vendor is offering a "fair special" on sandals. If you buy one pair of sandals at the regular price of $50, you get a second pair at a 40% discount, and a third pair at half the regular price. Javier took advantage of the "fair special" to buy three pairs of sandals. What percentage of the $150 regular price did he save?13.When Clara totaled her scores, she inadvertently reversed the units digit and the tens digit of one score. By which of the following might her incorrect sum have differed from the correct one?14.Abe holds 1 green and 1 red jelly bean in his hand. Bea holds 1 green, 1 yellow, and 2 red jelly beans in her hand. Each randomly picks a jelly bean to show the other. What is the probability that the colors match?15.If , , and , what is the product of , , and ?16.A number of students from Fibonacci Middle School are taking part in a community serviceproject. The ratio of -graders to -graders is , and the the ratio of -graders to-graders is . What is the smallest number of students that could be participating in the project?17.The sum of six consecutive positive integers is 2013. What is the largest of these six integers?18.Isabella uses one-foot cubical blocks to build a rectangular fort that is 12 feet long, 10 feet wide, and 5 feet high. The floor and the four walls are all one foot thick. How many blocks does the fort contain?19.Bridget, Cassie, and Hannah are discussing the results of their last math test. Hannah shows Bridget and Cassie her test, but Bridget and Cassie don't show theirs to anyone. Cassie says, 'I didn't get the lowest score in our class,' and Bridget adds, 'I didn't get the highest score.' What is the ranking of the three girls from highest to lowest?20.A rectangle is inscribed in a semicircle with longer side on the diameter. What is thearea of the semicircle?21.Samantha lives 2 blocks west and 1 block south of the southwest corner of City Park. Her school is 2 blocks east and 2 blocks north of the northeast corner of City Park. On school days she bikes on streets to the southwest corner of City Park, then takes a diagonal path through the park to the northeast corner, and then bikes on streets to school. If her route is as short as possible, how many different routes can she take?22.Toothpicks are used to make a grid that is 60 toothpicks long and 32 toothpicks wide. How many toothpicks are used altogether?23.Angle of is a right angle. The sides of are the diameters of semicirclesas shown. The area of the semicircle on equals , and the arc of the semicircle onhas length . What is the radius of the semicircle on ?24.Squares , , and are equal in area. Points and are the midpointsof sides and , respectively. What is the ratio of the area of the shaded pentagonto the sum of the areas of the three squares?25.A ball with diameter 4 inches starts at point A to roll along the track shown. The track iscomprised of 3 semicircular arcs whose radii are inches, inches, andinches, respectively. The ball always remains in contact with the track and does notslip. What is the distance the center of the ball travels over the course from A to B?。

澳大利亚数学竞赛小学高年级(5—6)(2013年)

澳大利亚数学竞赛小学高年级（5—6）（2013年）1.请问下图中矩形的几分之几被涂上阴影？（）（A）五分之一（B）五分之二（C）三分之二（D）三分之一（E）五分之三2. 请问下列哪一个数最接近 0？（）（A）0.03 （B）0.048 （C）0.009 （D）0.005 （E）0.023.一架波音 737 飞机的中间走道两侧每排各有 3 个座位。

若这架飞机150 位乘客，请问它的座位共有多少排？（）（A）50 （B）37 （C）33 （D）32 （E）254.小艾有两枚 50 元硬币、三枚 20 元硬币与八枚 5 元硬币，小德有四枚 2硬币与六枚 10 元硬币。

请问小艾的钱比小德的钱多了多少元？（）（A）40 元（B）60 元（C）80 元（D）140 元（E）2005.用下面 5 张数码卡片拼成五位数，请问在所能拼出的数中，最大的数与的数相差多少？（）（A）41967 （B）41976 （C）44444 （D）42024 （E）410766.在超市中，正常包装的薯条每包重量为 75 g。

有一种促销包，每包薯条量增加三分之一。

请问此促销包每包的重量是多少g？（）（A）50 （B）78 （C）100 （D）125 （E）1507. 请问下图中共有多少个不同位置的三角形？（）（A）9 （B）10 （C）13 （D）14 （E）178.小珍将一个数乘以2 后再加 2，接着再将所得的数除以 2 后再减 2，最所得到的结果是 6。

请问小珍原来的这个数是什么？（）（A ）1 （B ）6 （C ）7 （D ）14 （E ）169. 在下面的数线上，请问31应该位于哪里？（）（A ）在 0 与 0.3 之间；（B ）在 0.3 与 0.4 之间；（B ）在 0.4 与 0.7 之间（D ）在 0.7 与 0.8 之间；（D ）在 0.8 与 1 之间。

10. 下图中每个三角形都是正三角形。

请问阴影部分的面积占最大三角形面几分之几？（）（A ）41 （B ）6415 （C ）31 （D ）163 （E ） 327 11. 在下面的算式中，三个数码已经被□取代。

AMC Intermediate 2013AMC-D：9-10年级中文历年真题

2013INTERMEDIATE DIVISIONAUSTRALIAN S CHOOL YEARS 9 and 10TIME ALLOWED: 75 MINUTESINSTRUCTIONS AND INFORMATIONGENERAL1. Do not open the booklet until told to do so by your teacher.2. NO calculators, slide rules, log tables, maths stencils, mobile phones or other calculating aids are permitted.Scribbling paper, graph paper, ruler and compasses are permitted, but are not essential. 3. Diagrams are NOT drawn to scale. They are intended only as aids.4. There are 25 multiple-choice questions, each with 5 possible answers given and 5 questions that require awhole number answer between 0 and 999. The questions generally get harder as you work through the paper. There is no penalty for an incorrect response.5. This is a competition not a test; do not expect to answer all questions. You are only competing against yourown year in your own country or Australian state so different years doing the same paper are not compared. 6. Read the instructions on the answer sheet carefully. Ensure your name, school name and school year areentered. It is your responsibility to correctly code your answer sheet. 7. When your teacher gives the signal, begin working on the problems.THE ANSWER SHEET 1. Use only lead pencil.2. Record your answers on the reverse of the answer sheet (not on the question paper) by FULLY colouring thecircle matching your answer.3. Your answer sheet will be scanned. The optical scanner will attempt to read all markings even if they are inthe wrong places, so please be careful not to doodle or write anything extra on the answer sheet. If you want to change an answer or remove any marks, use a plastic eraser and be sure to remove all marks and smudges.INTEGRITY OF THE COMPETITIONThe AMT reserves the right to re-examine students before deciding whether to grant official status to their score.©AMT P ublishing 2013 AMTT liMiTed Acn 083 950 341A ustrAliAn M AtheMAtics c oMpetitionsponsored by the c oMMonweAlth b AnkAn AcTiviTy of The AusTrAliAn MATheMATics TrusTNAMEYEAR TEACHERA u s T r A l i A n M A T h e M A T i c s T r u s TIntermediate DivisionQuestions1to10,3marks each1.2013+2014+2015equals(A)642(B)2016(C)6022(D)6032(E)60422.In the diagram below,what is the value,in degrees,of angle x?37◦85◦x◦(A)48(B)85(C)122(D)132(E)1433.If every digit of a whole number is either a3or a5,the number will always be(A)divisible by3(B)divisible by5(C)prime(D)even(E)odd4.The average of two numbers is twice the smaller number.The larger number is12.What is the smaller number?(A)2(B)3(C)4(D)6(E)85.The length of the base of a triangle is3times its perpendicular height and the areaof the triangle is24cm2.The sum of its base length and its perpendicular height, in centimetres,is(A)12(B)13(C)14(D)15(E)166.A regular icosahedron is a solid shape with twenty faces,where each face is directlyopposite another face.I label the faces from1to20so that,for all pairs of opposite faces,the two labels in any pair always add up to the same number.What number is on the face opposite the one labelled8?(A)11(B)12(C)13(D)14(E)157.If p=4b+26and b is a positive integer,then p could not be divisible by(A)2(B)4(C)5(D)6(E)78.My two dogs were running on the beach when I called them back.The faster dogwas100m away and the slower dog was70m away.The faster dog runs twice as fast as the slower dog.How far away was the second dog when theﬁrst dog reached me?(A)15m(B)20m(C)30m(D)40m(E)50m9.The value of x2+1x2when x=23is closest to(A)0(B)1(C)2(D)3(E)410.A piece of paper in the shape of an equilateral triangle has one corner folded over,as shown.What is the value of x?(A)60(B)70(C)80(D)90(E)100Questions11to20,4marks each11.Start with the number1and create the sequence1,2,4,8,16,22,24,28,...where each number is the sum of the previous number and itsﬁnal digit.How many numbers in the sequence are less than1000?(A)10(B)100(C)101(D)200(E)20112.A six-sided dice has the numbers1,2,2,3,3and3on its faces.Two such dice arerolled and a score is made by adding the numbers on the uppermost faces.The probability of rolling an odd score is(A)19(B)29(C)13(D)49(E)5913.If x2=x+3,then x3equals(A)x+6(B)2x+6(C)3x+9(D)4x+3(E)27x+914.The point T divides the side QR of the rectangle P QRS into two equal segments.The point U divides P Q such that P U:UQ=1:2.Point V divides SP such that SV:V P=1:3andﬁnally,point W divides RS such that RW:W S=1:4.Find the area of the quadrilateral T UV W if the area of P QRS equals120.V(A)67(B)70(C)72(D)75(E)7715.Three line segments of lengths1,a and2a are the sides of a triangle.Which ofthe following deﬁnes all possible values of a?(A)13<a<1(B)0<a<13(C)a<1(D)for all a>0(E)for no a16.The shaded segment in the circle below,centre O,has anarea of1cm2.The radiusof the circle,in centimetres,is(A) 4π(B)8π(C) 4π−2(D)4π(E)2√π17.Dan and Jane each have a measuring tape of length1m.Dan’s tape got stuck ina door and was extended by4cm.Jane left her tape in a pocket and it shrank by5cm after washing.However,the centimetre marks on both tapes remained evenly distributed.Measuring the schoolyard,Dan noted the length as23.75m.What length will Jane get measuring the same schoolyard with her tape?(A)23m(B)24m(C)25m(D)26m(E)27m18.In the regular hexagon pictured,the midpoints of the sides are joined to form theshaded regular hexagon.What fraction of the larger hexagon is shaded?(A)34(B)23(C)56(D)12(E)7819.A circular wheel of radius r rolls,without slipping,through half a revolution.Thepoint X is on the horizontal diameter at the start.XThe distance between the starting andﬁnishing position of the point X is(A)2πr(B)(π+2)r(C)(π−2)r(D)2(π+1)r(E)2(π−1)r20.The sport of bingbong involves two players.Each match consists of a number ofrounds and each round consists of a number of points.Theﬁrst player to win four points in a round wins the round.Theﬁrst player to win six rounds in a match wins the match.Suppose that after a match of bingbong,the winner has won W points while the loser has won L points.What is the largest possible value of L−W?(A)−6(B)−4(C)0(D)12(E)14Questions21to25,5marks each21.In how many ways can the numbers1,2,3,4,5,6be arranged in a row so thatthe product of any two adjacent numbers is even?(A)64(B)72(C)120(D)144(E)72022.Two circles,one of radius1and the other of radius2,touch externally at P.Astraight line through P cuts the area formed by these two circles in the ratio1:2.In what ratio does this line cut the area of the smaller circle?(A)1:2(B)2:5(C)1:3(D)2:7(E)1:423.How many positive integers n are there such that2n+1is a divisor of8n+46?(A)0(B)1(C)2(D)3(E)424.The rectangle P QRS shown has P Q=4,P S=12and centre C.The two shadedcircles have radius1and touch P S at U and V where P U=1and P V=4.The line CW divides the unshaded area in half.The length of P W isW(A)27(B)25(C)14(D)13(E)1225.In3013,King Warren of Australia isﬁnally deposed.Theﬁve remaining earls argueabout which one of them will be king,and which one of the others will be treasurer.Akaroa will be satisﬁed only if Darlinghurst or Erina is treasurer.Bairnsdale will be satisﬁed only if Claremont is treasurer.Claremont will be satisﬁed only if Darlinghurst is either king or treasurer.Darlinghurst will be satisﬁed only if Akaroa is either king or treasurer.Erina will be satisﬁed only if Akaroa is not king.It is not possible for allﬁve to be satisﬁed,so in the end they appoint king and treasurer so that the other three earls are satisﬁed.Who becomes king?(A)Akaroa(B)Bairnsdale(C)Claremont(D)Darlinghurst(E)ErinaFor questions26to30,shade the answer as an integer from0to999inthe space provided on the answer sheet.Question26is6marks,question27is7marks,question28is8marks, question29is9marks and question30is10marks.26.The4-digit number pqrs has the property that pqrs×4=srqp.If p=2,what isthe value of the3-digit number qrs?27.Three diﬀerent non-zero digits are used to form six diﬀerent3-digit numbers.Thesum ofﬁve of them is3231.What is the sixth number?28.A hockey game between two teams is‘relatively close’if the number of goals scoredby the two teams never diﬀer by more than two.In how many ways can theﬁrst 12goals of a game be scored if the game is‘relatively close’?29.How many pairs(a,b)of positive integers are there such that a and b are factorsof66and a is a factor of b?30.All the digits of the positive integer N are either0or1.The remainder afterdividing N by37is18.What is the smallest number of times that the digit1can appear in N?Intermediate 2013 Answers Question Answer 1E2C3E4C5E6C7B8B9D10C11E12D13D14A15A16C17D18A19B20E21D22D23D24A25B26178277652897229784305。

AMC Senior 2011(澳大利亚数学竞赛AMC-E：11-12年级中英文历年真题)

A u s t r A l i A n M At h e M At i c s c o M p e t i t i o na n a c t i v i t y o f t h e a u s t r a l i a n m a t h e m a t i c s t r u s tt h u r sd ay4a u g u s t2011senior Division Competition papera u st r a l i a n s c h o o l y e a r s11a n d12t i m e a l l o w e d:75m i n u t e sinstruCtions anD informationGeneraL1. Do not open the booklet until told to do so by your teacher.2. NO calculators, slide rules, log tables, maths stencils, mobile phones or other calculating aids arepermitted. Scribbling paper, graph paper, ruler and compasses are permitted, but are not essential.3. Diagrams are NOT drawn to scale. They are intended only as aids.4. There are 25 multiple-choice questions, each with 5 possible answers given and 5 questions thatrequire a whole number answer between 0 and 999. The questions generally get harder as you work through the paper. There is no penalty for an incorrect response.5. This is a competition not a test; do not expect to answer all questions. You are only competingagainst your own year in your own State or Region so different years doing the same paper are not compared.6. Read the instructions on the answer sheet carefully. Ensure your name, school name and schoolyear are entered. It is your responsibility to correctly code your answer sheet.7. When your teacher gives the signal, begin working on the problems.tHe ansWer sHeet1. Use only lead pencil.2. Record your answers on the reverse of the answer sheet (not on the question paper) by FULLYcolouring the circle matching your answer.3. Your answer sheet will be scanned. The optical scanner will attempt to read all markings evenif they are in the wrong places, so please be careful not to doodle or write anything extra on the answer sheet. If you want to change an answer or remove any marks, use a plastic eraser and be sure to remove all marks and smudges.inteGritY of tHe CompetitionThe AMT reserves the right to re-examine students before deciding whether to grant official status to their score.©amt P ublishing2011amtt limited acn083 950 341Senior DivisionQuestions 1to 10,3marks each1.The expression 3x (x −4)−2(5−3x )equals (A)3x 2−3x −14(B)3x 2−6x −10(C)3x 2−18x +10(D)3x 2−18x −10(E)9x 2−22x2.A coach notices that 2out of 5players in his club are studying at university.If there are 12university students in his club,how many players are there in total?(A)20(B)24(C)30(D)36(E)603.The value of 14÷0.4is (A)3.5(B)35(C)5.6(D)350(E)0.144.In the diagram,ABCD is a square.What is the value of x ?(A)142(B)128(C)48(D)104(E)52................................................................................................................ (52)◦x◦ABCD5.Which of the following is the largest?(A)210(B)210(C)102(D)201(E)2106.If m and n are positive whole numbers and mn =100,then m +n cannot be equalto (A)25(B)29(C)50(D)52(E)1017.P QRS is a square.T is a point on RS such that QT =2RT .The value of x is (A)100(B)110(C)120(D)150(E)160.......................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................P QRS T x ◦8.In my neighbourhood,90%of the properties are houses and 10%are shops.Today,10%of the houses are for sale and 30%of the shops are for sale.What percentage of the properties for sale are houses?(A)9%(B)80%(C)3313%(D)75%(E)25%9.The value of 12+14+182+4+8is(A)16(B)4(C)1(D)14(E)11610.Anne’s morning exercise consists of walking a distance of 1km at a rate of 5km/h,jogging a distance of 3km at 10km/h and fast walking for a distance of 2km at 6km/h.How long does it take her to complete her morning exercise?(A)30min(B)35min(C)40min(D)45min(E)50minQuestions 11to 20,4marks each11.The diagram shows a square of sidelength 12units divided into six triangles of equal area.What is the distance,in units,of T from the side P Q ?(A)4(B)3(C)2(D)1(E)√5......................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................PS QRT12.Each of the ﬁrst six prime numbers is written on a separate card.The cards areshu ﬄed and two cards are selected.The probability that the sum of the numbers selected is prime is(A)15(B)14(C)13(D)12(E)1613.Two tourists are walking 12km apart along a ﬂat track at a constant speed of4km/h.When each tourist reaches the slope of a mountain,she begins to climb with a constant speed of 3km/h.✲✛12km✡✡✣✑✑✸✑✑✰k m What is the kilometres,the two tourists during the climb?(A)16(B)12(C)10(D)9(E)814.Lines parallel to the sides of a rectangle 56cm by 98cm and joining its oppositeedges are drawn so that they cut this rectangle into squares.The smallest number of such lines is(A)3(B)9(C)11(D)20(E)7515.What is the sum of the digits of the positive integer n for which n 2+2011is thesquare of an integer?(A)6(B)7(C)8(D)9(E)1016.Of the sta ﬀin an o ﬃce,15rode a pushbike to work on Monday,12rode on Tuesdayand 9rode on Wednesday.If 22sta ﬀrode a pushbike to work at least once during these three days,what is the maximum number of sta ﬀwho could have ridden a pushbike to work on all three days?(A)4(B)5(C)6(D)7(E)812 kmk m17.How many integer values of n make n 2−6n +8a positive prime number?(A)1(B)2(C)3(D)4(E)an inﬁnite number18.If x 2−9x +5=0,then x 4−18x 3+81x 2+42equals(A)5(B)25(C)42(D)67(E)8119.The centre of a sphere of radius 1is one of the vertices of a cube of side 1.What is the volume of the combined solid?(A)7π6+1(B)7π6+56(C)7π6+43(D)7π8+1(E)π+120.In a best of ﬁve sets tennis match (where the ﬁrst player to win three sets wins thematch),Chris has a probability of 23of winning each set.What is the probabilityof him winning this particular match?(A)23(B)190243(C)89(D)1927(E)6481Questions 21to 25,5marks each21.How many 3-digit numbers can be written as the sum of three (not necessarilydi ﬀerent)2-digit numbers?(A)194(B)198(C)204(D)287(E)29622.A rectangular sheet of paper is folded along a single line so that one corner lieson top of another.In the resulting ﬁgure,60%of the area is two sheets thick and 40%is one sheet thick.What is the ratio of the length of the longer side of the rectangle to the length of the shorter side?(A)3:2(B)5:3(C)√2:1(D)2:1(E)√3:223.An irrational spider lives at one corner of a closed box which is a cube of edge 1metre.The spider is not prepared to travel more than √2metres from its home (measured by the shortest route across the surface of the box).Which of the following is closest to the proportion (measured as a percentage)of the surface of the box that the spider never visits?(A)20%(B)25%(C)30%(D)35%(E)50%24.Functions f ,g and h are deﬁned byf (x )=x +2g (0)=f (1)g (x )=f (g (x −1))for x ≥1h (0)=g (1)h (x )=g (h (x −1))for x ≥1.Find h (4).(A)61(B)117(C)123(D)125(E)31325.A cone has base diameter 1unit and slant height 3units.From a point A halfwayup the side of the cone,a string is passed twice around it to come to a point B on the circumference of the base,directly below A .The string is then pulled until taut.............................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................ABHow far is it from A to B along this taut string?(A)38(√29+√53)(B)3√72(C)3√32(D)94(E)3√1088For questions 26to 30,shade the answer as an integer from 0to 999inthe space provided on the answer sheet.Question 26is 6marks,question 27is 7marks,question 28is 8marks,question 29is 9marks and question 30is 10marks.26.Paul is one year older than his wife and they have two children whose ages are alsoone year apart.Paul notices that on his birthday in 2011,the product of his age and his wife’s age plus the sum of his children’s ages is 2011.What would have been the result if he had done this calculation thirteen years before?27.The diagram shows the net of a cube.On each face there is an integer:1,w ,2011,x ,y and z .................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................... (x)yz2011w1If each of the numbers w ,x ,y and z equals the average of the numbers written on the four faces of the cube adjacent to it,ﬁnd the value of x .28.Two beetles sit at the vertices A and H of a cube ABCDEF GH with edge length40√110units.The beetles start moving simultaneously along AC and HF with the speed of the ﬁrst beetle twice that of the other one.....................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................ADCBEF GH✈s✈s ..................................................................................................................................................................................................................................................................................................................................What will be the shortest distance between the beetles?29.A family of six has six Christmas crackers to pull.Each person will pull twocrackers,each with a di ﬀerent person.In how many di ﬀerent ways can this be done?30.A40×40white square is divided into1×1squares by lines parallel to its sides.Some of these1×1squares are coloured red so that each of the1×1squares, regardless of whether it is coloured red or not,shares a side with at most one red square(not counting itself).What is the largest possible number of red squares?Senior 2011 Answers Question Answer 1B2C3B4A5B6C7C8D9E10E11B12A13D14B15A16D17B18D19A20E21B22C23C24D25B269972780528440297030420。

2013A试卷AMC-A：澳大利亚数学3-4年级中文历年真题

THURSDAY 1 AUGUST 2013MIDDLE PRIMARY DIVISIONAUSTRALIAN S CHOOL YEARS 3 & 4TIME ALLOWED: 60 MINUTESINSTRUCTIONS AND INFORMATION GENERAL 1. Do not open the booklet until told to do so by your teacher. 2. You may use any teaching aids normally available in your classroom, such as MAB blocks, counters, currency, calculators, play money etc. You are allowed to work on scrap paper and teachers may explain the meaning of words in the paper. 3. Diagrams are NOT drawn to scale. They are intended only as aids. 4. There are 25 multiple-choice questions, each with 5 possible answers given and 5 questions that require a whole number answer between 0 and 999. The questions generally get harder as you work through the paper. There is no penalty for an incorrect response. 5. This is a competition not a test; do not expect to answer all questions. You are only competing against your own year in your own State or Region so different years doing the same paper are not compared. 6. Read the instructions on the answer sheet carefully. Ensure your name, school name and school year are entered. It is your responsibility to correctly code your answer sheet. 7. When your teacher gives the signal, begin working on the problems. THE ANSWER SHEET 1. Use only lead pencil.2. Record your answers on the reverse of the answer sheet (not on the question paper) by FULLY colouring the circle matching your answer.3. Your answer sheet will be scanned. The optical scanner will attempt to read all markings even if they are in the wrong places, so please be careful not to doodle or write anything extra on the answer sheet. If you want to change an answer or remove any marks, use a plastic eraser and be sure to remove all marks and smudges.INTEGRITY OF THE COMPETITIONThe AMT reserves the right to re-examine students before deciding whether to grant official status to their score.©AMT P ublishing 2013 AMTT liMiTed Acn 083 950 341A ustrAliAn M AtheMAtics c oMpetitionsponsored by the c oMMonweAlth b AnkAn AcTiviTy of The AusTrAliAn MATheMATics TrusT姓名：年级：监考老师：意事项一般规定1.未获监考老师许可之前不可翻开此测验题本。

AMC8(美国数学竞赛)历年真题、答案及中英文解析

AMC8（美国数学竞赛）历年真题、答案及中英文解析艾蕾特教育的AMC8 美国数学竞赛考试历年真题、答案及中英文解析：AMC8-2020年：真题 --- 答案---解析（英文解析+中文解析）AMC8 - 2019年：真题----答案----解析（英文解析+中文解析）AMC8 - 2018年：真题----答案----解析（英文解析+中文解析）AMC8 - 2017年：真题----答案----解析（英文解析+中文解析）AMC8 - 2016年：真题----答案----解析（英文解析+中文解析）AMC8 - 2015年：真题----答案----解析（英文解析+中文解析）AMC8 - 2014年：真题----答案----解析（英文解析+中文解析）AMC8 - 2013年：真题----答案----解析（英文解析+中文解析）AMC8 - 2012年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 2010年：真题----答案----解析（英文解析+中文解析）AMC8 - 2009年：真题----答案----解析（英文解析+中文解析）AMC8 - 2008年：真题----答案----解析（英文解析+中文解析）AMC8 - 2007年：真题----答案----解析（英文解析+中文解析）AMC8 - 2006年：真题----答案----解析（英文解析+中文解析）AMC8 - 2005年：真题----答案----解析（英文解析+中文解析）AMC8 - 2004年：真题----答案----解析（英文解析+中文解析）AMC8 - 2003年：真题----答案----解析（英文解析+中文解析）AMC8 - 2002年：真题----答案----解析（英文解析+中文解析）AMC8 - 2001年：真题----答案----解析（英文解析+中文解析）AMC8 - 2000年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 1998年：真题----答案----解析（英文解析+中文解析）AMC8 - 1997年：真题----答案----解析（英文解析+中文解析）AMC8 - 1996年：真题----答案----解析（英文解析+中文解析）AMC8 - 1995年：真题----答案----解析（英文解析+中文解析）AMC8 - 1994年：真题----答案----解析（英文解析+中文解析）AMC8 - 1993年：真题----答案----解析（英文解析+中文解析）AMC8 - 1992年：真题----答案----解析（英文解析+中文解析）AMC8 - 1991年：真题----答案----解析（英文解析+中文解析）AMC8 - 1990年：真题----答案----解析（英文解析+中文解析）AMC8 - 1989年：真题----答案----解析（英文解析+中文解析）AMC8 - 1988年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 1986年：真题----答案----解析（英文解析+中文解析）AMC8 - 1985年：真题----答案----解析（英文解析+中文解析）◆AMC介绍◆AMC（American Mathematics Competitions) 由美国数学协会（MAA）组织的数学竞赛，分为 AMC8 、 AMC10、 AMC12 。

2013年AMC_12真题(B)

8. Line 1 has equation 3x − 2y = 1 and goes through A = (−1, −2). Line 2 has equation y = 1 and meets line 1 at point B. Line 3 has positive slope, goes through point A, and meets 2 at point C. The area of △ABC is 3. What is the slope of 3 ? (A)
USA AMC 12/AHSME 2013 B
1. On a particular January day, the high temperature in Lincoln, Nebraska, was 16 degrees higher than the low temperature, and the average of the high and low temperatures was 3 . In degrees, what was the low temperature in Lincoln that day? (A) − 13 (B) − 8 (C) − 5 (D) 3 (E) 11
16. Let ABCDE be an equiangular convex pentagon of perimeter 1. The pairwise intersections of the lines that extend the side of the pentagon determine a five-pointed star polygon. Let s be the perimeter of the star. What is the difference between the maximum and minimum possible perimeter of s?

2013年AMC 10B竞赛真题及答案(英文版)

2013年美国数学竞赛AMC10B真题What is ?Problem 2Mr. Green measures his rectangular garden by walking two of the sides and finding that it is steps by steps. Each of Mr. Green's steps is feet long. Mr. Green expects a half a pound of potatoes persquare foot from his garden. How many pounds of potatoes does Mr. Green expect from his garden?Problem 3On a particular January day, the high temperature in Lincoln, Nebraska, was degrees higher than the low temperature, and the average of the high and the low temperatures was . In degrees, whatwas the low temperature in Lincoln that day?Problem 4When counting from to , is the number counted. When counting backwardsfrom to , is the number counted. What is ?Problem 5Positive integers and are each less than . What is the smallest possible value for ?The average age of 33 fifth-graders is 11. The average age of 55 of their parents is 33. What is the average age of all of these parents and fifth-graders?Problem 7Six points are equally spaced around a circle of radius 1. Three of these points are the vertices of atriangle that is neither equilateral nor isosceles. What is the area of this triangle?Problem 8Ray's car averages 40 miles per gallon of gasoline, and Tom's car averages 10 miles per gallon of gasoline. Ray and Tom each drive the same number of miles. What is the cars' combined rate of milesper gallon of gasoline?Problem 9Three positive integers are each greater than , have a product of , and are pairwise relativelyprime. What is their sum?Problem 10A basketball team's players were successful on 50% of their two-point shots and 40% of their three-point shots, which resulted in 54 points. They attempted 50% more two-point shots than three-point shots. How many three-point shots did they attempt?Real numbers and satisfy the equation . What is ?Problem 12Let be the set of sides and diagonals of a regular pentagon. A pair of elements of are selected atrandom without replacement. What is the probability that the two chosen segments have the samelength?Problem 13Jo and Blair take turns counting from to one more than the last number said by the other person. Jostarts by saying "", so Blair follows by saying "" . Jo then says "" , and so on. What is the53rd number said?Problem 14Define . Which of the following describes the set of points forwhich ?Problem 15A wire is cut into two pieces, one of length and the other of length . The piece of length is bent to form an equilateral triangle, and the piece of length is bent to form a regular hexagon. The triangle and the hexagon have equal area. What is ?In triangle , medians and intersect at , , , and . What isthe area of ?Problem 17Alex has red tokens and blue tokens. There is a booth where Alex can give two red tokens andreceive in return a silver token and a blue token, and another booth where Alex can give three blue tokens and receive in return a silver token and a red token. Alex continues to exchange tokens until nomore exchanges are possible. How many silver tokens will Alex have at the end?Problem 18The number has the property that its units digit is the sum of its other digits, thatis . How many integers less than but greater than share this property?Problem 19The real numbers form an arithmetic sequence with . Thequadratic has exactly one root. What is this root?Problem 20The number is expressed in the formwhere and are positive integers and is as small aspossible. What is ?Two non-decreasing sequences of nonnegative integers have different first terms. Each sequence has the property that each term beginning with the third is the sum of the previous two terms, and the seventh term of each sequence is . What is the smallest possible value of N?Problem 22The regular octagon has its center at . Each of the vertices and the center are to beassociated with one of the digits through , with each digit used once, in such a way that the sums ofthe numbers on the lines , , , and are all equal. In how many ways can this bedone?In triangle , , , and . Distinct points , , and lie onsegments , , and , respectively, such that , , and . Thelength of segment can be written as , where and are relatively prime positive integers.What is ?Problem 24A positive integer is nice if there is a positive integer with exactly four positive divisors (including and ) such that the sum of the four divisors is equal to . How many numbers in theset are nice?Bernardo chooses a three-digit positive integer and writes both its base-5 and base-6 representations on a blackboard. Later LeRoy sees the two numbers Bernardo has written. Treating the two numbers as base-10 integers, he adds them to obtain an integer . For example, if ,Bernardo writes the numbers and , and LeRoy obtains the sum . For how many choices of are the two rightmost digits of , in order, the same as those of ?Answer Key1. C2. A3. C4. D5. B6. C7. B8. B9. D10.C11.B12.B13.E14.E15.B16.B17.E18.D19.D20.B21.C22.C23.B24.A25.E。

美国中学数学竞赛(AMC12-12级)(附答案)

2004 AMC12 试题1. 爱丽每小时的工资为美金20元，其中的1.45%要缴地方税，试问爱丽每小时的工资中要付地方税美金多少分？(美金1元二美金100分)(A) 0.0029 (B) 0.029 (C) 0.29 (D) 2.9 (E) 292. 于AMC2的测验中，每答对一题可得6分，答错可得0分，未作答可得2.5分.假设凯琳在25题中有8题未作答，她至少要答对几题，总分才会不低于100 分？(A) 11(B) 13(C) 14(D) 16(E) 173.滿足x 2y100的正整数序对(x, y)有多少組？(A) 33(B) 49(C) 50(D) 99(E) 1004.白婆婆有6个女儿、没有儿子，有些女儿也恰有6个女儿，其他的女儿没有孩子. 白婆婆有女儿及外孙女共30位，没有外曾孙女. 试问白婆婆的女儿及外孙女中有多少位没有女儿？(A) 22 (B) 23 (C) 24 (D) 25 (E) 265.直线y mx(A) mb 1 (B) 1 mb 0 (C) mb 0 (D) 0 mb 1 (E) mb 16.设u 2 20042005, V 20042005, W 2003 20042004, X 2 20042004Y 20042004, Z 20042003.試问以下何者值最大？(A) U V (B) V W (C) W X (D) X Y (E) Y Z7. 一种代币的游戏，其规那么如下：每回持有最多代币者须分给其他每一位参与游戏者一枚代币，并放一枚代币於回收桶中；当有一位游戏参与者没有代币时，那么游戏结束.假设A、B C三人玩此游戏，在游戏开始时分别持有15、14及13枚代币.试问游戏从开始到结束，共进行了多少回？(A) 36 (B) 37 (C) 38 (D) 39 (E) 409. 有一个将花生酱装在圆桶状瓶子内出售的公司 .市场研究建议瓶子较粗时可增加销售量.假设瓶子的直径增加25%,而体积仍维持不变，那么瓶子的高度应减少百分之多少？(A) 10(B) 25(C) 36(D) 50(E) 6010. 有49个连续整数，它的和为75,則它们排在最中间的数为何？(A) 7(B) 72(C) 73(D) 74(E) 7511. 某国的硬币中有1分、5分、10分及25分四种，在保拉的皮包內硬币的平均值为20分.假设再增加一枚25分的硬币，平均值則增为 21分.試问她的皮包內有多少枚10分的硬币？(A) 0(B) 1(C) 2(D) 3(E) 412. 设A (0, 9) , B (0,12).点A 、B 在直线y x 上, 且AA 与BB 交于点 C (2,8).試问AB的长度是多少？(A) 2(B) 2 2(C) 3(D) 22(E) 3“13. 以S 表示坐标平面上所有的点(a,b)所形成的集合，其中a,b 等于1, 0,或1.試问有多少条相异的直线至少通过集合 S 中的两个点？(A) 8(B) 20(C) 24(D) 27(E) 3614. 三个实数的数列形成一个等差数列，首项是9.假设将第二项加2、第三项加20可使得这三个数变为等比数列，那么这个等比数列中第三项最小可能是多少？(A) 1(B) 4(C) 36(D) 49(E) 8115.小美与小雯在一个圆形的跑道上向相反的方向跑，开始两人分别从8.如下图EAB 及 ABC 为直角，厢 4 , BC 6 , AE 8, AC 与BE 交于D 点.試问差为多少？(A) 2(B) 4(C) 5 (D) 8 (E) 9ADE 与BDC 面积之圆形跑道直径的两端起跑.小美跑了 100公尺时她们第一次相遇；在第一次相遇后小雯跑了 150公尺时她们第二次相遇.假设她们跑的速度都分别维持固定不变，试问此圆形跑道的长度是多少公尺？(A) 250(B) 300(C) 350(D) 400(E) 50016.使函数 log 2004{log 2003{log 2002{log 2001 X}}}有定义的集合为之值是多少？(A) 0(B) 20012002(C) 20022003(D) 20032004{xx c}.試问 c(E) 20012°°丹317.函数f 满足以下性质： (i) f(1) 1,且(ii)对任意的正整数n ,f(2n) n f(n).试问f(2100)之值为何？(A) 1(B) 299(C) 2100(D) 24950 18.如下图，ABCD 是边长2的正方形.在正方形的内部作一个以AB 为直径的半圆，且自C 点引此半圆的切线交AD 边于E 点•试问CE 的长度是多少？(E) 5 ■.5(E) 29999(B) 5 (C) 6 (D)?19.如下图，A B C 三圆彼此外切且均内切于圆等，圆A 的半径1且通过圆D 的圆心.试问圆B 的半径是多少?D.B 、C 两圆相(A)3(B)于(C) 土 (D) |20.从0与1之间的数，随机独立取出两实数a 与b,并将a, b 之和记作c.分别以A, B, C 表示最接近a, b, c 的整数.试问的机率为何？(A) 1(B) !(C) 1(D) 2(E) 443 23421.假设n 02nCOS5,那么Cos2之值是多少？(A) 15 (B) I (C) ±55(D) ?(E)上5522.三个半径为1的球彼此外切且放置在一水平面上，一个半径为2 的大球放在它们的上面 .试问大球的最高点至平面的距离是多少？C 2004 0,且 P (x)=0 有 2004个复数根 z a k b k i , 20042004b k 为实数，a ib i 0,且a kb k .k 1k 1试问以下哪一项可能不是 0 ?2004(A) C 0(B)C2003(C) b z b s L b s 004 (D)a kk 124. 设A 、B 为平面上的两点，其中AB 1.令S 为平面上所有半径是1且能盖住线段AB 之圆的并集，則S 的面积是多少？(A) 2.3(B) -(C) 33(D)卫 3(E) 4 2 33 2325. 对每一个整数n 4,令a n 表示n 进位的循环小数0.133n .把乘积a 4a 5L a 99写成—的形式，其中m P 为正整数，且p 尽可能小.试问mp!之值是多少？(A) 98(B) 101(C) 132(D) 798(E) 962Q屈o J123 52(A) 3(B) 3 -(C) 3 -(D)2349(E) 3 2、223.多项式P(x) GX C 0 ,的系数都是实数, 1 k 2004,其中 a k ,2004(E)C kk 12004C 2004X 2003C 2003XL答案:1 ( E )2 ( C )3 ( B )4 ( E )5 ( B )6 ( A )7 ( B )8 ( B )9 ( C )10 ( C ) 11 ( A )12 ( B )13 ( B )14 ( A )15 ( C ) 16 ( B )17 ( D )18 ( D )19 ( D )20( E ) 21 ( D )22 ( B )23 ( E )24 ( C )25 ( E )。

澳大利亚数学竞赛小学中年级(3--4年级)(2013年)

当小珍向镜子里看去，请问她所看到这顶帽子上的字样是什么？（）7.直线棋盘上的格子从左至右的编号为 1, 2, 3, …。

小莎的棋子向右移动 6 格，向左移动 4 格，然后向右移动 3 格。

他知道只要沿着如下图所示螺旋状的路径便可走出这个迷宫。

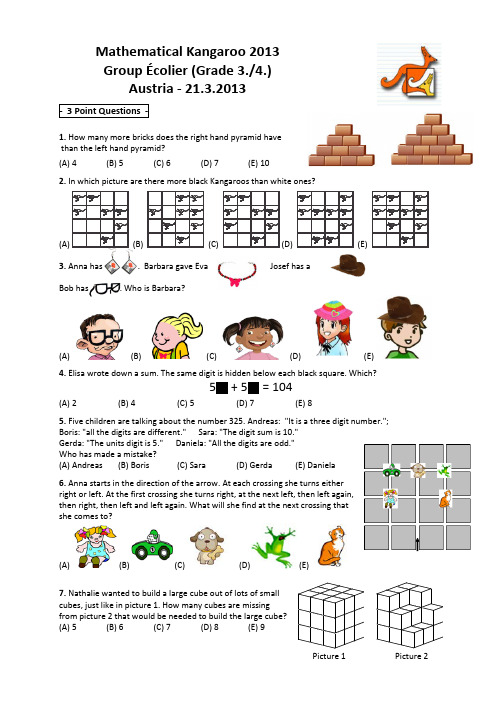
澳大利亚kangaroo袋鼠数学竞赛试题及答案grade3-11 2013年

- 3 Point Questions -1. How many more bricks does the right hand pyramid havethan the left hand pyramid?(A) 4 (B) 5 (C) 6 (D) 7 (E) 102. In which picture are there more black Kangaroos than white ones?(B) (E)3. Anna has . Barbara gave Eva . Josef has a .Bob has . Who is Barbara?(A) (B) (C) (D) (E)4. Elisa wrote down a sum. The same digit is hidden below each black square. Which?5 + 5 = 104(A) 2 (B) 4 (C) 5 (D) 7 (E) 85. Five children are talking about the number 325. Andreas: "It is a three digit number."; Boris: "all the digits are different." Sara: "The digit sum is 10."Gerda: "The units digit is 5." Daniela: "All the digits are odd."Who has made a mistake?(A) Andreas (B) Boris (C) Sara (D) Gerda (E) Daniela6. Anna starts in the direction of the arrow. At each crossing she turns eitherright or left. At the first crossing she turns right, at the next left, then left again,then right, then left and left again. What will she find at the next crossing thatshe comes to?(A) (B)(C)(D) (E)7. Nathalie wanted to build a large cube out of lots of smallcubes, just like in picture 1. How many cubes are missingfrom picture 2 that would be needed to build the large cube?(A) 5 (B) 6 (C) 7 (D) 8 (E) 9Mathematical Kangaroo 2013Group Écolier (Grade 3./4.)Austria - 21.3.2013Picture 1 Picture 28. The rectangular mirror has broken. Which piece is missing?(A) (B) (C) (D) (E)- 4 Point Questions -9. How many triangles can be seen in the picture on the right? (Be careful!A triangle can be also be made by joining several smaller trianglestogether.)(A) 9 (B) 10 (C) 11 (D) 13 (E) 1210. Veras Mum has made sandwiches, each using two slices of bread.There are 24 slices in a pack. How many sandwiches can she make withtwo whole and one half packets of bread?(A) 6 (B) 12 (C) 24 (D) 30 (E) 4811. Daniel had 36 sweets. He shared them equally between his siblings. How many siblings can he definitely not have?(A) 2 (B) 3 (C) 4 (D) 5 (E) 612. Pinocchios nose is 9cm long. If he lies his nose grows by 6cm. If he tells the truth it shortens by2cm. He tells three untruths and twice the truth. How long is Pinocchios nose now?(A) 14 cm (B) 15 cm (C) 19 cm (D) 23 cm (E) 31 cm13. Which of the following pieces can be joined to the one pictured so that a rectangle isformed?(A) (B) (C) (D) (E)14. In a shop you can buy oranges in bags of 4 or bags of 10. Pedro wants to buy exactly 48 oranges. What is the smallest number of bags he must buy?(A) 8 (B) 7 (C) 6 (D) 5 (E) 415. At the London 2012 Olympic games the USA won the most medals:46 Gold-, 29 Silver- and 29 Bronze medals. China was second with 38 Gold-, 27 Silver- and23 Bronze medals. How many more medals did the USA win than China?(A) 6 (B) 14 (C) 16 (D) 24 (E) 2616. 30 children took part in a sports competition, in the sports football and handball. 15 took part in football and 20 took part in handball. How many students took part in both sports?(A) 25 (B) 15 (C) 30 (D) 10 (E) 5- 5 Point Questions -17. The number 35 has the property that it can be divided by its unit digit, because 35 divided by 5 is exactly 7. The number 38 does not have this property. How many numbers bigger than 21, but smaller than 30 have this property?(A) 2 (B) 3 (C) 4 (D) 5 (E) 618. In February 2013 Schnurrli the tomcat slept for exactly three weeks. For how many hours in this month was he awake?(A) 168 (B) 192 (C) 216 (D) 240 (E) 50419. Andi, Betti, Clara and Dani were born in the same year. Their birthdays are on the 20th February, 12th April, 12th May and 25th May, but not necessarily in that order. Betti and Andi were born in the same month. Andi and Clara were born on the same day in different months. Who is the oldest? (A) Andi (B) Betti (C) Clara (D) Dani(E) There is not enough information to answer the question.20. If I join the midpoints of the sides of the large triangle in the picture, a smalltriangle is formed. If I join the midpoints of the sides of this small triangle, a tinytriangle is formed. How many of these tiny triangles can fit into the largesttriangle at the same time?(A) 5 (B) 8 (C) 10 (D) 16 (E) 3221. Chrissi wants to sell 10 glass marbles which each have a different weight. Their weights are:1 dag,2 dag,3 dag,4 dag,5 dag,6 dag,7 dag,8 dag,9 dag and 10 dag. They should be packed into bags two at a time, so that each bag has the same weight. Which two marbles will be put into the same bag?(A) 3 and 6 (B) 3 and 7 (C) 3 and 8 (D) 3 and 9 (E) 3 and 1022. Baris has a few dominoes as shown in the picture. He wants to lay them in a line according to the rules of dominoes, that is that two dominoes can only be laid together if the neighbouring squares have the same number of dots in them. What is the biggest number of these dominoes that he can lay in a single line?(A) 3 (B) 4 (C) 5 (D) 6 (E) 723. Peter has bought a rug that is 36 dm wide and60 dm long. On the rug you can see squares thatcontain either a sun or a moon, as shown in thepicture. As you can see there are exactly ninesquares along the width of the rug. The totallength of the rug cannot be seen. How manymoons would you see, if you could see the entirerug?(A) 68 (B) 67 (C) 65 (D) 63 (E) 6024. Beatrice has a few grey tiles that all look exactly like the one pictured. Atleast how many of these tiles does she need in order to make a completesquare? (A) 3 (B) 4 (C) 6 (D) 8 (E) 16- 3 Point Questions -1. Which answer completes the addition tree? (A) 2 (B) 3 (C) 4 (D) 5 (E) 62. Nathalie wanted to build a large cube out of lots of small cubes. How many cubes are missing from the picture on the right that would be needed to build the large cube on the left? (A) 5 (B) 6 (C) 7 (D) 8 (E) 9(A) 300 m (B) 400 m (C) 800 m (D) 1 km (E) 700 m 4. Nick can turn right but not left on his bicycle. What is the least number of right turns he must make in order to get from A to B? (A) 3 (B) 4 (C) 6 (D) 8 (E) 10 5. Anna, Bob and Chris are altogether 31 years old. How old will all three be altogether in three years time? (A) 32 (B) 34 (C) 35 (D) 37 (E) 40 6. In the following sum the same digit is used in each square: □□ × □ = 176 Which digit must be used so that the sum is correct? (A) 6 (B) 4 (C) 7 (D) 9 (E) 8 7. Michael must take a tablet every 15 minutes. He takes the first at 11:05. When does he take the fourth? (A) 11:40 (B) 11:50 (C) 11:55 (D) 12:00 (E) 12:05 8. Anne has a few grey tiles like the one in the picture. What is the maximum number of these tiles that she can place on the 5 ×overlaps? (A) 2 (B) 3 (C) 4 (D) 5 (E) 6- 4 Point Questions -9. The number 36 has the following property: 36 can be divided by its units digit without a remainder (36 is divisible by 6). With the number 38 this doesn't work. How many numbers between 20 and 30 have the same property as 36? (A) 2 (B) 3 (C) 4 (D) 5 (E) 6Mathematical Kangaroo 2013 Group Benjamin (Grade 5/6) Austria - 21.3.201310. Maria drew the following figures on square sheets of paper.How many of these figures have the same perimeter as the square sheet of paper itself?(A) 2 (B) 3 (C) 4 (D) 5 (E) 611. Patricia drives one afternoon at a constant speed to her friend. She looks at her watch as she leaves and when she arrives.In which position will the minute hand be when she has completed one third of her journey?12. Johann stacks 1×1 cubes on the squares of a 4×4 grid. The diagram on the rightshows how many cubes were piled on top of each other on each square of the grid.What will Johann see if he looks from behind (hinten) at the tower?13. 36 children voted for five students from their class. Each child was only allowed to vote once. The winner received 12 votes, and the student placed last just 4 votes. If each student received a different number of votes, how many votes did the second placed student receive?(A) 8 (B) 8 or 9 (C) 9 (D) 9 or 10 (E) 1014. A 1×1×1 cube is cut out of each corner of a 3×3×3 cube. The picture shows theresult after the first cube is cut out. How many faces will the final shape have?(A) 16 (B) 20 (C) 24 (D) 30 (E) 3615. How many different subtraction sums between two digit numbers are there,which have the answer 50?(A) 40 (B) 30 (C) 50 (D) 60 (E) 1016. In the last game of the hockey match there were lots of goals. In the first half 6 goals were scored and the visiting team were leading. After the home team scored another three goals in the second half, they won the match. How many goals did the hometeam score in total?(A) 3 (B) 4 (C) 5 (D) 6 (E) 7- 5 Point Questions -17. Which of the figures below will cover the most dots when laid on the square shownon the right.(A)(B)(C)(D)(E)18. Matthias is catching fish. If he had caught three times as many fish as he has actually caught, he would have 12 more fish. How many fish has he caught?(A) 7 (B) 6 (C) 5 (D) 4 (E) 319. Numbers are written in the 4x4 grid: any two numbers in neighbouringsquares should have a difference of 1, that is squares that share an edge. Thenumber 3 is already given. The number 9 will be used somewhere in the grid.How many different numbers will have been used once the grid is filled incompletely?(A) 4 (B) 5 (C) 6 (D) 7 (E) 820. Two buttons with smiling faces and two buttons with sad faces are in a row as shown in the picture. When you press a button the face changes, and so do the faces of the neighbouring buttons. What is the minimum number of button presses needed so that only smiling faces can be seen?(A) 2 (B) 3 (C) 4 (D) 5 (E) 621. If you start with three numbers, the 'addition machine' produces three new ones by adding together each pair of two. For instance from the numbers {3, 4, 6} the addition machine makes {10, 9, 7}. If you use the addition machine again these numbers become {16, 17, 19}. We feed the three numbers {20, 1, 3} into the addition machine and let the machine calculate 2013 times. What is the biggest possible difference between two of the three resulting numbers?(A) 1 (B) 2 (C) 17 (D) 19 (E) 201322. From an old model train set there are only identical pieces oftrack to use. Matthias puts 8 such pieces in a circle (picture on theleft). Martin begins his track with 2 pieces as shown in the picture onthe right. He also wants to build a closed track and use the smallestnumber of pieces possible. How many pieces will his track use?(A) 11 (B) 12 (C) 14 (D) 15 (E) 1623. 2013 people live on an island. Some of these people aretruthtellers and the others are liars. The truthtellers always tell the truth whereas the liars always lie. Each day one of the people says ' when I have left the island the number of truthtellers will be the same as the number of liars.' Then he leaves the island. After 2013 days there is no longer anybody living on the island. How many liars were living there to begin with?(A) 0 (B) 1006 (C) 1007 (D) 2013 (E) It is not possible to answer.24. 40 boys and 28 girls hold hands in a big circle. Exactly 18 boys give their right hand to a girl. How many boys give their left hand to a girl?(A) 18 (B) 9 (C) 28 (D) 14 (E) 20- 3 Point Questions - 1. Triangle ABC is equilateral and has area 9. The dividing lines are parallel to the sides, and divide the sides into three equal lengths. What is the area of the grey shaded part of the triangle?(A) 1 (B) 4 (C) 5 (D) 6 (E) 72. We know that . How big is the sum ? (A) 5 (B) 9 (C) 11 (D) 55 (E) 993. In sea water the ratio of Salt to fresh water is 7 : 193. How many kilograms of salt will be found in 1000kg of sea water? (A) 35 (B) 186 (C) 193 (D) 200 (E) 3504. Melanie has a square piece of paper with a 4x4 grid drawn on it. She cuts along the gridlines and cuts several shapes out which all look either the same as the one pictured, or the same as its mirror image. How many squares are left over if she cuts out as many shapes as possible?(A) 0 (B) 2 (C) 4 (D) 6 (E) 85. Matthias catches fish. If he had caught three times as many fish as he has actually caught, he would have 12 fish more. How many fish has he caught? (A) 7 (B) 6 (C) 5 (D) 4 (E) 36. A sack contains marbles in five different colours: 2 red, 3 blue, 10 white, 4 green, and 3 black marbles. You take marbles out of the bag without looking and without putting them back. What is the smallest number of marbles you must remove from the sack to be sure of having two of the same colour?(A) 2 (B) 12 (C) 10 (D) 5 (E) 67. Alex lights a candle every 10 minutes. Each candle burns for 40 minutes before going out. How many candles are burning 55 minutes after he lit the first candle?(A) 2 (B) 3 (C) 4 (D) 5 (E) 68. Marie calculates the average number of children in families in her village. Five families live in the village. Which answer could she not get?(A) 1⋅0 (B) 1⋅2 (C) 1⋅3 (D) 1⋅4 (E) 2⋅09. Tom and Laura stand directly opposite each other around a circular well. At the same time, they begin to run clockwise around the well. Tom's speed is of Laura's speed. How many time full laps of the well will laura run before Tom catches her for the first time?(A) 4(B) 8 (C) 9 (D) 2 (E) 7210. For the positive whole numbers x, y, z the following is true: x × y = 14, y × z = 10 und z× x = 35. What is the value of x + y + z? (A) 10 (B) 12 (C) 14 (D) 16 (E) 18111110111=33336666101303+=98Mathematical Kangaroo 2013 Group Kadett (Grade 7./8.) Austria - 21.3.2013 A BC11. Anne plays 'sink the ship' with a friend, on a 5×5 grid. She has already drawn in a 1×1 shipand a 2×2 ship (as shown in the picture). She must also draw a (rectangular) 3×1 ship. Ships may be neither directly nor diagonally adjacent to each other. How many possible positionsare there for the 3×1 ship? (A) 4 (B) 5 (C) 6 (D) 7 (E) 8 12. In the diagram pictured, α = 55°, β = 40° and γ = 35°. How big is δ? (A) 100° (B) 105° (C) 120° (D) 125° (E) 130°13. The perimeter of a trapezium is 5, and the side lengths are whole numbers. How many degrees do the smallest angles measure? (A) 30° and 30° (B) 60° and 60° (C) 45° and 45° (D) 30° and 60° (E) 45° and 90°14. The five shapes pictured were cut out of paper. Four of them can be folded to form a cube. For which shape is this not possible.(A) Shape 1 (B) Shape 2 (C) Shape 3 (D) Shape 4 (E) Shape 5 15. Willi wrote down a few consecutive whole numbers. A certain percentage of these numbers are odd. Which of the following values cannot be the calculated percentage?(A) 40% (B) 45% (C) 48% (D) 50% (E) 60%16. Aron, Ben and Carl always lie. Each of them picks a red or a green stone.Aron says: "My stone has the same colour as Bens stone." Ben says: "My stone has the same colour as Carls stone." Carl says: "Exactly two of us have red stones." Which of the following is correct? (A) Arons stone is green. (B) Bens stone is green. (C) Carls stone is red. (D) Arons stone and Carls Stone have different colours. (E) None of the possibilities are correct.17. All four digit positive numbers, which have the same digits as 2013 were written on a blackboard in ascending order. Determine the largest possible difference between two consecutive numbers on the blackboard. (A) 702 (B) 703 (C) 693 (D) 793 (E) 19818. In the 8×6 grid pictured, there are 24 squares that have not been cut by eitherof the two diagonals. Now we draw the two diagonals on a 10×6 grid. How many squares in this grid will not be cut by either of the two diagonals?(A) 28 (B) 29 (C) 30 (D) 31 (E) 3219. Andi, Berti, Christa, Doris and Edi were born on the following days; 20.02.2000, 12.03.2000, 20.03.2000, 12.04.2000 and 23.04.2000 respectively. Andi and Edi have their birthday in the same month. Berti and Christa also have their birthday in the same month. Andi and Christa were born on the same day in different months. Doris and Edi were also born on the same day in different months. Which of these children is the youngest? (A) Andi (B) Berti (C) Christa(D) Doris (E) Edi20. Johann stacked 1×1 cubes on the squares of a 4×4 grid. The diagram on the right shows the number of cubes that were stacked on top of each other above each square. What will Johann see if he looks from the back (hinten) at the tower?21. Ralf wants to say a number to Karl, such that the product of its digits is exactly 24. What is the digit sum of the smallest number that Ralf can say?(A) 6 (B) 8 (C) 9 (D) 10 (E) 1122. The sides of the rectangle ABCD are parallel to the co-ordinate axes. Therectangle is positioned below the x-axis and to the right of the y-axis, asshown in the picture. The co-ordinates of the points A, B, C, D are wholenumbers. For each of the points we calculate the value of (y co-ordinate) ÷ (xco-ordinate). Which of the points will give the smallest value?(A) A (B) B (C) C (D) D(E) It depends on the rectangle.23. On a sheet of paper a grid is drawn such that each of the squares has sides 2cm long. How big is the area of the grey shaded quadrilateral ABCD?(A) 96 cm2(B) 84 cm2(C) 76 cm2(D) 88 cm2(E) 104 cm224. Robert chose a five digit positive number. He removed one of the digits so that afour digit number remained. The sum of this four digit and the original five digitnumber is 52713. What is the digit sum of the original five digit number?(A) 26 (B) 20 (C)23 (D) 19 (E) 1725. A gardener wants to plant a row of 20 trees (linden and oak) in a park. There must never be exactly three trees between any two oak trees. What is the maximum number of the 20 trees which could be oak?(A) 8 (B) 10 (C) 12 (D) 14 (E) 1626. In the finishing order of a cross country race, there are twice as many runners behind Alex as there are before Daniel, and 1⋅5 as many behind Daniel as before Alex. Alex finished in 21st place. How many runners finished the race?(A) 31 (B)41 (C) 51 (D) 61 (E) 8127. A sequence of numbers begins with 1, -1, -1, 1, -1. Each new number is found by taking the product of the two preceding numbers. For instance the sixth number is the product of the fourth and fifth numbers. What is the sum of the first 2013 numbers?(A) −1006 (B) −671 (C) 0 (D) 671 (E) 100728. Dad made 6 pancakes, one after the other, and numbered them 1 to 6 in the order that they were made. Sometimes while he did this his children ran into the kitchen and ate the hottest pancakes. In which of the following orders could the pancakes not have been eaten.(A) 123456 (B) 125436 (C) 325461 (D) 456231 (E) 65432129. Each of the 4 vertices and 6 edges of a tetrahedron is labelled with one ofthe numbers 1, 2, 3, 4, 5, 6, 7, 8, 9 and 11. (The number 10 is left out). Eachnumber is only used once. The number on each edge is the sum of the numberson the two vertices which are connected by that edge. The edge AB has thenumber 9. With which number is the edge CD labelled?(A) 4 (B) 5 (C) 6 (D) 8 (E) 1130. Four cars drive into a roundabout at the same point in time, each onecoming from a different direction (see diagram). No car drives all the wayaround the roundabout, and no two cars leave at the same exit. In how many different ways can the cars exit the roundabout?(A) 9 (B) 12 (C) 15 (D) 24 (E) 81- 3 Point Questions - 1) Which of the numbers is not a factor of 200013 – 2013?(A) 2 (B) 3 (C) 5 (D) 7(E) 112) Maria has six equally big square pieces of plain paper. On each piece of paper she draws one of the figures shown below. How many of these figures have the same perimeter as the plain piece of paper itself?(A) 2(B) 3 (C) 4 (D) 5 (E) 63) Mrs. Maisl buys four pieces of corn-on-the-cob for each of the fourmembers of her family and get the discount offered. How much does sheend up paying?(A) 0.80 € (B) 1.20 € (C) 2.80 € (D) 3.20 € (E) 80 €4) The product of three numbers out of the numbers 2, 4, 16, 25, 50, 125 is 1000. How big is the sum of those three numbers?(A) 70 (B) 77 (C) 131 (D) 143 (E) 1775) On a square grid made up of unit squares, six points are marked as shown on the right. Three of which form a triangle with the least area. How big is this smallest area?(A) 1/2 (B) 1/3 (C) 1/4 (D) 1 (E) 26) If you add 415 and 810, you obtain a number that is a power of two. Determine thatnumber!(A) 210 (B) 215 (C) 220 (D) 230 (E) 2317) A cube is coloured on the outside as if it was made up of four white and four black cubes where no cubes of the same colour are next to each other (see picture).Which of the following figures represents a possible net of the coloured cube?(A)(B)(C)(D)(E)8) The number n is the biggest natural number for which 4n is three-digits long and m is the smallest natural number for which 4m is three-digits long. Which value does 4n – 4m have?(A) 900 (B) 899 (C) 896 (D) 225 (E) 2249) In a drawing we can see a three quarter circle with centre M and an indicatedorientation arrow. This three-quarter circle is first turned 90° anti-clockwise about Mand then reflected in the x – axis. Which is the resulting picture?(A)(B)(C)(D)(E) Special corn-on-the-cob offer! 1 Cob 20 Cent Every 6th cob free!Mathematical Kangaroo 2013 Group Junior (Grade 9./10.) Austria - 21.3.2013A CB D10) Which of the following numbers is biggest?(A)20×13(B)20×13(C)20×13(D)201×3(E) 2013- 4 Point Questions -11) Triangle RZT is generated by rotating the equilateral triangle AZCabout point Z. Angle β = ∠CZR = 70°. Determine angle α = ∠CAR.(A) 20° (B) 25° (C) 30° (D) 35° (E) 40°12) The figure on the right is made up of six unit squares. Its perimeter is 14 cm.Squares will be added to this figure in the same way until it is made up of 2013 unitsquares (zigzag: alternating bottom right and top right). How big is the perimeter ofthe newly created figure?(A) 2022 (B) 4028 (C) 4032 (D) 6038 (E) 805013) A and B are opposite vertices of a regular six-side shape, the points C and D are the mid-points of two opposite sides. The area of the regular six-sided shape is 60. Determine theproduct of the lengths of the lines AB and CD!(A) 40 (B) 50 (C) 60 (D) 80 (E) 10014) A class has written a test. If every boy had obtained 3 more points, the points average would be 1.2 points higher than now. Which percentage of the children in this class are girls?(A) 20% (B) 30% (C) 40% (D) 60% (E) There is too little information given to determine the answer.15) The sides of the rectangle ABCD are parallel to the co-ordinate axis. The rec-tangle lies below the x-axis and to the right of the y-axis, as shown in the diagram.For each of the points A, B, C, D the quotient(y-coordinate):(x- coordinate) is calculated.For which point will you obtain the smallest quotient?(A) A (B) B (C) C (D) D(E) It depends on the position of the rectangle and its side lengths.16) Today is Hans’ and his son’s birthday. Hans multiplies his age with the age of his son and obtains2013. In which year was Hans born?(A) 1952 (B) 1953 (C) 1981 (D) 1982(E) More information is needed to be able to answer this question.17) Tarzan wanted to draw a rhombus made up of two equilateral triangles. He drew the linesegments inaccurately. When Jane checked the measurements of the four angles shown, she seesthat they are not equally big (see diagram). Which of the five line segments in this diagram is thelongest?(A) AD (B) AC (C) AB (D) BC (E) BD18) Five consecutive positive integers have the following property: The sum of three of the numbers isas big as the sum of the other two. How many sets of 5 such numbers are there?(A) 0 (B) 1 (C) 2 (D) 3 (E) more than 319) How many different ways are there in the diagram shown, to get from point A to point Bif you are only allowed to move in the directions indicated?(A) 6 (B) 8 (C) 9 (D) 12 (E) 1520) Given a six-digit number whose digit sum is even and whose digit product is odd. Whichof the following statements are true for this number?(A) Two or four of the digits of this number are even.(B) There is no such number.(C) The number of odd digits of this number is odd.(D) The number can be made up of 6 different digits.(E) None of the statements (A) – (D) are correct.- 5 Point Questions -21) How many decimal places are necessary to write the number 11024000 as a decimal?(A) 10 (B) 12 (C) 13 (D) 14 (E) 102400022) The date 2013 is made up of four consecutive digits 0, 1, 2, 3. How many years before the year 2013 was the date last made up of four consecutive digits?(A) 467 (B) 527 (C) 581 (D) 693 (E) 99023) We are looking at rectangles where one side is of length 5.0 cm. Amongst those are some that can be cut into a square and a rectangle one of which has an area of 4,0 cm². How many such rectangles are there?(A) 1 (B) 2 (C) 3 (D) 4 (E) 524) “Sum change” is a procedure where in a set of three numbers, each number is replaced by the sum of the other two. So for instance {3, 4, 6} becomes the set {10, 9, 7} and this again becomes {16, 17, 19}. Let the starting point be the set {1, 2, 3}.How many such sum changes are necessary until the number 2013 appears in the set?(A) 8 (B) 9 (C) 10 (D) 2013 appears several times. (E) 2013 never comes up.25) Let Q be the number of square numbers amongst the natural numbers from 1 to 20136 and K the number of cubic numbers (powers of three) amongst the natural numbers from 1 to 20136. Which of the following holds true:(A) Q = 2013×K (B) 2Q = 3K (C) 3Q = 2K (D) Q = K (E) Q3= K226) Using the numbers 1, 2, 3, …, 22, 11 fractions a b are formed where each number is used exactly once. What is the maximum number of fractions with whole number values that can be obtained?(A) 11 (B) 10 (C) 9 (D) 8 (E) 727) Any three vertices of a regular 13-sided-shape are joined up to form a triangle. How many of these triangles contain the circumcentre of the 13-sided-shape?(A) 72 (B) 85 (C) 91 (D) 100 (E) another number28) A car starts in point A and drives on a straight road at 50 km/h. Every hour after that another car leaves point A with a speed 1 km/h faster than the one before. The last car leaves A 50 hours after the first car and drives with a speed of 100 km/h. What is the speed of the car that is leading 100 hours after the start of the first car?(A) 50 km/h (B) 66 km/h (C) 75 km/h (D) 84 km/h (E) 100 km/h29) 100 trees (oaks and birches) are standing in a row. The number of trees between any two oaks is not equal to 5. What is the maximum number of trees out of the 100 that can be oak trees?(A) 60 (B) 52 (C) 50 (D) 48 (E) This situation is not possible.30. A positive integer N is smaller than the sum of its three biggest true factors (N itself is not a true factor of N). Which of the following statements is true?(A) All such numbers N are divisible by 7.(B) All such numbers N are divisible by 6.(C) All such numbers N are divisible by 5.(D) All such numbers N are divisible by 4.(E) Such a number N does not exist.- 3 Point Questions -1. Which of the following numbers is biggest?(A) 2013 (B) 20+13(C) 2013(D) 2013(E) 20 ⋅ 132. The regular eight-sided shape on the right has sides of length 10. A circle touches all inscribeddiagonals of this eight-sided shape. What is the radius of this circle?(A) 10 (B) 7,5 (C) 5 (D) 2,5 (E) 23. The surface of a prism is made of 2013 faces. How many edges does the prism have?(A) 2011 (B) 2013 (C) 4022 (D) 4024 (E) 60334. The third root of333takes which value? (Note: ()c cb ba a=.)(A) 33(B) 3313−(C) 323(D) 233(E) 35. The date 2013 is made up of four consecutive digits 0, 1, 2, 3. How many years before the year 2013 was the date last made up of four consecutive digits?(A) 467 (B) 527 (C)581 (D) 693 (E) 9906. Let f be a linear function for which(2013)(2001)100f f−=gilt. holds true. What is the value of(2031)(2013)f f−?(A) 75 (B) 100 (C) 120 (D) 150 (E) 1807. We know that the relationship2 < x < 3 is valid for a number x. How many of the following statements are true in this case?2249429639023x x x x x<<<<<<<−<(A) 0 (B) 1 (C) 2 (D) 3 (E) 48. Each of six lone heros has captured wanted people. In total they have captured 20 wanted people: the first hero one wanted person, the second hero two wanted people, the third hero three wanted people. The fourth hero has captured more wanted people than any other hero. Determine the smallest number of wanted peoplethat the fourth hero could have captured, so that this statement could be true.(A) 7 (B) 6 (C) 5 (D) 4 (E) 39. Inside the cube lattice pictured on the side one can see a solid, non-seethrough pyramidABCDS with square base ABCD, whose top S is exactly in the middle of one edge of thecube. If you look at the pyramid from above, from below, from the front, from the back, fromthe right and from the left – which of the following views cannot be possible?(A) (B)(C) (D) (E)10. If a certain substance melts the volume increases by 112.By how much does the volume decrease if the substance solidifies again?(A) 110(B) 111(C) 112(D) 113(E) 114- 4 Point Questions -11. Ralf has a number of equally big plastic plates each in the form of a regular five sidedMathmatical Kangaroo 2013Group Student (Grade 11. and above)Austria - 21.3.2013。

2009E试卷(澳大利亚数学竞赛AMC-E：11-12年级中英文历年真题)

高级卷（11—12年级）考试时间：75分钟─────────────────────────────────高级卷(11-12年级)──────────────────────────────────1-10题，每题3分1. 算式(2000＋9)－(2000－9)等于（A ）4000 （B ）2018 （C ）3982 （D ）0 （E ）18────────────────────────────────── 2. 在右图中，x 的值等于（A ）140 （B ）122 （C ）80 （D ）90 （E ）98────────────────────────────────────────────────────────────────── 3. 方程y =kx 的图形通过点(2,1)−−，则k 的值等于（A ）2 （B ）2− （C ）4 （D ）12 （E ）12−────────────────────────────────── 4. 算式2(0.6)−等于（A ）0.36− （B ）0.036 （C ）925 （D ）259（E ）3.6────────────────────────────────── 5. 多项式(x －y )－2(y －z )+3(z －x )等于（A ）－2x －3y +5z （B ）－2x －3y －z （C ）4x ＋y －z （D ）4x ＋3y －z （E ）2x ＋3y －5z──────────────────────────────────────────────────────────────────── 6. 有一串珠子，最大的珠子在正中央而最小的珠子在两端。

珠子的尺寸由两端至中央逐渐增大，如图所示。

最小的珠子每枚价格为＄1、次小的珠子每枚价格为＄2、再次小的珠子每枚价格为＄3，依此类推。

已知这串珠子共有25枚珠子，请问支付＄200购买此串珠子应可找回多少钱？（A ）＄25 （B ）＄31 （C ）＄40 （D ）＄52 （E ）＄55140° 122° x °y x 7. 对于所有的正数a 、b ，规定1*a b a b=+，则1*(2*3)之值等于（A ）103 （B ）107 （C ）116 （D ）92 （E ）310──────────────────────────────────8. 右图所示为2y ax bx c =++的图像，其顶点在y 轴上。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

THURSDAY 1 AUGUST 2013SENIOR DIVISIONAUSTRALIAN S CHOOL YEARS 11 and 12TIME ALLOWED: 75 MINUTES©AMT P ublishing 2013 AMTT liMiTed Acn 083 950 341A ustrAliAn M AtheMAtics c oMpetitionsponsored by the c oMMonweAlth b AnkAn AcTiviTy of The AusTrAliAn MATheMATics TrusTNAMEYEAR TEACHERA u s T r A l i A n M A T h e M A T i c s T r u s T姓名：年级：监考老师：意事项一般规定1.未获监考老师许可之前不可翻开此测验题本。

2.各种通讯器材一律不得携入考场，不准使用电子计算器、计算尺、对数表、数学公式等计算器具。

作答时可使用直尺与圆规，以及两面全空白的草稿纸。

3.题目所提供之图形只是示意图，不一定精准。

4.最前25题为选择题，每题有五个选项。

最后题要求填入的答案为000至999的正整数。

题目一般而言是依照越来越难的顺序安排，对于错误的答案不会倒扣分数。

5.本活动是数学竞赛而不同于学校测验，别期望每道题目都会作。

考生只与同地区同年级的其它考生评比，因此不同年级的考生作答相同的试卷将不作评比。

6.请依照监考老师指示，谨慎地在答案卡上填写您的基本数据。

若因填写错误或不详所造成之后果由学生自行负责。

7.进入试场后，须等待监考老师宣布开始作答后，才可以打开题本进行答题。

作答须知1.限用B 或2B 铅笔填写答案。

2.请用B 或2B 铅笔在答案卡上（不是在题本上）将您认为正确选项的圆圈涂满。

3.您的答案卡将由计算机阅卷，为避免计算机误判，请不要在答案卡上其它任何地方涂划任何记号。

填写答案卡时，若需要修改，可使用软性橡皮小心擦拭，并确定答案卡上无残留痕迹。

特别约定为确保竞赛之公平性及认证成绩优异学生，AMC 主办单位保留要求考生重测之权利。

注意事项一般规定1.未获监考老师许可之前不可翻开此测验题本。

2.各种通讯器材一律不得携入考场，不准使用电子计算器、计算尺、对数表、数学公式等计算器具。

作答时可使用直尺与圆规，以及两面全空白的草稿纸。

3.题目所提供之图形只是示意图，不一定精准。

4.最前25题为选择题，每题有五个选项。

最后5题要求填入的答案为000至999的正整数。

题目一般而言是依照越来越难的顺序安排，对于错误的答案不会倒扣分数。

5.本活动是数学竞赛而不同于学校测验，别期望每道题目都会作。

考生只与同地区同年级的其它考生评比，因此不同年级的考生作答相同的试卷将不作评比。

6.请依照监考老师指示，谨慎地在答案卡上填写您的基本数据。

若因填写错误或不详所造成之后果由学生自行负责。

7.进入试场后，须等待监考老师宣布开始作答后，才可以打开题本进行答题。

作答须知1.限用B 或2B 铅笔填写答案。

2.请用B 或2B 铅笔在答案卡上（不是在题本上）将您认为正确选项的圆圈涂满。

3.您的答案卡将由计算机阅卷，为避免计算机误判，请不要在答案卡上其它任何地方涂划任何记号。

填写答案卡时，若需要修改，可使用软性橡皮小心擦拭，并确定答案卡上无残留痕迹。

特别约定为确保竞赛之公平性及认证成绩优异学生，AMC 主办单位保留要求考生重测之权利。

高级卷1-10题，每题3分1.算式0.612等于（A ）5（B ）0.5（C ）0.05 （D ）0.005（E ）0.00052.请问以下哪一项是直角三角形？（A ）（B ）（C ）（D ）（E ）3.一块农地本季的产量增加20%而达到114公吨。

请问上一季的产量是多少公吨？（A ）90（B ）91 （C ）93 （D ）94（E ）954.请问201320132013的立方根接近于下列哪一个数？（A ）600（B ）5000（C ）6000（D ）50000（E ）600005.若p =4b +26且b 为正整数，则p 不可能被下列哪一个数整除？（A ）2 （B ）4 （C ）5 （D ）6 （E ）76.一个数列的前五项依序为：1、2、1、1−、2−、…已知从第二项之后，每一项都是由前面一项减去再前面一项而得到的。

请问这个数列的前42项之总和是多少？（A ）0 （B ）4 （C ）12 （D ）24（E ）30345.24681012.13372所示。

请问下列哪一项可能是这条直线的方程？（A ）512120x y +−=（B ）410x y ++=（C ）3770x y −+=（D ）3220x y −+=（E ）2330x y +−=8.小孔与小克进行100 m 的赛跑，小孔赢了5 m(即当小孔抵达终点时，小克距离终点还有5 m)；小克与小杰进行100 m 的赛跑，小克赢了10 m 。

请问若是小孔与小杰进行100 m 的赛跑，小孔应会赢多少m ？（A ）15.5（B ）15 （C ）14.5（D ）14（E ）13.59.在牧场中共有两种动物：骆驼与羊驼，而牧场也有许多位管理员。

已知骆驼与羊驼的数量比为2：3及羊驼与管理员的数量比为8：1，请问所有的动物与管理员的数量比是什么？（A ）16：3（B ）13：1（C ）12：1（D ）40：3（E ）20：310.从计算器上显示的数为0开始，我进行五个步骤的计算，每一个步骤都是加1或是乘以2。

请问不可能是计算结果之最小的数是什么？（A ）11 （B ）10 （C ）9 （D ）8 （E ）711-20题，每题4分11.在一个箱子中共有三个袋子。

一个袋子内有一颗白石子及三颗黑石子，另一个袋子内也是有一颗白石子及三颗黑石子，而第三个袋子内则有一颗白石子及四颗黑石子。

现从箱子中任选一个袋子后，再从袋子里任取一颗石子，请问取出白石子的机率是多少？（A ）730（B ）313（C ）19（D ）14（E ）113612.在坐标平面上，有一个由x 轴、y 轴、直线x =2与方程()y f x =所围成的区域，其中101()112x f x x ≤≤⎧⎪=⎨+<≤⎪⎩當當请问此区域的面积为多少平方单位？（A ）52（B ）14π+（C ）12π+（D ）2π+（E ）24π+13.将三个边长分别为3 cm 、5 cm 及8 cm 的正方形如下图所示的方式排列：请问阴影部分的梯形面积为多少cm 2？（A ）12（B ）736（C ）554（D ）14 （E ）25214.一个周长为20 cm 的正方形内接于一个周长为28 cm 的正方形。

请问一个在内部正方形的顶点与一个在外部正方形的顶点之最大距离是多少cm ？（A（B ）752（C ）8 （D（E）15.用三个非零且互不同的数码可以组成六个不同的二位数。

已知这六个数中的五个数之和为100，请问第六个数是什么？（A ）23（B ）32 （C ）45 （D ）54（E ）6716.掷三支飞镖进入一个3×3的方格靶内，每支飞镖都射入不同的小方格内。

经过每次投掷后，飞镖射入剩余的小方格之机率都相等。

请问最后三支飞镖所在的方格形成水平线、铅垂线或对角线的机率是什么？（A ）163（B ）221（C ）19（D ）142（E ）88117.一个箱子的每个面都是矩形且边长都是整数，若主对角线XY = 9，请问这个箱子的体积最大可能值是什么？（A ）32（B ）81 （C ）90 （D ）108（E ）11218.已知x 、y 都是整数且0x ≥，请问满足方程2222169x xy y −+=的不同数对(x ,y )共有多少组？（A ）2（B ）4 （C ）5 （D ）7 （E ）819.一个跑道所围成的区域是由一个矩形连接二个半圆所形成的，如下图所示。

若跑道全长必须恰好为400 m ，请问此矩形的最大面积为多少m 2？（A ）2160000(2)π+（B ）20000π（C ）30000π（D ）10000（E ）40000πXY20.请问将2013写成二个或二个以上的连续正整数之和的方式共有多少种？（A ）5 （B ）6 （C ）7 （D ）8 （E ）921-25题，每题5分21.若23x x =+，则5x 等于（A ）712x + （B ）127x + （C ）1717x +（D ）1921x + （E ）2119x +22.下图矩形PQRS 的中心为点C ，已知PQ = 4、PS = 12。

二个涂上阴影且半径都是1的圆分别与PS 相切于点U 与点V ，其中PU = 1、PV = 4。

若直线CW 将非阴影区域切为面积相等的二块区域，请问PW 的长度是什么？（A ）27（B ）25（C ）14（D ）13（E ）1223.若三个非零的实数x 、y+则111x y z ++之值一定等于（A ）0 （B ）1 （C ）1−（D ）xyz （E ）x +y +z24.黑板上写了一个二位数，对于这个数有五位学生分别做了以下叙述：A ：此数是质数。

B ：此数可以写成二个完全平方数之和。

C ：此数至少有一个数码是7。

D ：将此数的数码顺序颠倒后所得的数是奇数且是个合数。

E ：此数数与某个质数之差为2。

若恰有一个学生是错的，请问是哪一位？（A ）A（B ）B （C ）C （D ）D（E ）EPW25.半径分别为1与2的二个球互相外切于点P 。

一个经过点P 的平面将这二个球所围成的区域分割为体积比为1：2(图中无阴影部份：阴影部份)的二块。

请问此平面将小球分割成二块的体积比(图中阴影部份：无阴影部份)是什么？（A ）1：2（B ）4：9（C ）1：3（D ）4：11 （E ）2：5问题26～30的答案为000～999之间的整数，请将答案填在答案卡上对应的位置。

第26题占6分，第27题占7分，第28题占8分，第29题占9分，第30题占10分。

26.在一场曲棍球比赛中，如果在比赛中两支交手球队的进球数之差从未超过2时，则称这两支球队「实力相当」。

若两支球队共进12球且两支球队一直处于「实力相当」的情况，请问整个球赛共有多少种不同可能的赛况？27.已知点X 在正方形PQRS 内部使得点X 与点R 的距离为25 m 、与点S 的距离为51 m 、与点P 的距离为53 m 。

若点X 与各条边之距离都是整数m ，请问△PQX 的面积为多少m2？P255351X SR Q28.正整数N 的数码都是0或1，将N 除以37时所得的余数为18。

请问N 的所有数码中，最少有多少个1？29.如图所示，点X 、Y 及Z 位于△PQR 的边上使得QZ ：ZY ：YR = 1：2：3且PX ：XR = 4：5若QS = 11 cm ，请问ST 的长度是多少cm ？30.在坐标平面上，一个锐角三角形的三个顶点的坐标都是不同的整数且没有任何一条边与坐标轴平行。

2013E试卷(澳大利亚数学竞赛AMC-E：11-12年级中英文历年真题)

合集下载

AMC Senior 2008(澳大利亚数学竞赛AMC-E：11-12年级中英文历年真题)

2013澳大利亚高考数学试题评分标准

VCE考试数学2013试题1

澳大利亚数学竞赛小学中年级(3--4年级)(2013年)

2013年AMC8竞赛真题(英文版)

澳大利亚数学竞赛小学高年级(5—6)(2013年)

AMC Intermediate 2013AMC-D：9-10年级中文历年真题

AMC Senior 2011(澳大利亚数学竞赛AMC-E：11-12年级中英文历年真题)

2013A试卷AMC-A：澳大利亚数学3-4年级中文历年真题

AMC8(美国数学竞赛)历年真题、答案及中英文解析

2013年AMC_12真题(B)

2013年AMC 10B竞赛真题及答案(英文版)

美国中学数学竞赛(AMC12-12级)(附答案)

澳大利亚数学竞赛小学中年级(3--4年级)(2013年)

澳大利亚kangaroo袋鼠数学竞赛试题及答案grade3-11 2013年

2009E试卷(澳大利亚数学竞赛AMC-E：11-12年级中英文历年真题)

相关主题

文档推荐

最新文档