Heat Transfer热传作业3解答

热能的传递和传导练习题热能的传递和传导是热学领域中的重要概念。

在生活中，我们经常会遇到热能的传递和传导现象，而了解这些现象对于我们的日常生活和工作都有重要的意义。

下面是一些关于热能传递和传导的练习题，通过这些题目的回答，我们可以更好地理解这些概念。

练习题一：传热方式1. 什么是热传导？它是如何发生的？2. 什么是热传递？列举出三种不同的热传递方式，并简要解释每一种方式的原理。

3. 列举一些你生活中常见的热传递现象，并解释它们所属的传热方式。

练习题二：传热因素1. 影响热传导的主要因素有哪些？2. 温度是如何影响热传导的？请用适当的例子进行说明。

3. 什么是热导率？它与热传导的关系是怎样的？练习题三：传热计算1. 如何计算导热方程？2. 如果一根铜棒的热导率为400 W/(m·K)，棒的长度为50 cm，横截面积为0.05 m²，两端温度差为100 K，求通过铜棒的热流量。

3. 如果用蜡烛来加热室内面积为20 m²的房间，室内温度为15°C，外界温度为5°C。

假设房间没有绝热层，蜡烛燃烧产生的热量全部传递给室内空气，计算蜡烛需要燃烧多长时间才能使房间温度升高到25°C。

练习题四：传热应用1. 热传导在哪些领域被广泛应用？请列举三个例子，并简要介绍每个例子中的热传导应用。

2. 利用热传导的原理，解释为什么在冬天，大地比空气温暖？3. 热传导对于建筑材料的选择有哪些影响？请列举两个例子。

练习题五：传热实验1. 设计一个简单的实验，用来观察热传导现象。

2. 描述实验步骤和所需材料。

3. 分析实验结果，得出结论，并解释现象背后的物理原理。

以上是关于热能的传递和传导练习题，通过回答这些问题，我们可以更好地理解热学中的基本概念。

热能的传递和传导在我们的生活中无处不在，了解和掌握这些概念，可以帮助我们更好地利用热能，改善生活和工作环境。

希望通过这些练习题的完成，能对读者有所帮助。

热传递试题及答案一、选择题1. 热传递的三种基本方式是什么？A. 热传导、热对流、热辐射B. 热传导、热对流、热交换C. 热传导、热交换、热辐射D. 热对流、热交换、热辐射答案：A2. 下列哪种物质的导热性能最好？A. 木头B. 玻璃C. 金属D. 水答案：C3. 热对流主要发生在哪种介质中？A. 固体B. 液体C. 气体D. 真空答案：B4. 热辐射不需要介质，其传播速度是多少？A. 3×10^8 m/sB. 3×10^5 m/sC. 3×10^2 m/sD. 3×10^1 m/s答案：A二、填空题1. 热传递的三种基本方式包括________、________和________。

答案：热传导、热对流、热辐射2. 热传导是通过________进行热量传递的。

答案：物质内部分子的振动和碰撞3. 热对流是依靠________的流动来传递热量的。

答案：流体4. 热辐射是物体通过________向外传递能量的。

答案：电磁波三、简答题1. 请简述热传导的基本原理。

答案：热传导是热量通过直接接触的物体内部分子振动和碰撞传递的过程，从温度高的区域传递到温度低的区域。

2. 热对流与热传导的主要区别是什么？答案：热对流依赖于流体的流动来传递热量，而热传导是通过物体内部分子的振动和碰撞传递热量，不需要流体的流动。

四、计算题1. 假设一个金属棒长2米，横截面积为0.01平方米，热传导系数为200 W/(m·K)，两端温差为100K，求金属棒的热传导率。

答案：Q = k * A * ΔT / L = 200 * 0.01 * 100 / 2 = 100 W2. 一个封闭的容器内装有水，水的比热容为4186 J/(kg·K)，质量为5kg，初始温度为20℃，加热到100℃，求加热过程中需要的热量。

答案：Q = mcΔT = 5 * 4186 * (100 - 20) = 1.8344 * 10^6 J。

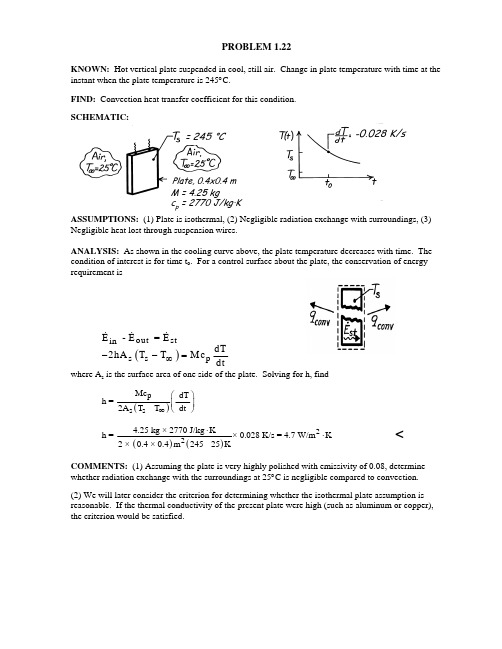
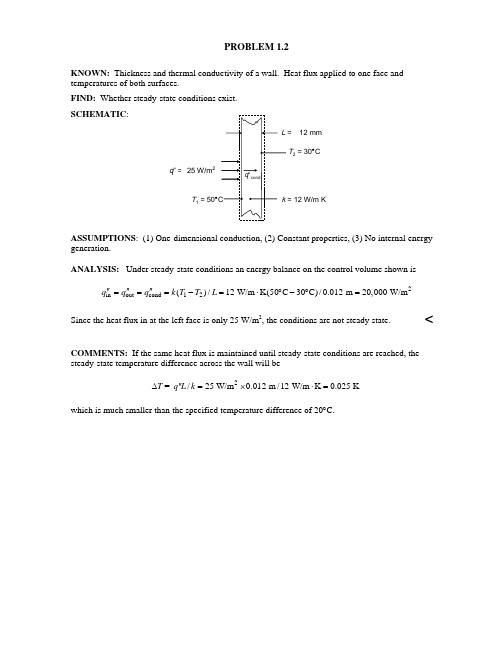
PROBLEM 1.22KNOWN: Hot vertical plate suspended in cool, still air. Change in plate temperature with time at the instant when the plate temperature is 245°C.FIND: Convection heat transfer coefficient for this condition.SCHEMATIC:ASSUMPTIONS: (1) Plate is isothermal, (2) Negligible radiation exchange with surroundings, (3)Negligible heat lost through suspension wires.ANALYSIS: As shown in the cooling curve above, the plate temperature decreases with time. The condition of interest is for time t o . For a control surface about the plate, the conservation of energy requirement is()outst in s s pE - E = E dT 2hA T T Mc dt∞−−=&&&where A s is the surface area of one side of the plate. Solving for h, find()ps s Mc -dT h =2A T - T dt ∞⎛⎞⎜⎟⎝⎠()()224.25 kg × 2770 J/kg K h = × 0.028 K/s = 4.7 W/m K 2 × 0.4 × 0.4m 245 - 25K⋅⋅<COMMENTS: (1) Assuming the plate is very highly polished with emissivity of 0.08, determinewhether radiation exchange with the surroundings at 25°C is negligible compared to convection.(2) We will later consider the criterion for determining whether the isothermal plate assumption is reasonable. If the thermal conductivity of the present plate were high (such as aluminum or copper), the criterion would be satisfied.-0.022 K/s-0.028 K/s = 245 °CPlate, 0.3×0.3 m M = 4.25 kgc p = 2770 J/kg·KPlate, 0.4x0.4 mPROBLEM 1.29KNOWN: Air and wall temperatures of a room. Surface temperature, convection coefficient and emissivity of a person in the room.FIND: Basis for difference in comfort level between summer and winter.ASSUMPTIONS: (1) Person may be approximated as a small object in a large enclosure.ANALYSIS: Thermal comfort is linked to heat loss from the human body, and a chilled feeling is associated with excessive heat loss. Because the temperature of the room air is fixed, the different summer and winter comfort levels cannot be attributed to convection heat transfer from the body. In both cases, the heat flux isSummer and Winter : ()22q h T T 2 W/m K 12 C 24 W/m convs ′′=−=⋅×=∞oHowever, the heat flux due to radiation will differ, with values of Summer : ()()448244442q T T 0.9 5.6710W/m K 305300K 28.3 W/m rads sur εσ−′′=−=××⋅−= Winter :()()448244442q T T 0.9 5.6710W/m K 305287K 95.4 W/m rad s surεσ−′′=−=××⋅−=There is a significant difference between winter and summer radiation fluxes, and the chilled condition is attributable to the effect of the colder walls on radiation.COMMENTS: For a representative surface area of A = 1.5 m 2, the heat losses are q conv = 36 W, q rad(summer) = 42.5 W and q rad(winter) = 143.1 W. The winter time radiation loss is significant and if maintained over a 24 h period would amount to 2,950 kcal.PROBLEM 1.44KNOWN: Radial distribution of heat dissipation in a cylindrical container of radioactive wastes. Surface convection conditions.FIND: Total energy generation rate and surface temperature.SCHEMATIC:ASSUMPTIONS: (1) Steady-state conditions, (2) Negligible temperature drop across thin container wall.ANALYSIS: The rate of energy generation is()()r 2g o o 022g o o o E qdV=q 1-r/r 2rLdr E 2Lqr /2r /4o ⎡⎤=⎢⎥⎣⎦=−∫∫&&&&&ππor per unit length,&&.′=E q r 2g o o 2π <Performing an energy balance for a control surface about the container yields, at an instant,&&′−′=E E g outand substituting for the convection heat rate per unit length,()()2o o o s qr h 2r T T 2∞=−&ππT T qr 4hs o o =+∞&. <COMMENTS: The temperature within the radioactive wastes increases with decreasing r from T s at r o to a maximum value at the centerline.PROBLEM 1.76KNOWN: Thickness and thermal conductivity, k, of an oven wall. Temperature and emissivity, ε, of front surface. Temperature and convection coefficient, h, of air. Temperature of large surroundings.FIND: (a) Temperature of back surface, (b) Effect of variations in k, h and ε.SCHEMATIC:ASSUMPTIONS: (1) Steady-state, (2) One-dimensional conduction, (3) Radiation exchange with large surroundings.ANALYSIS: (a) Applying an energy balance, Eq. 1.13 to the front surface and substituting the appropriate rate equations, Eqs. 1.2, 1.3a and 1.7, find()()44122sur 2T T k h T T T T Lεσ∞−=−+−.Substituting numerical values, find()()1220.05m W 448T T 20100K 240.7W/m K m K W 0.8 5.6710400K 300K 200K m K −−=⋅⋅+××−=⋅⎤⎡⎡⎤⎥⎢⎢⎥⎣⎦⎣⎦.Since 2T = 400 K, it follows that 1T = 600 K.<(b) Parametric effects may be evaluated by using the IHT First Law Model for a Nonisothermal Plane Wall. Changes in k strongly influence conditions for k < 20 W/m ⋅K, but have a negligible effect for larger values, as 2T approaches 1T and the heat fluxes approach the corresponding limiting values100200300400Thermal conductivity, k(W/m.K)300400500600T e m p e r a t u r e , T 2(K )100200300400Thermal conductivity, k(W/m.K)0200040006000800010000H e a t f l u x , q ''(W /m ^2)Conduction heat flux, q''cond(W/m^2)Convection heat flux, q''conv(W/m^2)Radiation heat flux, q''rad(W/m^2)Continued…PROBLEM 1.76 (Cont.)The implication is that, for k > 20 W/m ⋅K, heat transfer by conduction in the wall is extremely efficient relative to heat transfer by convection and radiation, which become the limiting heat transfer processes. Larger fluxes could be obtained by increasing ε and h and/or by decreasing T ∞ and sur T .With increasing h, the front surface is cooled more effectively (2T decreases), and although radq ′′ decreases, the reduction is exceeded by the increase in convq ′′. With a reduction in 2T and fixed values of k and L, condq ′′ must also increase.100200Convection coefficient, h(W/m^2.K)400500600T e m p e r a t u r e , T 2(K )100200Convection coefficient, h(W/m^2.K)0100002000030000H e a t f l u x , q ''(W /m ^2)Conduction heat flux, q''cond(W/m^2)Convection heat flux, q''conv(W/m^2)Radiation heat flux, q''rad(W/m^2)The surface temperature also decreases with increasing ε, and the increase in rad q ′′ exceeds the reduction in convq ′′, allowing cond q ′′ to increase with ε. 00.20.40.60.81Emissivity550555560565570575T e m p e r a t u r e , T 2(K )0.20.40.60.81Emissivity0200040006000800010000H e a t f l u x , q ''(W /m ^2)Conduction heat flux, q''cond(W/m^2)Convection heat flux, q''conv(W/m^2)Radiation heat flux, q''rad(W/m^2)COMMENTS: Conservation of energy, of course, dictates that irrespective of the prescribed conditions, cond conv radq q q ′′′′′′=+.PROBLEM 1.77KNOWN: Temperatures at 15 mm and 30 mm from the surface and in the adjoining airflow for a thick stainless steel casting.FIND: Surface convection coefficient, h. SCHEMATIC:ASSUMPTIONS: (1) Steady-state, (2) One-dimensional conduction in the x-direction, (3) Constant properties, (4) Negligible generation.ANALYSIS: From a surface energy balance, it follows that′′=′′q q cond convwhere the convection rate equation has the form()0convq h T T ,∞′′=−and ′′q cond can be evaluated from the temperatures prescribed at surfaces 1 and 2. That is, from Fourier’s law,()()12cond212condT T q k x x 6050C Wq 1510,000 W/m .m K 30-1510m−′′=−−′′==⋅×oSince the temperature gradient in the solid must be linear for the prescribed conditions, it follows thatT o = 70°C. Hence, the convection coefficient ish =q T T cond 0′′−∞2210,000 W/m h 333 W/m K 30°C==⋅<COMMENTS: The accuracy of this procedure for measuring h depends strongly on the validity of the assumed conditions.T 1= 60°C T 2= 50°C 3015PROBLEM 1.81KNOWN: Conditions associated with surface cooling of plate glass which is initially at 600°C. Maximum allowable temperature gradient in the glass.FIND: Lowest allowable air temperature, T ∞.SCHEMATIC:ASSUMPTIONS: (1) Surface of glass exchanges radiation with large surroundings at T sur = T ∞, (2) One-dimensional conduction in the x-direction.ANALYSIS: The maximum temperature gradient will exist at the surface of the glass and at the instant that cooling is initiated. From the surface energy balance, Eq. 1.13, and the rate equations, Eqs. 1.1, 1.3a and 1.7, it follows that()()44s s sur dT -kh T T T T 0dxεσ∞−−−−=or, with (dT/dx)max = -15°C/mm = -15,000°C/m and T sur = T ∞,()C 2W W1.415,0005873T K m K m m K ∞⎡⎤−−=−⎢⎥⋅⎢⎥⋅⎣⎦o844424W0.8 5.6710873T K .m K −∞⎡⎤+××−⎢⎥⎣⎦⋅T ∞ may be obtained from a trial-and-error solution, from which it follows that, for T ∞ = 618K,21000127519730,,.W m W m Wm222≈+Hence the lowest allowable air temperature isT K =345C.∞≈618o<COMMENTS: (1) Initially, cooling is determined primarily by radiation effects.(2) For fixed T ∞, the surface temperature gradient would decrease with increasing time into the cooling process. Accordingly, T ∞ could be decreasing with increasing time and still keep within themaximum allowable temperature gradient.。

青岛版五年级上册科学第3单元热的传递综合训练一、选择题1.太阳能热水器的集热管是深色的，这是因为（）。

A.深色美观B.深色吸收辐射热能力强C.防止爆裂2.将大小、形状、厚薄相同的陶瓷勺和金属勺，同时放入盛有热水的杯子中，稍等片刻后用手触摸未浸入水中的部分，两种勺的温度（）oA.陶瓷勺比金属勺热B.金属勺比陶瓷勺热C.一样热3.在探究“热是怎样在固体中传递的”实验中，火柴的作用是（）。

A.便于观察热的传递B.加快热传递的速度4.物体以电磁波的形式向外发射热能的过程叫（）oA.热对流B.热辐射C.热传递5.以下选项中不属于热辐射在生活中应用的是（）«A.喝热水取暖B.用“小太阳”取暖C.篝火取暖D.微波炉加热食物6.为了使整个房间更暖和，暖气片要安装在房间的（）。

A.下方B.上方C.中间7.不同材料的导热性是不一样的，以下材料中按导热性从强到弱的顺序排列正确的是（）。

A.铜一铝一铁B.铁一铝一铜C.铁一铜一铝8.下列选项中，体现热对流的是（）0A.用小太阳电热器取暖B.夏天开空调会让整个房间凉爽9.工厂里安装排气扇大都安装在厂房的上部，这是因为（）。

A.热空气向上升B.美观C.安全10.在一壶水被加热的过程中，下面的热水会不断上浮的原因是（）oA.体积不变，重量减轻B.体积增大，密度变大C.体积增大，密度变小二、填空题11.不同颜色的物体吸收辐射热的能力（）o12.在阳光下，戴黑手套的手的温度要比戴白手套的手的温度（）。

13.双手握住一个装有热水的杯子，手会慢慢地（）起来。

14.不同颜色的物体吸收辐射热的能力不一样。

一般来说，________ 色物体比___________ 色物体吸收辐射热的能力强。

15.比较实验中测得的温度数值，找出数值变化的（）,得出（）就是在分析数据。

16.在探究“热在水中是怎样传递的”实验中，木屑会在水中游动起来，说明热在水中是通过（）的方式传递的。

17.太阳的热直接传递到地球上的方式是o18.纸张放在烧红的炉盖上会被点燃，是由于（）；纸张悬挂在烧红的炉盖上方不远处会上下摆动，是由于（）o三、判断题19.双手不停对搓感到暖和，是由于热传递使温度升高的。

2015/03/12, M3510A, Heat Transfer, Homework III, Due 2015/03/19Problem 2.5 Assume steady-state, one-dimensionalheat conduction through the symmetric shape shown.Assuming that there is no internal heat generation,derive an expression for the thermal conductivity()k x for these conditions: ()()1A x x =-,()()330012T x x x =--, and q = 6000 W, where A is in square meters, T in kelvins, and x in meters.Problem 2.16 Steady-state, one dimensionalconduction occurs in a rod of constant thermalconductivity k and variable cross-sectional area()0ax x A x A e =, where 0A and a are constants. Thelateral surface of the rod is well insulated. (a) Write anexpression for the conduction heat rate, ()x q x . Usethis expression to determine the temperaturedistribution ()T x . (b) Now consider conditions for which thermal energy is generated in therod at a volumetric rate ()0exp q q ax ''''''=-, where 0q ''' is a constant. Obtain an expression for ()x q x when the left face (x = 0) is well insulated.Problem 2.28 Uniform internal heat generation at 73610W m q '''=⨯ is occurring in a cylindrical nuclear reactor fuel rod of 60-mm diameter, and under steady-state conditions the temperature distribution is of the form ()52900 5.2610T r r =-⨯⨯, where T is in degrees Celsius and r is in meters. The fuel rod properties are 30W m K k =⋅, 31100kg m ρ=, and 800J kg K P c =⋅. (a) What is the rate of heat transfer per unit length of the rod at r = 0 (the centerline) and at r = 30 mm (the surface)? (b) If the reactor power level is suddenly increased to 83210W m q '''=, what is the initial time rate of temperature change at r = 0 and r = 30 mm?Problem 2.40 The steady-state temperature distribution in a one-dimensional wall of thermal conductivity k and thickness L is of the form 32T ax bx cx d =+++. Derive expressions for the heat generation rate per unit volume in the wall and the heat fluxes at the two wall faces (x = 0, L).Problem 2.43 The cylindrical systemillustrated has negligible variation oftemperature in the r- and z-directions.Assume that o i r r r ∆=- is small comparedto i r , and denote the length in thez-direction, normal to the page, as L . (a)Beginning with a properly defined controlvolume and considering energy generationand storage effects, derive the differentialequation that prescribes the variation in temperature with the angular coordinate φ. (b) For steady-state conditions with no internal heat generation and constant properties, determine the temperature distribution ()T φ in terms of the constants 1T , 2T , i r , and o r . (c) For the conditions of part (b) write the expression for the heat rate q φ.Problem 2.51 A Spherical shell of inner and outer radii i r and o r , respectively, contains heat-dissipating components, and at a particular instant the temperature distribution in the shell is known to be of the form ()12C T r C r=+. Are conditions steady-state or transient? How do the heat flux and heat rate vary with radius?。

2014/03/18, M3510A, Heat Transfer, Quiz I1. A cylinder of radius o r , length L , and thermal conductivity k is immersed in a fluid of convection coefficient h and unknown temperature T ∞. At a certain instant the temperature distribution in the cylinderis ()2T r a br =+, where a and b are constants. Obtain an expression for the fluid temperature.2. The temperature distribution in a cylinder of thermal conductivity k is of the form432T a r b c d t φ=⨯+⨯++⨯, where a, b, c, and d are constants. Derive expressions for the heat generation rate per unit volume in the cylinder.3. The temperature distribution across a wall 1 m thick at a certain instant of time is given as()290030050T x x x =--, A uniform heat generation, 1000q '''=3W m , is present in the wall of area 102m having the properties 1600ρ=3kg m , 40k =W m K ⋅, and 4c =kJ kg K ⋅. Determine the rate of change of energy storage in the wall.4. An experiment to determine the convection coefficient associated with airflow over the surface of a thick stainless steel casting involves the insertion of thermocouples into the casting at distances of 10 and 20 mm from the surface along a thermal conductivity of 10W m K ⋅. If the thermocouples measure temperatures of 60 and 40℃ in the steel when the air temperature is 200℃, what is the convection coefficient?5. An uninsulated steam pipe passes through a room in which the air and walls are at 25℃. The outsidediameter of the pipe is 70 mm, and its surface temperature and emissivity are 200℃ and 0.8, respectively. If the coefficient associated with free convection heat transfer from the surface to the air is 152W m K ⋅, what is the rate of heat loss from the surface per unit length of pipe?6. The wall of an oven used to cure plastic parts is of thickness 0.1L =m and is exposed to largesurroundings and air at its outer surface. The air and the surroundings are at 300 K. If the temperature of the outer surface is 500 K and its convection coefficient and emissivity are 50h =2W m K ⋅ and 0.6ε=, respectively, what is the temperature of the inner surface if the wall has a thermal conductivity of 1k =W m K ⋅?7. Consider a carton of milk that is refrigerated at a temperature of 5m T =℃. The kitchen temperature on a hot summer day is 30T ∞=℃. If the four side of the carton are of height and width 150L =mm and 80w =mm, respectively, determine the heat transferred to the milk carton as it sits on the kitchen counter for durations of t = 300 s before is it returned to the refrigerator. The convection coefficient associated with natural convection on the sides of the carton is 10h =2W m K ⋅. The surface emissivity is 0.90. Assume the milk carton temperature remains at 5℃ during the process, the top and bottom surfaces are well insulated, and the wall temperature of the room is 30℃.8. An electric resistance heater is embedded in a long cylinder of diameter 10 mm. When water with a temperature of 20℃ and velocity of 1 m/s flows crosswise over the cylinder, the power per unit length required to maintain the surface at a uniform temperature of 100℃ is 10 kW/m. When air, also at 20℃, but with a velocity of 10 m/s is flowing, the power per unit length required to maintain the same surfacetemperature is 400 W/m. Calculate and compare the convection coefficients for the flows of water and air.9. The heat flux that is applied to the left face of a plane wall is 25q ''=2W m . The wall is of thickness 12L =mm and of thermal conductivity 12k =W m K ⋅. If the surface temperatures of the wall aremeasured to be 50℃ on the left side and 30℃ on the right side, determine if steady-state conditions exist?10. Two-dimensional, steady-state conduction occurs in a hollow cylindrical solid of thermal conductivity 22k =W m K ⋅, outer radius 1.5o r =m and overall length 28o z =m, where the origin of the coordinate system is located at the midpoint of the center line. The inner surface of the cylinder is insulated, and the temperature distribution within the cylinder has the form ()22,2015012ln 300T r z r r z =-+--. Determine the inner radius i r of the cylinder.T T T T k k k q c x x y y z z tρ⎛⎫∂∂∂∂∂∂∂⎛⎫⎛⎫'''+++= ⎪ ⎪ ⎪∂∂∂∂∂∂∂⎝⎭⎝⎭⎝⎭ 211T T T T kr k k q c r r r z z tr ρφφ⎛⎫∂∂∂∂∂∂∂⎛⎫⎛⎫'''+++= ⎪ ⎪ ⎪∂∂∂∂∂∂∂⎝⎭⎝⎭⎝⎭ 22222111sin sin sin T T T T kr k k q c r r t r r r θρφφθθθθ⎛⎫∂∂∂∂∂∂∂⎛⎫⎛⎫'''+++= ⎪ ⎪ ⎪∂∂∂∂∂∂∂⎝⎭⎝⎭⎝⎭ sin r T k T k T q kq q r r r θφθθφ∂∂∂''''''=-=-=-∂∂∂ r z T k T T q kq q k r r z φφ∂∂∂''''''=-=-=-∂∂∂ x y z T T T q k q kq k x y z ∂∂∂''''''=-=-=-∂∂∂ ()()()()d f x dx f x f x dx dx +=+⋅。

《化工传递过程导论》课程第九次作业解题参考第5章 热量传递及其微分方程1. 某不可压缩的黏性流体层流流过与其温度不同的无限宽度的平板壁面。

设流动为定态，壁温及流体的密度、黏度等物理性质恒定。

试由方程(5-13a)出发，简化上述情况的能量方程，并说明简化过程的依据。

解：课本(5-13a)式如下：222222()x y z T T T T T T T u u u t x y z x y zα∂∂∂∂∂∂∂+++=++∂∂∂∂∂∂∂ 由题意可知，定态流动0Tt∂⇒=∂。

在直角坐标系中，三维方向对应长、宽、高，题中“无限宽度的平板壁面”则可认为是在宽这个维度上无限，姑且设定此方向垂直于纸面且为z 方向，故可认为题意所指流动过程为二维流动，且0z u = 且2200T Tz z∂∂=⇒=∂∂则(5-13a)式可简化为2222()x y T T T Tu u x y x yα∂∂∂∂+=+∂∂∂∂ 如果引入热边界层概念，则基于尺度和量级的考虑，可进一步简化上式为22x y T T T u u x y yα∂∂∂+=∂∂∂ 其中，y 方向为垂直主流方向（x ）的距壁面的距离。

2. 假定人对冷热的感觉是以皮肤表面的热损失（刘辉注：换言之，是传热或散热速率）作为衡量依据。

设人体脂肪层的厚度为3mm ，其内表面温度为36℃且保持不变。

在冬天的某一天气温为-15℃。

无风条件下裸露皮肤表面与空气的对流传热系数为25W/(m 2·K)；有风时，表面对流传热系数为65W/(m 2·K)。

人体脂肪层的导热系数k ＝0.2W/(m ·K)。

试确定：(a) 要使无风天的感觉与有风天气温-15℃时的感觉一样（刘辉注：换言之，是传热或散热速率一样），则无风天气温是多少？(b) 在同样是-15℃的气温下，无风和刮风天，人皮肤单位面积上的热损失（刘辉注：单位面积上的热损失就是传热通量）之比是多少？解：（a ）此处，基本为对象是：人体皮下为脂肪层，层内传热为导热；体外或体表之外暴露在流动的空气中，紧邻表面之上为对流传热。

2015/03/05, M3510A, Heat Transfer, Homework II, Due 2015/03/12Problem 1.22 The free convection heat transfer coefficient on a thin hot vertical plate suspended in still air can be determined from observations of the change in plate temperature with time as it cools. Assuming the plate is isothermal and radiation exchange with its surroundings is negligible, evaluate the convection coefficient at the instant of time when the plate temperature is 245℃ and the change in plate temperature with time (dT dt ) is -0.028 K/s. The ambient air temperature is 25℃ and the plate measures 0.4 x 0.4 m with a mass of 4.25 kg and a specific heat of 2770J kg K ⋅Problem 1.29 Under conditions for which the same room temperature is maintained by a heating or cooling system, it is not uncommon for a person to feel chilled in the winter but comfortable in the summer. Provide a plausible explanation for this situation (with supporting calculations) by considering a room whose air temperature is maintained at 20℃ throughout the year, while the walls of the room are nominally at 27℃ and 14℃ in the summer and winter, respectively. The exposed surface of a person in the room may be assumed to be at a temperature of 32℃ throughout the year and to have an emissivity of 0.90. The coefficient associated with heat transfer by natural convection between the person and the room air is approximately 22W m K ⋅.Problem 1.44 Radioactive wastes are packed in a long, thin-walled cylindrical container. The wastes generate thermal energy non-uniformly according to the relation ()21o o q q r r ⎡⎤=-⎣⎦, where q is the local rate of energy generation per unit volume, o q is a constant, and o r is the radius of the container. Steady-state conditions are maintained by submerging the container in a liquid that is at T ∞ and provides auniform convection coefficient h . Obtain an expression for the total rate at which energy is generated in a unit length of the container. Use this result to obtain an expression for the temperature s T of the containerwall. Problem 1.76 The wall of an oven used to cure plastic parts is of thickness 0.05L =m and is exposed to large surroundings and air at its outer surface. The air and the surroundings are at 300 K. If the temperature of the outer surface is 400 K and its convection coefficient and emissivity are 20h =2W m K ⋅ and 0.8ε=, respectively, what is the temperature of the inner surface if the wall has a thermal conductivity of 0.7k =W m K ⋅?Problem 1.77 An experiment to determine the convection coefficient associated with airflow over thesurface of a thick stainless steel casting involves the insertion of thermocouples into the casting at distances of 15 and 30 mm from the surface along a thermal conductivity of 15W m K ⋅. If the thermocouplesmeasure temperatures of 60 and 50℃ in the steel when the air temperature is 100℃, what is the convection coefficient?Problem 1.81 During its manufacture, plate glass at 600℃ is cooled by passing air over its surface such that the convection heat transfer coefficient is 5h =2W m K ⋅. To prevent cracking, it is known that the temperature gradient must not exceed 15℃/mm at any point in the glass during the cooling process. If the thermal conductivity of the glass is 1.4W m K ⋅ and its surface emissivity is 0.8, what is the lowesttemperature of the air that can initially be used for the cooling? Assume that the temperature of the air equals that of the surroundings.。

2015/04/09, M3510A, Heat Transfer, Homework VI, Due 2015/04/16Problem 3.111 Copper tubing isjoined to a solar collector plate ofthickness t , thermal conductivity k ,and the working fluid maintains thetemperature of the plate above thetubes at o T . There is a uniform netradiation heat flux radq '' to the top surface of the plate, while the bottom surface is well insulated. The top surface is also exposed to a fluid at T ∞ that provides for a uniform convection coefficient h . Derive the differential equation that governs the temperature distribution ()T x in the plate.Problem 3.122 A rod of diameter25D =mm and thermal conductivity60k =W m K ⋅ protrudes normallyfrom a furnace wall that is at200w T =℃ and is covered byinsulation of thickness 200ins L =mm.The rod is welded to the furnace walland is used as a hanger for supportinginstrumentation cables. To avoid damaging the cables, the temperature of the rod at its exposed surface, o T , must be maintained below a specified operating limit of max 100T =℃. The ambient air temperature is 25T ∞=℃, and the convection coefficient is15h =2W m K ⋅. Derive an expression for the exposed surface temperature o T as afunction of the prescribed thermal and geometrical parameters. The rod has an exposed length o L , and its tip is well insulated.Problem 3.139 Consider two long, slender rods of the same diameter but different materials. One end of each rod is attached to a base surface maintained at 100℃, while the surfaces of the rods are exposed to ambient air at 30℃. By traversing the length of each rod with a thermocouple, it was observed that the temperatures of the rods were equal at the positions 0.17A x =m and 0.08B x =m, where x is measured from the base surface. If the thermal conductivity of rod A is known to be 75A k =W m K ⋅, determine the value of B k for rod B. (Two rods can be considered as L →∞.)Problem 3.141 An experimental arrangement for measuring the thermal conductivity of solid materials involves the use of two long rods that are equivalent in every respect, except that one is fabricated from a standard material of known thermal conductivity A k while the otheris fabricated from the material whose thermal conductivity B k is desired. Both rods areattached at one end to a heat source of fixed temperature b T , are exposed to a fluid oftemperature T ∞, and are instrumented with thermocouples to measure the temperature at afixed distance 1x from the heat source. If the standard material is aluminum, with180A k =W m K ⋅, and measurements reveal values of 77A T =℃ and 62B T =℃ at 1x for 100b T =℃ and 25T ∞=℃, what is the thermal conductivity B k of the test material?(Two rods can be considered as L →∞.)Problrm 3.144 An isothermal silicon chipof width 20W =mm on a side is solderedto an aluminum heat sink(180k =W m K ⋅) of equivalent width.The heat sink has a base thickness of3b L =mm and an array of rectangular fins,each of length 15f L =mm. Airflow at20T ∞=℃ is maintained throughchannels formed by the fins and a coverplate, and for a convection coefficient of100h =2W m K ⋅, a minimum finspacing of 1.8 mm is dictated bylimitations on the flow pressure drop. The solder joint has a thermal resistance of6,210t c R -''=⨯2m K W ⋅. Consider limitations for which the array has 11N = fins, in which case values of the fin thickness 0.182t =mm and pitch 1.982S =mm are obtained from the requirements that ()1W N S t =-+ and 1.8S t -=mm. If the maximum allowable chip temperature is 85c T =℃, what is corresponding value of the chip power c q ? An adiabatic fin tip condition may be assumed, and airflow along the outer surface of the heat sink may be assumed to provide a convection coefficient equivalent to that associated with airflow through the channels.。

热量传递试题及答案一、选择题（每题2分，共20分）1. 热量传递的三种基本方式是什么？A. 热传导B. 热对流C. 热辐射D. 热扩散答案：ABC2. 下列哪种物质的热传导性能最好？A. 木头B. 铜C. 空气D. 水答案：B3. 热对流主要发生在哪种介质中？A. 固体B. 液体C. 气体D. 所有介质答案：BC4. 热辐射不需要介质传播，其传播速度是多少？A. 300,000 km/sB. 1,000 km/sC. 3,000 km/sD. 10,000 km/s答案：A5. 热传导的速率与哪种因素无关？A. 温差B. 材料的导热系数C. 材料的厚度D. 材料的密度答案：D二、填空题（每题2分，共20分）1. 热量传递的三种基本方式包括热传导、热对流和______。

答案：热辐射2. 热传导的速率与物体的______成正比。

答案：温差3. 热对流的速率与流体的______有关。

答案：流速4. 热辐射的传播速度与______相同。

答案：光速5. 热传导的速率与物体的______成反比。

答案：厚度三、简答题（每题10分，共40分）1. 请简述热传导的基本原理。

答案：热传导是指热量从高温区域通过物质内部传递到低温区域的过程，其传递方式依赖于物质内部分子的振动和碰撞。

2. 热对流与热传导有何不同？答案：热对流是指热量通过流体的流动来传递，而热传导则是通过物质内部分子的振动和碰撞来传递热量。

3. 热辐射是如何传递热量的？答案：热辐射是通过电磁波的形式传递热量，不需要介质，可以在真空中传播。

4. 在日常生活中，哪些现象是热对流的例子？答案：热对流的例子包括烧开水时水的沸腾、暖气系统的热空气上升和冷空气下降、以及烹饪时食物的加热过程。

四、计算题（每题20分，共20分）1. 一块厚度为5厘米的铜板，其导热系数为400 W/(m·K)，当铜板两侧的温度差为100°C时，计算铜板的热传导速率。

答案：热传导速率Q = k * A * ΔT / d，其中 k 为导热系数，A 为面积，ΔT 为温差，d 为厚度。

2015/03/26, M3510A, Heat Transfer, Homework V , Due 2015/04/09Problem 3.67 A hollow aluminum sphere (230k =W m K ⋅), with an electrical heater in the center, is used in tests to determine the thermal conductivity of insulating materials. The inner and outer radii of the sphere are 0.18 and 0.21 m, respectively, and testing is done under steady-state conditions with the inner surface of the aluminum maintained at 250℃. In a particular test, a spherical shell of insulation is cast on the outer surface of the sphere to a thickness of 0.15 m. The system is in a room for which the air temperature is 20℃ and the convection coefficient at the outer surface of the insulation is 302W m K ⋅. If 80 W are dissipated by the heater under steady-state conditions, what is the thermal conductivity of the insulation? (The surface area of a sphere is 24r π.)Problem 3.75 A spherical shell of inner and outer radii i r and o r , respectively, is filled with aheat-generating material that provides for a uniform volumetric generation rate of q '''[3W m ]. The outer surface of the shell is exposed to a fluid having a temperature T ∞ and a convection coefficient h . Obtain an expression for the steady-state temperature distribution ()T r in the shell, expressing your result in terms of i r , o r , q ''', h , T ∞, and the thermal conductivity k of the shell material. (The volume of a sphere is 343r π.)Problem 3.81 A plane wall of thickness 0.2 m and thermal conductivity 30W m K ⋅ having uniform volumetric heat generation of 0.43MW m is insulated on one side, thile the other side is exposed to a fluid at 92℃. The convection heat transfer coefficient between the wall and the fluid is 5002W m K ⋅. Determine the maximum temperature in the wall.Problem 3.96 A cylinderical shell of inner and outer radii, i r and o r , respectively, is filled with a heat generating material that provides a uniform volumetric generation rate of q '''[3W m ]. The inner surface is insulated, while the outer surface of the shell is exposed to a fluid at T ∞ and a convection coefficient h . (a) Obtain an expression for the steady-state temperature distribution ()T r in the shell, expressing your result in terms of i r , o r , q ''', h , T ∞, and the thermal conductivity k of the shell material. (b) Determine an expression for the heat rate, ()o q r ', at the outer radius of the shell in terms of q ''' and shell dimensions.Problem 3.103 Radioactive wastes are packed in a thin-walled spherical container. The waste generate thermal energy nonuniformly according to the relation ()201o q q r r '''⎡⎤'''=-⎣⎦where q ''' is the local rate of energy generation per univolume, 0q ''' is a constant, and o r is the radius of the container. Steady-state conditions are maintained by submerging the container in a liquid that is at T ∞ and provides a uniform convection coefficient h . Determine the temperature distribution, ()T r , in the container. Express your result in terms of 0q ''', o r , T ∞, h , and the thermal conductivity k of the radioactive wastes.。

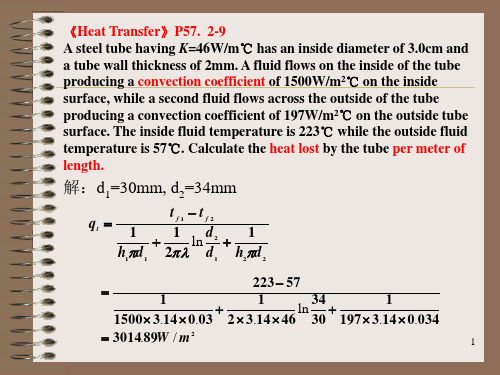
2015/03/19, M3510A, Heat Transfer, Homework IV , Due 2015/03/26Problem 3.5 A dormitory at a large university, built 50 years ago, has exterior walls constructed of 30s L =-mm-thick sheathing with a thermal conductivity of0.1s k =W m K ⋅. To reduce heat losses in the winter, the university decides to encapsulate the entire dormitory by applying an 30i L =-mm-thick layer of extruded insulation characterized by 0.029i k =W m K ⋅ to the exterior of the original sheathing. The extruded insulation is, in turn, covered with an 5g L =-mm-thick architectural glass with1.4g k =W m K ⋅. Determine the heat flux through the original and retrofitted walls when the interior and exterior air temperatures are ,22i T ∞=℃ and ,20o T ∞=-℃, respectively. The inner and outer convection heat transfer coefficients are 5i h =2W m K ⋅ and 25o h =2W m K ⋅, respectively.Problem 3.18 The composite wall of an oven consist ofthree materials, two of which are of known thermalconductivity, 25A k =W m K ⋅ and 60C k =W m K ⋅,and known thickness, 0.40A L =m and 0.20C L =m.The third material, B, which is sandwiched between materials A and C, is of known thickness, 0.20B L =m, but unknown thermal conductivity B k . Under steady-state operating conditions, measurements reveal an outer surface temperature of ,20s o T =℃, and inner surface temperature of ,600s i T =℃, and an oven temperature of 800T ∞=℃. The inside convection coefficient h is known to be 252W m K ⋅. The is the value of B k ?Problem 3.31 A commercial grade cubical freezer, 4 m on a side, has a composite wall consisting of an exterior sheet of 7.0-mm-thick plain carbon steel (64st k =W m K ⋅), an intermediate layer of 100-mm-thick cork insulation (0.039ins k =W m K ⋅), and an inner sheet of 7.0-mm-thick aluminum alloy (2024) (173al k =W m K ⋅). Adhesive interfaces between the insulation and the metallic strips are each characterized by a thermal contact resistance of 4, 2.510t c R -''=⨯2m K W ⋅. What is the steady-state cooling load that must be maintained by the refrigerator under conditions for which the outer and inner surface temperatures are 25℃ and -5℃, respectively?Problem 3.39 The diagram shows a conical section fabricatedfrom pure aluminum (236al k =W m K ⋅). It is of circular cross section having diameter 12D ax =, where 0.4a =12m . Thesmall end is located at 124x =mm and the large end at2124x =mm. The end temperature are 1600T =K and2400T =K, while the lateral surface is well insulated. (a) Derivean expression for the temperature distribution ()T x insymbolic form, assuming one-dimensional conditions. (b) Calculate the heat rate x q .Problem 3.47 To maximize production and minimize pumping costs, crude oil is heated to reduce its viscosity during transportation from a production field. (a) consider a pipe-in-pipe configuration consisting of concentric steel tubes with an intervening insulating material. The inner tube is used to transport warm crude oil through cold ocean water. The inner steel pipe (35s k =W m K ⋅) has an inside diameter of ,1150i D =mm and wall thickness 10i t =mm while the outer steel pipe has an inside diameter of ,2250i D =mm and wall thickness o i t t =. Determine the maximum allowable crude oil temperature to ensure the polyurethane foam insulation (0.075p k =W m K ⋅) between the two pipes does not exceed its maximum service temperature of ,max 70p T =℃. The ocean water is at ,5o T ∞=-℃ and provides an external convection heat transfer coefficient of 500o h =2W m K ⋅. The convection coefficient associated with the flowing crude oil is 450i h =2W m K ⋅. (b) It is proposed to enhance the performance of the pipe-in-pipe device by replacing a thin (5a t =mm) section of polyurethane located at the outside of the inner pipe with an aerogel insulation material (0.012a k =W m K ⋅). Determine the maximum allowable crude oil temperature to ensure maximum polyurethane temperatures are below ,max 70p T =℃.Problem 3.50 A stainless steel (AISI 304, 14.2st k =W m K ⋅) tube used to transport a chilled pharmaceutical has an inner diameter of 40 mm and a wall thickness of 2 mm. The pharmaceutical and ambient air are at temperatures of 6℃ and 23℃, respectively, while the corresponding inner and outer convection coefficients are 4002W m K ⋅ and 62W m K ⋅, respectively. (a) What is the heat gain per unit tube length? (b) What is the heat gain per unit length if a 10-mm-thick layer of calcium silicate insulation (0.050ins k =W m K ⋅) is applied to the tube?。

热信号的传输和传热的应用练习题热信号的传输和传热是热力学领域中的重要概念，涉及到能量的传递和转化。

在本篇文章中，我们将通过一些练习题来探讨热信号的传输和传热的应用。

请仔细阅读每个问题，并尝试解答。

问题一：热信号的传输假设有一个金属杆，一端加热源温度为100°C，另一端的温度为25°C，金属杆的长度为1米。

热传导系数为0.5 W/(m·K)。

请计算以下内容：1. 金属杆中心位置的温度是多少？2. 热流密度在金属杆中心位置是多少？问题二：传热的应用考虑一个房间内的暖气系统。

暖气片表面的温度为80°C，室内温度为20°C。

请回答以下问题：1. 暖气片表面到室内温度的热流密度是多少？2. 如果暖气片的表面积为2平方米，暖气片每小时释放的热量是多少？问题三：热信号的传输与电信号的传输请简要比较热信号的传输与电信号的传输的异同点。

列举至少3个方面的异同。

问题四：传热的应用——太阳能热水器太阳能热水器是一种利用太阳能将水进行加热的设备。

请回答以下问题：1. 太阳能热水器的工作原理是什么？2. 太阳能热水器中光能如何转化为热能？3. 太阳能热水器相比传统热水器有哪些优势？问题五：传热的应用——热交换器热交换器是常用的传热设备，用于在流体之间传递热能。

请回答以下问题：1. 热交换器的基本原理是什么？2. 请以一个具体的例子说明热交换器的工作过程。

问题六：热信号的传输与声信号的传输请简要比较热信号的传输与声信号的传输的异同点。

列举至少3个方面的异同。

问题七：热信号的传输与光信号的传输请简要比较热信号的传输与光信号的传输的异同点。

列举至少3个方面的异同。

问题八：传热的应用——冷却系统冷却系统在工业生产中扮演着重要角色，用于控制设备或工艺的温度。

请回答以下问题：1. 冷却系统的基本原理是什么？2. 请以一个具体的例子说明冷却系统的应用。

问题九：热信号的传输与信息传输请简要比较热信号的传输与信息传输的异同点。

3-1气流温度按简谐波变化时，热电偶的温度响应为()*cos B θωτϕ=+(1.1)其中()arctan r A B ϕωτ==-按题目要求221/1/2010s s Tπππω===,3890039011028.925620r cV s hAρτ-⨯⨯⨯===⨯,()220/h W m K = ，根据题目提供的热电偶测量的最高、最低温度，求出热电偶测量的温度变化的振幅如下式13012432A -== (1.2)把r ωτ、的数据代入（1.2）中得气流温度变化的振幅27.4f A =，所以真实气体温度变化的最大、最小值为 m ax 13012427.4154.42t +=+=(1.3)m in 13012427.499.62t +=-=(1.4)3-21）该导热问题的数学描述为（设w t t θ=-，00w t t θ=-）22000a x x xx θθττθθθδθ⎧∂∂=⎪∂∂⎪==⎪⎨∂⎪==⎪∂⎪==⎩ (1.5)2）用分离变量法求解平壁中温度场设()()(),x X x T θττ=则式（1.5）中的导热微分方程式可写为'2"1X T Xa Tε==-(1.6)解()T τ的方程得()2a T Ceεττ-= (1.7)解()X x 的方程得()()()cos sin X x A x B x εε=+(1.8)把关于x 的边界条件代入（1.8）式得0B =，2n n ππεδ+=（n=0，1，2…）()220exp 22,cos n n x a A n n x ππτππδδθτ∞=⎡⎤⎡⎤⎛⎫⎛⎫+-+⎢⎥⎪ ⎪⎢⎥⎝⎭⎝⎭⎣⎦⎢⎥⎣⎦=∑(1.9)把初始条件代入式（1.9）得002cos n n x A n ππδθ∞=⎡⎤⎛⎫+ ⎪⎢⎥⎝⎭⎣⎦=∑(1.10)解得()()()()0002022cos 4121cos nn x n dx A x n n dx δδππθπθδππδ⎡⎤+⎢⎥-⎣⎦==+⎡⎤+⎢⎥⎣⎦⎰⎰(1.11)把（1.11）代入（1.9）得()()()2020exp 2241,cos 21nn xa n n x n ππτππδδθτθπ∞=⎡⎤⎡⎤⎛⎫⎛⎫+-+⎢⎥⎪⎪⎢⎥⎝⎭⎝⎭⎣⎦⎢⎥⎣⎦-=+∑(1.12) 3）用拉普拉斯变换法求该问题适用于短时间的解设0t t θ=-则拉式变换后的导热问题数学描述为_2_2__00w s d a dx d x dx x sθθθθδθ⎧⎪=⎪⎪⎪==⎨⎪⎪==⎪⎪⎩(1.13)解得_chxθ=(1.14)整理上式可得_expexp x xθ+=(1.15)())()_exp exp exp x x δθ⎡⎤+=(1.16)())()()()()_expexp 1exp 2nw n x x s θθδδδ∞=⎡⎤=-++--⎣⎦∑(1.17)()()()()(){}_1exp 21exp 21nwn n x n x sθθδδ∞=⎡⎤⎡⎤=-++++-⎣⎦⎣⎦∑(1.18)短时间的解()()()(()()({}_01e 21e 21nw n rfc n x rfc n x θθδδ∞=⎡⎤⎡⎤=-++++-⎣⎦⎣⎦∑ (1.19)3-3该导热问题的数学描述为，设0t t θ=-22000a xx qxx θθττθθλδθ⎧∂∂=⎪∂∂⎪==⎪⎨∂⎪=-=⎪∂⎪==⎩ (1.20)解上述导热问题设()2qx θθδλ=--，首先求解()2,x θτ()2222222000a xqx x xx θθττθδλθδθ⎧∂∂=⎪∂∂⎪⎪==-⎪⎨⎪∂==⎪∂⎪⎪==⎩ (1.21)与题3-2相同，解上式可得()220exp 22,cos n n x a A n n x ππτππδδθτ∞=⎡⎤⎡⎤⎛⎫⎛⎫+-+⎢⎥⎪ ⎪⎢⎥⎝⎭⎝⎭⎣⎦⎢⎥⎣⎦=∑(1.22)把初始条件代入可得 ()02cos n n qx x A n πδπλδ∞=⎡⎤⎛⎫-+ ⎪⎢⎥⎝⎭⎣⎦-=∑(1.23)解得()()()142112121n n q x A n n δλπδπ-⎡⎤⎛⎫=--+⎢⎥ ⎪++⎝⎭⎣⎦ (1.24)()2qx θθδλ=--，把（1.24）代入（1.22）得平壁中温度场()()()221041exp 2122211cos 21n n qq x a x n n n x n δππτδππλλπδδθδπ∞-=⎡⎤⎡⎤⎡⎤⎛⎫⎛⎫⎛⎫-++-+⎢⎥⎢⎥⎪ ⎪ ⎪⎢⎥+⎝⎭⎝⎭⎝⎭⎣⎦⎢⎥⎢⎥⎣⎦⎣⎦=--++∑(1.25)3-4解：该问题的数学描述可表示为：22t t axτ∂∂=∂∂ 0x δ≤≤ 0τ>0t t = 0x δ≤≤ 0τ= 0t t q Cxλτ∂∂-=-∂∂ x δ= 0τ>0t t = 0x = 0τ>将上述数学描述无量纲化可得：22FoXθθ∂∂=∂∂ 01X ≤≤ 0F o >0θ= 01X ≤≤ 0F o =M KXF oθθ∂∂=-∂∂ 1X = 0F o >0θ= 0X = 0F o >其中：2a F o τδ=xX δ=CCaK cδρλδ==0q M δλ=对无量纲化的方程及边界条件做拉普拉斯变换，得220d s dxθθ-= 01X ≤≤ 0s >d M K s dxsθθ=- 1X = 0s >0θ= 0X = 0s >解此方程可得：M shθ⋅=对θ做拉普拉斯反变换可得出原函数θ。

中考物理热能的传递与转换历年真题及答案解读热能的传递与转换是物理学中的一个重要概念。

在中考物理考试中，对于这个知识点经常会涉及到一些历年真题。

本文将通过解读几道历年中考物理真题的答案，来帮助同学们更好地理解热能的传递与转换。

1. 真题解读首先，我们来看一道典型的物理真题：题目：一个物体在具有热导性的材料中传热，下列说法正确的是（）A.只能通过热辐射传热B.只能通过热对流传热C.只能通过热传导传热D.能够通过热辐射、热对流和热传导传热正确答案：D解析：根据热能的传递方式，热能的传递主要有三种方式，即热传导、热对流和热辐射。

所以正确答案是D，能够通过热辐射、热对流和热传导传热。

2. 真题解析接下来，我们来解析另外一道中考物理真题：题目：用一定量的水和一定量的冰块混合，保持系统密闭，观察水温的变化过程，下列说法正确的是（）A.水温上升，冰块完全融化B.水温下降，冰块完全融化C.水温上升，冰块部分融化D.水温下降，冰块部分融化正确答案：C解析：在这个实验中，水和冰块混合后的系统会发生热交换。

由于冰块的温度低于水的温度，所以水会向冰块传递热能，冰块会融化。

但由于冰块的质量有限，无法吸收全部的热能，所以只有部分冰块会融化，同时水的温度也会上升。

因此，正确答案是C，水温上升，冰块部分融化。

3. 真题应用最后，我们再来应用一道中考物理真题：题目：在屋里带手电筒在半空操作时，某次手电筒不慎掉在了木地板上，此时，手电筒内电池的化学能转化成了（）A. 动能B. 振动能C. 热能D. 光能正确答案：C解析：当手电筒掉在地板上时，由于物体会发生碰撞，这个过程中会产生能量转化。

手电筒内的电池化学能转化成了热能，因为碰撞会造成摩擦和微小的振动，从而将电池的化学能转化为热能。

因此，正确答案是C，电池的化学能转化为热能。

通过对以上三道中考物理真题的解析，我们可以看到热能的传递与转换在物理学中的重要性。

在物理学中，热能是一种能量形式，它可以通过热传导、热对流和热辐射进行传递。