SAT数学经典试题解析

2023年SAT数学真题解析（正文）2023年的SAT数学真题提供了对学生数学能力的综合测试。

本文将对这套题目进行解析，帮助学生更好地理解题意并提供解题的方法和思路。

在解析的过程中，将全部使用中文进行说明，以确保语句通顺，表达流畅，同时保障文章整洁美观。

（开篇）2023年的SAT数学真题共有多道题目，涵盖了各个数学领域的内容。

以下将对其中几道题目进行详细解析，帮助学生更好地理解数学概念并掌握解题技巧。

（主体）题目一：题目描述：在一个正三角形ABC中，角A的大小为60度。

求三角形ABC内角A的余弦值。

解析：首先，我们需要知道正三角形的定义，即三个内角都为60度的三角形。

由于题目已经给出角A的大小为60度，我们仅需计算角A的余弦值。

余弦是三角函数中的一个重要概念，定义为一个角的邻边与斜边的比值。

根据题目描述，我们可以知道正三角形的三个内角都为60度，因此，角A的余弦值等于角A的邻边与斜边的比值。

在正三角形ABC中，斜边为边AB，邻边为边BC。

根据几何关系，边BC的长度等于边AB长度的一半。

因此，角A的余弦值等于1/2。

综上所述，题目中角A的余弦值为1/2。

题目二：题目描述：一个圆的半径为3 cm，求该圆的面积和周长的比值。

解析：题目已经给出了圆的半径为3 cm，我们需要计算面积和周长的比值。

圆的面积公式为πr²，其中π为圆周率，r为圆的半径。

圆的周长公式为2πr。

根据题目中给出的半径为3 cm，我们可以代入公式来计算。

首先，计算圆的面积：面积= π × (3 cm)² = 9π cm²。

然后，计算圆的周长：周长= 2π × 3 cm = 6π cm。

最后，求面积和周长的比值：比值 = 面积 / 周长 = (9π cm²) / (6π cm) = 3/2。

综上所述，题目中圆的面积和周长的比值为3/2。

题目三：题目描述：已知直角三角形ABC中，角A为90度，角B的正弦值为1/2。

银川海派英语【 SAT 数学】练习题含详细答案解析下面是 5 道 SAT 数学练习题，每道题目后面都有答案和详细的解析，希望同学们在练习的时候先不看答案，看看自己的能做对几道题，然后再与答案对照，找出错题原因，针对自己的SAT 数学复习进行查漏补缺。

1.If a2 = 12 then a4 =A. 144B. 72C. 36D. 24E. 16Correct Answer: A解析 :a4 = a2 x a2 = 12 x 12 = 1442. If n is even which of the following cannot be odd?I n + 3II 3nIII n2 - 1A. I onlyB. II onlyC. III onlyD. I and II onlyE.I II and III CorrectAnswer: B解析 :In case I even plus odd will give odd. In case II odd times even will give even. In case III even squared is even and even minus odd is odd. (You can check this by using an easy even number like2 in place of n). Only case II cannot be odd.3.One side of a triangle has length 8 and a second side has length 5. Which of the following could be the area of the triangle?I24II 20III 5A. I onlyB. II onlyC. III onlyD. II and III onlyE. I II and IIICorrect Answer: D解析 :The maximum area of the triangle will come when the given sides are placed at right angles. If we take 8 as the baxxxxse and 5 as the height the area = ? x 8 x 5 = 20. We can alter the angle between the sides to make it less or more than 90 but this will only reduce the area. (Draw it out for yourself). Hence the area can be anything less than or equal to 20.4.A certain animal in the zoo has consumed 39 pounds of food in six days. If it continues toeat at the same rate in how many more days will its total consumption be 91 pounds?A. 12B. 11C. 10D.9E.8Correct Answer: E解析 :Food consumed per day = 39/6. In the remaining days it will consume 91 - 39 pounds = 52 pounds. Now divide the food by the daily consumption to find the number of days. 52 / (39/6) = 52x (6/39)=85. A perfect cube is an integer whose cube root is an integer. For example 27 64 and 125are perfect cubes. If p and q are perfect cubes which of the following will not necessarily be a perfect cube?A.8pB.pqC.pq + 27D.-pE.(p - q)6 CorrectAnswer: C解析 :A perfect cube will have prime factors that are in groups of 3; for example 125 has the prime factors 5 x 5 x 5 and 64 x 125 will also be a cube because its factors will be 4 x 4 x 4 x 5 x 5 x 5. Consider the answer choices in turn. 8 is the cube of 2 and p is a cube and so the product will also be a cube. pq will also be a cube as shown above.pq is a cube and so is 27 but their sum need not be a cube. Consider the case where p =1 and q = 8 the sum of pq and 27 will be 35 which has factors 5 x 7 and is not a cube. -p will be a cube. Since the difference between p and q is raised to the power of 6 this exxxxxpression will be a cube (with cube root = difference squared).要想 SAT 数学成绩有所提高，坚持每天做练习是十分必要的，就像这样每天完成 5 道SAT 数学题目，并进行总结，日积月累，成绩就会有明显地提高。

SAT考试2024数学历年题目全解SAT考试是一项全球性的标准化考试，旨在评估学生在阅读、写作和数学方面的能力。

数学部分是SAT考试的一个重要组成部分，涵盖了各种数学概念和技巧。

本文将为您提供2024年SAT数学部分的历年题目全解，帮助您更好地应对这一考试。

第一题：题目：求解以下方程：3x + 5 = 20解析：要求解方程3x + 5 = 20，我们首先将5从等式两边减去，得到3x = 15。

然后，我们将方程两边都除以3，即x = 5。

因此，方程的解为x = 5。

第二题：题目：计算以下比例的值：5:8 = x:40解析：要计算比例5:8与x:40的值，我们可以采取交叉乘法的方法。

将5乘以40，并将结果除以8，即可求得x的值。

计算过程如下：5 * 40 / 8 = 200 / 8 = 25因此，比例5:8与x:40的值为25。

第三题：题目：已知一个等边三角形的边长为12，计算其面积。

解析：一个等边三角形的边长为12，则其高可以通过勾股定理求得。

根据勾股定理，我们有：高的平方= 边长的平方- 底边的一半的平方。

设高为h，则有 h^2 = 12^2 - (12/2)^2= 144 - 36= 108因此，高h = √108 = 6√3由于等边三角形的高等于边长的一半乘以根号3，所以面积S可以计算为：S = 1/2 * 12 * 6√3= 6 * 6√3= 36√3因此，该等边三角形的面积为36√3。

第四题：题目：在一个长方形花坛中，长度是宽度的3倍，已知宽度为2米，计算花坛的面积。

解析：我们知道长方形花坛的面积可以通过长度乘以宽度来计算。

已知宽度为2米，则长度为3 * 2 = 6米。

因此，花坛的面积为2 * 6 =12平方米。

通过以上题目的解析，我们可以看到SAT数学部分考察了各种数学概念和技巧，包括方程的求解、比例的计算、勾股定理的应用以及长方形面积的计算等。

熟练掌握这些数学知识，并能够灵活运用于实际问题的解决中，将有助于您在SAT考试中取得更好的成绩。

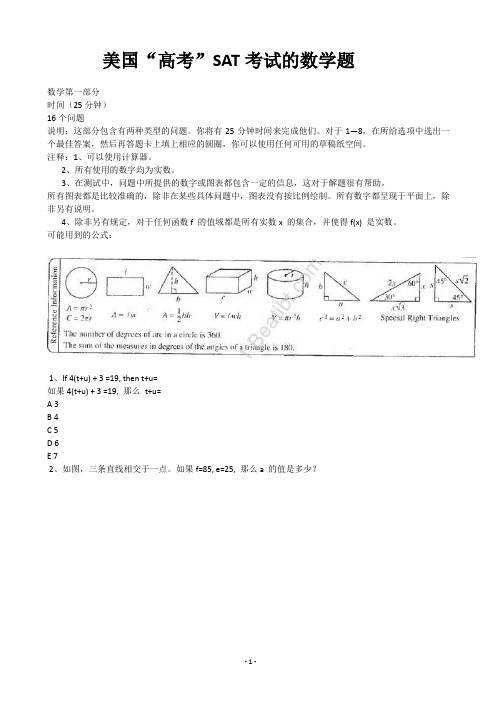
美国“高考”SAT考试的数学题数学第一部分时间（25分钟）16个问题说明：这部分包含有两种类型的问题。

你将有25分钟时间来完成他们。

对于1—8，在所给选项中选出一个最佳答案，然后再答题卡上填上相应的圆圈，你可以使用任何可用的草稿纸空间。

注释：1、可以使用计算器。

2、所有使用的数字均为实数。

3、在测试中，问题中所提供的数字或图表都包含一定的信息，这对于解题很有帮助。

所有图表都是比较准确的，除非在某些具体问题中，图表没有按比例绘制。

所有数字都呈现于平面上，除非另有说明。

4、除非另有规定，对于任何函数f 的值域都是所有实数x 的集合，并使得f(x) 是实数。

可能用到的公式：1、If 4(t+u) + 3 =19, then t+u=如果4(t+u) + 3 =19, 那么t+u=A 3B 4C 5D 6E 72、如图，三条直线相交于一点。

如果f=85, e=25, 那么a 的值是多少？A 60B 65C 70D 75E 853、如果玛丽开车行驶n 英里用了t 小时，那么下列哪个可以表示她行驶的平均速度，英里/小时？A n/tB t/nC 1/ntD ntE n²t4、如果a 是一个奇数，b 是一个偶数，那么选项中哪一个是奇数？A 3bB a+3C 2(a+b)D a+2bE 2a+b5、在平面坐标内，F(-2,1),G(1,4), H(4,1)在以P为圆心的圆上，那么点P的坐标是什么？A（0,0）B（1,1）C（1,2）D（1，-2）E（2.5,2.5）6、如图，如果-3≤x≤6，那么x 有几个值，使得f(x)=2?A 零B 一个C 两个D 三个E 三个以上7、如果t 和t+2 的算术平均值是x, t 和t-2的算术平均值是y，那么x 和y 的算术平均值是多少？A 1B 1/2C tD t+1/2E 2t8、对于任何数x 和y,假设x△y=x²+xy+y²,那么（3△1）△1等于多少？A 5B 13C 27D 170E 1839、摩根的植物在一年之内从42厘米长到57厘米。

SAT数学真题解析SAT数学部分是考试中最具挑战性的部分之一，要求考生具备扎实的数学基础和解题能力。

本文将对SAT数学真题进行详细解析，帮助考生更好地应对这一部分的考试。

一、概述SAT数学部分涵盖的知识点包括代数、几何、数据分析和数学问题解决等。

题型主要包括选择题和解答题，其中选择题又分为单选和多选题。

考生在备考过程中，除了要系统学习相关知识点，还要注重解题技巧和策略的培养。

二、代数题解析代数是SAT数学部分的重点考察内容之一，下面将以一道典型的代数题为例进行解析。

Example 1:If 2x + 5 = 13, what is the value of x?解析：根据题目中的等式，我们可以求解出变量x的值。

首先，将等式中的5移到等号的另一边，得到2x = 13 - 5，即2x = 8。

接下来，我们将等式两边都除以2，得到x = 8 / 2，即x = 4。

所以，x的值为4。

三、几何题解析几何是SAT数学部分另一个重要的考察内容，下面以一道几何题进行解析。

Example 2:In triangle ABC, angle BAC = 60°, and BC = AB. What is the measureof angle BCA?解析：根据题目中给出的信息，我们需要求解角BCA的度数。

根据三角形内角和定理，三角形ABC的三个内角之和等于180度，即角BAC + 角ABC + 角BCA = 180°。

已知角BAC = 60°，并且BC = AB。

由于BC = AB，我们可以得出角ABC = 角ACB。

将以上信息代入角和的等式中，得到60° + 2角ABC = 180°。

进一步化简，得到2角ABC = 120°。

再继续化简，得到角ABC = 60°。

因为角ABC = 角ACB，所以角BCA的度数也为60°。

四、数据分析题解析数据分析是SAT数学部分的重要题型之一，要求考生对给定的数据进行分析和解读。

sat试题及答案解析SAT试题及答案解析1. 阅读下列句子，选择最恰当的词汇填入空白处。

句子：The artist's new painting was a _______ of colors that left the audience in awe.选项：A. explosionB. collectionC. mixtureD. gathering答案：A解析：在这个句子中，"explosion"（爆炸）一词用来形容色彩的强烈和丰富，给人以强烈的视觉冲击，因此是最合适的词汇。

2. 阅读以下段落，回答以下问题。

段落：In the early morning, the sun rose slowly over the horizon, casting a golden glow on the sleepy town. The streets were still quiet, with only a few people walking by.问题：What time of day is described in the passage?答案：Early morning解析：文中提到“the sun rose slowly over the horizon”和“streets were still quiet”，这些描述都暗示了时间是清晨。

3. 完成以下数学题。

题目：If a car travels 120 miles in 3 hours, what is its speed in miles per hour?答案：40 mph解析：速度的计算公式是距离除以时间。

因此，120英里除以3小时等于40英里每小时。

4. 阅读下列句子，判断下列陈述是否正确。

句子：The scientist's hypothesis was proven incorrectafter the experiment.陈述：The experiment confirmed the scientist's hypothesis.答案：错误解析：句子中提到“hypothesis was proven incorrect”，意味着实验结果与科学家的假设相反，因此陈述是错误的。

2024年SAT考试数学真题深度解读在2024年的SAT考试数学部分中，出现了一系列挑战性的问题，涵盖了几个重要的数学概念和技巧。

本文将对这些问题进行深度解读，帮助同学们更好地理解题目，并为他们提供解题思路和解题技巧。

问题1：在一个三角形ABC中，角A的度数是50。

已知边AB与BC的长度分别为5和8，求边AC的长度。

解析：首先，我们可以利用三角形的角度之和为180度的性质，求得角B为130度。

然后，我们可以使用余弦定理来求解边AC的长度。

根据余弦定理，我们有：AC^2 = AB^2 + BC^2 - 2 * AB * BC * cosA代入已知值，我们可以得到：AC^2 = 5^2 + 8^2 - 2 * 5 * 8 * cos50通过计算，我们可以得到AC的长度为约9.18。

问题2：已知函数f(x) = 2x^3 + 3x^2 - 4x + 1，求f'(2)的值。

解析：我们需要求函数f(x)在x = 2处的导数值，即f'(2)。

首先，我们对函数f(x)进行求导，得到f'(x) = 6x^2 + 6x - 4。

然后，将x = 2代入f'(x)中，我们可以计算得到f'(2)的值为28。

问题3：已知一个等差数列的第一个项为a，公差为d。

如果这个数列的第100项是200，求a和d的值。

解析：由于等差数列的通项公式为an = a + (n - 1)d，我们可以得到第100项的表达式a + 99d = 200。

而且，我们还知道该数列的第1项即为a，因此可以得到第1项的表达式a + 0d = a。

由题意可知，第100项与第1项的差值为99d，即200 - a = 99d。

将这两个方程组合起来，我们可以得到一个二元一次方程组：a + 99d = 200200 - a = 99d通过求解这个方程组，我们可以得到a的值为101，d的值为1。

问题4：某公司的销售额在过去的几年呈现如下的增长趋势：2019年为100万，2020年为120万，2021年为140万。

SAT考试2024数学历年题目精讲在本篇文章中，我们将重点讲解SAT考试2024年数学部分的历年题目。

我们将按照题目类型进行分类，并为每个题型提供详细的解答和解题技巧，帮助考生更好地应对这些题目。

一、单选题1. 题目描述：某汽车展厅共展出了150辆汽车，其中的三分之一是SUV车型，四分之一是轿车车型，其余的是其他车型。

问展厅中轿车车型的数量是多少？解答与技巧：首先，计算出SUV车型的数量：150 * (1/3) = 50辆。

然后，计算出其他车型的数量：150 - 50 - 150 * (1/4) = 50辆。

所以，轿车车型的数量是50辆。

2. 题目描述：某商场举办了一次打折活动，原价100元的商品现在只需80元购买。

如果小明购买了3件该商品，他需要支付多少钱？解答与技巧：首先，计算出每件商品的折扣金额：100 - 80 = 20元。

然后，计算出小明需要支付的金额：3 * 20 = 60元。

所以，小明需要支付60元。

二、多选题1. 题目描述：以下哪些数是正整数？（A）-1（B）0（C）1（D）2解答与技巧：在SAT考试中，如果题目要求选择多个选项，我们需要仔细审题。

在这个题目中，需要选择正整数，所以选项B和A都不是正整数。

所以正确答案是（C）和（D）。

2. 题目描述：以下哪些图形具有对称性？（A）正方形（B）长方形（C）圆形（D）三角形解答与技巧：我们需要判断每个选项是否具有对称性。

在这个题目中，正方形和圆形都具有对称轴，所以正确答案是（A）和（C）。

三、填空题1. 题目描述：若a + a^-1 = 5，求a^2 + a^-2的值。

解答与技巧：首先，我们可以对等式两边进行平方操作，得到a^2+ 2 + a^(-2) = 25。

然后，我们需要解方程，将等式左边与右边的常数项进行抵消，得到a^2 + a^(-2) = 23。

2. 题目描述：某比赛共有10个选手参加，其中3个选手退出比赛，剩余的选手中将决出第一名、第二名和第三名。

sat数学试题及答案SAT数学试题及答案一、选择题1. 一个圆的半径是5，求这个圆的面积。

A. 25πB. 50πC. 75πD. 100π答案：B2. 如果一个数列的前三项是2, 4, 6，那么第10项是多少？A. 18B. 20C. 22D. 24答案：B3. 一个三角形的三边长分别为3, 4, 5，这个三角形是：A. 等边三角形B. 等腰三角形C. 直角三角形D. 钝角三角形答案：C二、填空题4. 一个数的平方根等于它本身，这个数是________。

答案：0或15. 如果一个函数f(x) = 3x + 5，求f(-2)的值。

答案：-16. 一个长方形的长是10厘米，宽是5厘米，求它的周长。

答案：30厘米三、简答题7. 一个圆的周长是31.4厘米，求这个圆的直径。

解：根据圆的周长公式C = πd，我们有31.4 = πd。

解得d = 31.4 / π ≈ 10厘米。

8. 一个等差数列的首项是5，公差是3，求第20项的值。

解：等差数列的通项公式为an = a1 + (n - 1)d。

将首项a1 = 5和公差d = 3代入公式，得到a20 = 5 + (20 - 1) * 3 = 5 + 57 = 62。

9. 一个直角三角形的两条直角边分别是6和8，求斜边的长度。

解：根据勾股定理，斜边c的长度等于两直角边的平方和的平方根，即c = √(6² + 8²) = √(36 + 64) = √100 = 10。

四、解答题10. 一个工厂生产了1000个零件，其中5%是次品。

如果工厂决定只出售合格的零件，那么工厂将出售多少个零件？解：首先计算次品的数量，1000 * 5% = 50个。

然后从总数中减去次品的数量，得到出售的合格零件数量：1000 - 50 = 950个。

11. 一个投资项目预计在第一年结束时产生$10,000的利润，如果每年的增长率为5%，那么第三年结束时的利润是多少？解：使用复合利息公式计算，P = P0 * (1 + r)^n，其中P0是初始利润，r是增长率，n是年数。

that satisfy the second equation arethat satisfies both equations. That value isThe formula for a cone ‘s surface area is πr2 + πrl. A cone ‘s volume is 1/ 3 πr2h. So if一道SAT 数学题(抛物线)The correct answer is CExplanationmust be the vertex of the parabola. The parabola intersects the , so must be halfway. Of the choices, the only one withcould be the coordinates ofVertical height of triangle = 5 ; horizontal side = 4 ; hypotenuse = 2. n is an integer chosen at random from the set{5, 7, 9, 11 }p is chosen at random from the set{2, 6, 10, 14, 18}A. 0.75DB. 0.76DC. 0.765DD. 0.775Dwhat is the best approximation to the length of arc AB ?A. 9π. The length of the arc is 100/360 of themembers and the chess club hasstudents belong to only one of the two clubs, how manystudents belong to both clubs?Answer ChoicesThe correct answer is CExplanationstand for the number of students who belong to both clubs. Thestudents who are in the chess club only.that. Solving this equation gives belong to both clubs 。

SAT考试专题2024数学历年题目解析2024年的SAT考试将继续囊括数学科目，下面将对该年度的数学部分历年题目进行解析，帮助考生更好地准备SAT数学考试。

1. 第一题解析该题目是一道代数题，要求求解方程：3x + 5 = 20。

解题思路：将方程中的变量与常数项分离，得到：3x = 20 - 5。

计算得：3x = 15，再将等式两边同时除以3，得到：x = 5。

因此，方程的解为x = 5。

2. 第二题解析该题目是一道几何题，要求计算三角形的面积。

解题思路：已知三角形的底边长度为6，高为8。

直接使用三角形面积公式：面积 = 底边长度 ×高 ÷ 2。

代入已知的数值进行计算：面积 = 6 × 8 ÷ 2 = 24。

因此，该三角形的面积为24平方单位。

3. 第三题解析该题目是一道概率题，要求计算从一副标准扑克牌中随机抽取一张牌，该牌为红桃的概率。

解题思路：一副标准扑克牌中共有52张牌，其中有13张红桃牌。

因此，红桃牌的概率为：概率 = 红桃牌数目 ÷总牌数目。

代入已知数值进行计算：概率 = 13 ÷ 52 = 1 ÷ 4 = 0.25。

因此，从一副标准扑克牌中随机抽取一张牌，该牌为红桃的概率为0.25。

4. 第四题解析该题目是一道函数题，要求计算函数的值。

解题思路：已知函数 f(x) = 2x^2 + 3x + 1，需要计算当 x = 2 时的函数值。

将 x = 2 代入函数表达式中，得到：f(2) = 2 × 2^2 + 3 × 2 + 1。

计算得：f(2) = 8 + 6 + 1 = 15。

因此，当 x = 2 时，函数 f(x) 的值为15。

5. 第五题解析该题目是一道统计题，要求根据给定的数据计算平均数。

解题思路：已知一组数据为：10, 12, 15, 18, 20。

需要计算这组数据的平均数。

平均数的计算公式为：平均数 = 总和 ÷数据个数。

SAT数学试题及答案本文收集了SAT数学部分的一些题目及其答案，旨在帮助考生更好地备考。

选择题1. 如果$0 \leq x \leq 3$，则不等式$|x-2| \leq 1$的解集为A) $0 \leq x \leq 3$B) $1 \leq x \leq 3$C) $1 \leq x \leq 4$D) $0 \leq x \leq 4$答案：B解析：不等式 $|x-2| \leq 1$ 表示 $x$ 到 $2$ 的距离小于等于$1$。

当 $x$ 在区间 $[1,3]$ 时，$x$ 到 $2$ 的距离都不超过 $1$，因此解集为 $1 \leq x \leq 3$。

2. 抛物线 $y=x^2-2x-3$ 的顶点坐标为A) $(0,-3)$B) $(1,-4)$C) $(2,-3)$D) $(3,0)$答案：C解析：抛物线的顶点坐标为$(\frac{-b}{2a},c-\frac{b^2}{4a})$，其中 $a$、$b$、$c$ 分别是二次项系数、一次项系数和常数项。

将$y=x^2-2x-3$ 化为标准形式，即 $y=(x-1)^2-4$，可知该抛物线的顶点坐标为 $(1,-4)$。

填空题3. 矩阵 $\begin{matrix}3 & 2 \\1 & 4\end{matrix}$ 的逆矩阵是$$\begin{pmatrix}\text{______} & \text{______} \\ \text{______} & \text{______} \end{pmatrix}$$**答案：$\begin{pmatrix}\frac{4}{5} & -\frac{2}{5} \\-\frac{1}{5} & \frac{3}{5}\end{pmatrix}$**解析：设 $\begin{pmatrix}a &b \\c & d\end{pmatrix}$ 表示该矩阵的逆矩阵，则有 $ \begin{pmatrix}3 & 2 \\1 & 4\end{pmatrix}\begin{pmatrix}a &b \\c & d\end{pmatrix}= \begin{pmatrix}1 & 0 \\0 & 1\end{pmatrix}，$ 即 $\begin{cases}3a+2c=1, \\3b+2d=0, \\a+4c=0, \\b+4d=1. \\\end{cases}$ 解得逆矩阵为 $\begin{pmatrix}\frac{4}{5} & -\frac{2}{5} \\-\frac{1}{5} & \frac{3}{5}\end{pmatrix}$。

sat数学考试试题(可编辑修改版) SAT数学考试试题(可编辑修改版)在这份SAT数学考试试题中，我们为您精心挑选了一些题目，希望能够帮助您进行备考和巩固数学知识。

请您认真阅读题目，并尽量独立思考和解答。

每道题后面都附带了详细的解答和解题思路，以供参考。

祝您考试顺利！题目一：若a和b都是正整数，且a/b = 1/4，那么a+b的值是多少？A. 5B. 8C. 14D. 16E. 20解答一：我们可以通过代入法求解这道题。

假设a=1，b=4，则a/b=1/4。

因此，a+b=1+4=5。

所以，选项A是正确答案。

题目二：某公司的年度收入增长率为20%，每年的增长幅度相同。

如果这家公司在2018年的年度收入为100万美元，那么在2021年的年度收入是多少？A. 120万美元B. 160万美元C. 140万美元D. 180万美元E. 200万美元解答二：我们可以使用复合增长率的方法来解决这道题。

首先，我们假设2018年的年度收入为x万美元。

根据题目的信息，可得以下等式：x * (1 + 0.2) * (1 + 0.2) * (1 + 0.2) = 100解方程可得：(1.2)^3 * x = 1001.728x = 100x ≈ 57.87（万美元）因此，2021年的年度收入约为57.87 * (1 + 0.2) * (1 + 0.2) * (1 + 0.2) ≈ 140（万美元）。

所以，选项C是正确答案。

题目三：在直角三角形ABC中，角A是直角，边AC = 10，边BC = 24。

点D位于边BC上，使得边AD垂直于边BC。

求边AD的长度是多少？A. 7B. 8C. 12D. 15E. 20解答三：由题目可知，三角形ABC是一个直角三角形。

根据勾股定理，可得：AC^2 + BC^2 = AB^210^2 + 24^2 = AB^2100 + 576 = AB^2676 = AB^2因此，AB = √676 = 26。

美国“高考”SAT考试的数学题数学第一部分时间（25分钟）16个问题说明：这部分包含有两种类型的问题。

你将有25分钟时间来完成他们。

对于1—8，在所给选项中选出一个最佳答案，然后再答题卡上填上相应的圆圈，你可以使用任何可用的草稿纸空间。

注释：1、可以使用计算器。

2、所有使用的数字均为实数。

3、在测试中，问题中所提供的数字或图表都包含一定的信息，这对于解题很有帮助。

所有图表都是比较准确的，除非在某些具体问题中，图表没有按比例绘制。

所有数字都呈现于平面上，除非另有说明。

4、除非另有规定，对于任何函数f 的值域都是所有实数x 的集合，并使得f(x) 是实数。

可能用到的公式：1、If 4(t+u) + 3 =19, then t+u=如果4(t+u) + 3 =19, 那么 t+u=A 3B 4C 5D 6E 72、如图，三条直线相交于一点。

如果f=85, e=25, 那么a 的值是多少A 60B 65C 70D 75E 853、如果玛丽开车行驶n 英里用了t 小时，那么下列哪个可以表示她行驶的平均速度，英里/小时？A n/tB t/nC 1/ntD ntE n²t4、如果a 是一个奇数，b 是一个偶数，那么选项中哪一个是奇数？A 3bB a+3C 2(a+b)D a+2bE 2a+b5、在平面坐标内，F(-2,1),G(1,4), H(4,1)在以P为圆心的圆上，那么点P的坐标是什么？A（0,0）B（1,1）C（1,2）D（1，-2）E（,）6、如图，如果-3≤x≤6，那么x 有几个值，使得f(x)=2A 零B 一个C 两个D 三个E 三个以上7、如果t 和t+2 的算术平均值是x, t 和t-2的算术平均值是y，那么x 和y 的算术平均值是多少？A 1B 1/2C tD t+1/2E 2t8、对于任何数x 和y,假设x△y=x²+xy+y²,那么（3△1）△1等于多少？A 5B 13C 27D 170E 1839、摩根的植物在一年之内从42厘米长到57厘米。

2024 SAT考试数学历年题目精粹SAT考试是许多学生考入大学的重要考试之一，其中数学部分是考生需要面对的一项挑战。

为了帮助考生更好地备考SAT数学，本文将精选并解析2024年SAT考试数学部分的历年题目，以供参考。

第一部分：选择题题目1：一个矩形的宽度是它的长度的一半，若该矩形的面积为12，求其周长是多少？A. 12B. 14C. 16D. 18解析：设矩形的长度为l，则宽度为l/2。

根据面积的定义，我们可以列出方程l*(l/2)=12。

解方程得到l=4。

根据周长的计算公式，周长为2*l+2*(l/2)=14，选项B为正确答案。

题目2：若函数f(x)定义为f(x)=3x+1，求f(f(2))的值。

A. 9B. 10C. 11D. 12解析：由题意可知，f(2)=3*2+1=7。

将7代入函数f(x)，得到f(f(2))=f(7)=3*7+1=22。

选项D为正确答案。

题目3：已知一个等差数列的公差为3，前两个数的和为10，求这个等差数列的第n个数。

A. 3n-1B. 3n-2C. 3n-3D. 3n-4解析：设等差数列的首项为a，第n个数为an。

根据题意可得到方程a+(a+3)=10，解得a=3。

利用等差数列的通项公式an=a+(n-1)d，代入公差d=3和首项a=3，得到an=3+3(n-1)=3n。

选项A为正确答案。

第二部分：填空题题目1：若a为正整数，满足2a+5=11，则a的值为____________。

解析：将已知条件代入方程，得到2a+5=11，解得a=3。

填入空格中为3。

题目2：一个矩形的面积为25，其中宽度为5，长度为_________。

解析：设矩形的长度为l，则根据面积的定义可得到方程5l=25，解得l=5。

填入空格中为5。

第三部分：解答题题目：一辆汽车以40 mph的速度行驶了3小时，另一辆汽车以50 mph的速度行驶了t小时。

若两辆汽车行驶的距离相同，求t的值。

解析：汽车行驶的距离等于速度乘以时间，可以列出方程40*3=50*t，解得t=2.4。

SAT数学考试中包括了一些逻辑推理题，这些题目主要考察学生的逻辑推理能力和分析问题的能力，而不是单纯的数学运算。

以下是一些SAT逻辑题的例子：
题目描述：有5个不同颜色的球，分别放在两个盒子里。

如果从每个盒子中取出相同颜色的球，则两个盒子中剩下的球的颜色种类一定相同。

如果从每个盒子中取出不同颜色的球，则两个盒子中剩下的球的颜色种类一定不同。

根据以上描述，推断出以下哪个结论是正确的？
A. 如果从每个盒子中取出一个红球和一个白球，则两个盒子中剩下的球的颜色种类一定相同。

B. 如果从每个盒子中取出一个红球和一个白球，则两个盒子中剩下的球的颜色种类一定不同。

C. 如果从每个盒子中取出一个红球和一个绿球，则两个盒子中剩下的球的颜色种类一定相同。

D. 如果从每个盒子中取出一个红球和一个绿球，则两个盒子.
题目描述：有三个人，他们的职业分别是教师、医生和律师。

他们的年龄分别是二十多岁、三十多岁和四十多岁。

现在已知：甲不是教师；
乙既不是教师也不是医生；
丙既不是教师也不是律师；
甲的年龄与乙的年龄之和正好是丙的年龄的两倍。

根据以上信息，推断出以下哪个结论是正确的？
A. 甲是律师，乙是医生，丙是教师。

B. 甲是医生，乙是律师，丙是教师。

C. 甲是教师，乙是医生，丙是律师。

D. 甲是律师，乙是教师，丙是医生。

以上只是部分SAT逻辑题的例子，实际上SAT数学考试中的逻辑题还有很多种不同的形式和难度级别。

要想在考试中获得好成绩，需要多做题、多练习，提高自己的逻辑推理能力和分析问题的能力。

在回答SAT 数学题时，需要注意解题的方法和步骤。

虽然一些题目可能会被认为是难一些，但这通常是因为它们比较新颖或者涉及到了某些特定的概念或技巧。

首先，需要明确的是，SAT数学考试的目的是为了测试学生的数学知识和技能，而不是为了为难他们。

因此，即使某些题目看起来很难，只要学生掌握了必要的数学知识，并能够灵活运用，就一定能够解决它们。

接下来，让我们来看一个具体的例子。

假设有一道SAT数学题是这样的：题目：一个圆和一个球共有多少个表面？这道题目可能会让一些学生感到困惑，因为他们可能不知道如何计算一个球的表面面积。

但是，如果我们知道球和圆的性质，这个问题就会变得很简单。

首先，我们需要知道球和圆都是三维形状，而圆是二维的。

这意味着球有一个三维的表面，而圆只有一个二维的表面。

因此，要回答这个问题，我们只需要将球的表面面积除以2即可。

接下来，我们需要计算球的表面面积。

球是由一个半径为r的球体构成的，因此球的表面积公式为：4πr2。

如果我们知道球的半径r，就可以很容易地计算出球的表面面积。

现在，我们可以回到题目中来回答它。

这个圆和一个球共享一个表面，因此它们的表面之和就是球表面积的两倍。

我们可以通过将球表面积乘以2来得到答案。

那么这个答案是多少呢？通过上面的推理，我们可以得到这个问题的答案为：4πr2*2=8πr2。

这意味着圆和球的表面共有8πr2个表面。

最后，我们来总结一下这个题目的关键点和方法。

首先，我们了解球和圆的性质以及它们的表面积公式。

然后，我们利用这些知识来解决问题并得出答案。

这个方法的关键是灵活运用数学知识并理解题目中的信息。

当然，SAT数学考试中可能还会出现其他类型的难题，例如涉及复杂概念或技巧的问题。

但是只要学生掌握了必要的数学知识并能够灵活运用，就一定能够解决这些问题。

在解决SAT数学难题时，学生还需要注意一些其他事项。

首先，他们需要仔细阅读题目并理解其中的信息。

其次，他们需要使用正确的数学术语来描述问题并得出答案。

SAT数学练习题答案解析1.Correct Answer: A解析:Choice I is correct because f(x) = 6 when x=3. Choice II is incorrect because to make f(x) = 0, x2 would have to be 3. But 3 is not the square of an integer. Choice III is incorrect because to make f(x) = 0, x2 would have to be –3 but squares cannot be negative. (The minimum value for x2 is zero; hence, the minimum value for f(x) = -3)2.Correct Answer: C解析:1 < 4n + 7 < 200. n can be 0, or -1. n cannot be -2 or any other negative integer or the expression 4n + 7 will be less than1. The largest value for n will be an integer < (200 - 7) /4. 193/4 = 48.25, hence 48. The number of integers between -1 and 48 inclusive is 503.Correct Answer: B解析:First you must realize that the sum of two 2-digit numbers cannot be more that 198 (99 + 99). Therefore in the given problem D must be 1. Now use trial and error to satisfy the sum 5A + BC = 143. A + C must give 3 in the units place, but neither can be 1 since all the digits have to be different. Therefore A + C = 13. With one to carry over into the tens column, 1 + 5 + B = 14, and B = 8. A + C + B + D = 13 + 8 + 1 = 224.Correct Answer: B解析:Total volume of water = 12 liters = 12 x 1000 cm3. The base of the aquarium is 50 x 30 = 1500cm3. Base of tank x height of water = volume of water. 1500 x height = 12000; height = 12000 / 1500 = 85.Correct Answer: A解析:Let Ben’s age now be B. Anita’s age now is A. (A - 6) = P(B - 6)But A is 17 and therefore 11 = P(B - 6). 11/P = B-6(11/P) + 6 = BSAT数学练习题答案解析1.Correct Answer: E解析:Do not assume that AB and C are on a straight line. Make a diagram with A and B marked 5 miles apart. Draw a circle centered on B, with radius 6. C could be anywhere on this circle. The minimum distance will be 1, and maximum 11, but anywhere in between is possible.2.Correct Answer: B解析:We can write the statement mathematically, using x to mean ‘of’ and /100 for ‘per cent’. So ( √5/100) x 5√5 = 5 x 5 /100 = 0.253.Correct Answer: D解析:If pqr = 1, none of these variable can be zero. Since spr = 0 , and since p and r are not zero, s must be zero. (Note that although rst = 0, and so either s or t must be zero, this is not sufficient to state which must be zero)4.Correct Answer: E解析:65 = 64x 6(64 x 6) - 64 = 64(6 - 1) = 64 x 5 Now, dividing by 5 will give us 645.Correct Answer: E解析:All terms in the sequence will be multiples of 4. 762 is not a multiple of 4SAT数学练习题答案解析1.Correct Answer: BExplanation:The marked angle, ABC must be more than 90 degrees because it is the external angle of triangle BDC, and must be equal to the sum of angles BDC (90) and DCB. Also ABC is not a straight line and must be less than 180. Therefore 90 < 5x < 180 The only value of x which satisfies this relation is 20.2.Correct Answer: AExplanation:20 large cakes will require the equivalent of 10 helpers working for one hour. 700 small cakes will require the equivalent of 20 helpers working for one hour. This means if only one hour were available we would need 30 helpers. But since three hours are available we can use 10 helpers.3.Correct Answer: EExplanation:Indian stamps are common to both ratios. Multiply both ratios by factors such that the Indian stamps are represented by the same number. US : Indian = 5 : 2, and Indian : British = 5 : 1. Multiply the first by 5, and the second by 2.Now US : Indian = 25 : 10, and Indian : British = 10 : 2 Hence the two ratios can be combined and US : British = 25 : 24.Correct Answer: CExplanation:Draw the diagram. The diagonal of the rectangle is the diameter of the circle. The diagonal is the hypotenuse of a 3,4,5 triangle, and is therefore, 5. Circumference = π.diameter = 5π5.Correct Answer: DExplanation:If two sets of four consecutive integers have one integer in common, the total in the combined set is 7., and we can write the sets as n + (n + 1) + (n + 2) + (n + 3 ) and (n + 3) + (n + 4) + (n + 5) + (n + 6) Note that each term in the second set is 3 more than the equivalent term in the first set. Since there are four terms the total of the differences will be 4 x 3 = 12SAT数学练习题答案解析1.Correct Answer: DExplanation:You can solve this by back solving – substitute the answer choices in the expression and see which gives the greatest value.satA (-1 + 2) / (-1-2) = -2 / 2 = -1;B (0 + 2) / (0-2) = 2/ -2 = -1;C (1 + 2) / (1-2) = 3/-1 = -3;D (3 + 2) / (3-2) = 5/1 = 5;E (4+ 2) / (4-2) = 6/2 = 3If you had just chosen the largest value for x you would have been wrong. So although it looks a long method, it is actually quick and accurate since the numbers are really simple and you can do the math in your head.2.Correct Answer: DExplanation:(Total area of square - sum of the areas of triangles ADE and DCF) will give the area of the quadrilateral 9 - (2 x ? x 3 x 1.5) = 4.53.Correct Answer: EExplanation:Remember that n could be positive negative or a fraction. Try out a few cases: In case I, if n is -1, then 2n is less than n. In case II, if n is a fraction such as ? then n2 will be less than n. In case III, if n is 2, then 2-n = 0, which is less than n. Therefore, none of the choices must be greater than n4.Correct Answer: CExplanation:If after each bounce it reaches 2/5 of the previous height, then after the second bounce it will reach 2/5 x 125. After the third it will reach 2/5 x 2/5 x 125. After the fourth it will reach 2/5 x 2/5 x 2/5 x 125. This cancels down to 2 x 2 x 2 = 85.Correct Answer: AExplanation:The smallest value for n such that 5n is a square is 5. 75np can now be written as 75 x 5 x p. This gives prime factors.... 3 x 5 x 5 x 5 x p To make the expression a perfect cube, p will have to have factors 3 x 3 , and hence p =9 n + p = 5 + 9 = 14SAT数学练习题答案解析1.Correct Answer: A解析:a4 = a2 x a2 = 12 x 12 = 1442.Correct Answer: B解析:In case I , even plus odd will give odd. In case II, odd times even will give even. In case III even squared is even, and even minus odd is odd. (You can check this by using an easy even number like 2 in place of n). Only case II cannot be odd.3.Correct Answer: D解析:The maximum area of the triangle will come when the given sides are placed at right angles. If we take 8 as the base and 5 as the height the area = ? x 8 x 5 = 20. We can alter the angle between the sides to make it less or more than 90, but this will only reduce the area. (Draw it out for yourself). Hence the area can be anything less than or equal to 20.4.Correct Answer: E解析:Food consumed per day = 39/6. In the remaining days it will consume 91 - 39 pounds = 52 pounds. Now divide the food by the daily consumption to find the number of days. 52 / (39/6) = 52 x (6 / 39) = 85.Correct Answer: C解析:A perfect cube will have prime factors that are in groups of 3; for example 125 has the prime factors5 x 5 x 5 , and 64 x 125 will also be a cube because its factors will be 4 x 4 x 4 x 5 x 5 x 5. Consider the answer choices in turn. 8 is the cube of 2, and p is a cube, and so the product will also be a cube. pq will also be a cube as shown above.pq is a cube and so is 27, but their sum need not be a cube. Consider the case where p =1 and q = 8, the sum of pq and 27 will be 35 which has factors 5 x 7 and is not a cube. -p will be a cube. Since the difference between p and q is raised to the power of 6, this expression will be a cube (with cube root = difference squared).。