材料科学基础第一章习题答案
- 格式:doc
- 大小:177.50 KB
- 文档页数:15

第一章作业——材料结构的基本知识1、简述一次键与二次键的差异及各键的特点。
2、简述三大类材料中的结合键类型及性能(物性、力性)特点。
3、为什么金属材料的密度比陶瓷材料及高分子材料密度高?4、用金属键的特征解释金属材料的性能-----①良好的导电;②良好的导热性;③正的电阻温度系数;④不透明性及具有金属光泽;⑤良好的塑性⑥金属之间的溶解性(固溶能力)。
5、简述晶体与非晶体的主要区别。
6、简述原子结构、原子结合键、原子的排列方式及显微组织对材料性能的影响。
第一章作业解答1、述一次键与二次键的差异及各键的特点。
解答:(1)一次键结合力较强,包括金属键、离子键、共价键;二次键结合力较弱,包括范德华键和氢键。
一次键主要依靠外壳层电子转移或共享以形成稳定的电子壳层;二次键是借原子之间的偶极吸引力结合而成。
(2)金属键电子共有化,没有方向性和饱和性;离子键没有方向性,但要满足正负电荷平衡要求;共价键有明显的方向性和饱和性;范德华键没有方向性、饱和性;氢键(X-H…Y)有饱和性、方向性。
2、简述三大类材料中的结合键类型及性能(物性、力性)特点。
解答:(1)三大类材料主要指金属材料、陶瓷材料和高分子材料。
(2)金属材料中的结合键主要是金属键,其次是共价键、离子键,使金属材料具有较高的熔点、密度,良好的导电、导热性能及较高的弹性模量、强度和塑性。
陶瓷材料中的结合键主要是离子键和共价键,使其熔点高、密度低,具有良好的绝缘性能和绝热性能,高的弹性模量和强度,但塑性差,脆性大。
高分子材料中分子链内部虽为共价键结合,但分子链之间为二次键结合,使其具有较低的熔点、密度,良好的绝缘性能、绝热性能及较低的弹性模量、强度和塑性。
3、为什么金属材料的密度比陶瓷材料及高分子材料密度高?金属材料的密度较高是因为①金属元素具有较高的相对原子质量,②金属材料主要以金属键结合,金属键没有方向性和饱和性,使金属原子总是趋于密集排列,达到密堆结构。

第一章 原子排列与晶体结构1. fcc 结构的密排方向是 ,密排面是 ,密排面的堆垛顺序是 ,致密度为 ,配位数是 ,晶胞中原子数为 ,把原子视为刚性球时,原子的半径r 与点阵常数a 的关系是 ;bcc 结构的密排方向是 ,密排面是 ,致密度为 ,配位数是 ,晶胞中原子数为 ,原子的半径r 与点阵常数a 的关系是 ;hcp 结构的密排方向是 ,密排面是 ,密排面的堆垛顺序是 ,致密度为 ,配位数是 ,晶胞中原子数为 ,原子的半径r 与点阵常数a 的关系是 。
2. Al 的点阵常数为0.4049nm ,其结构原子体积是 ,每个晶胞中八面体间隙数为 ,四面体间隙数为 。
3. 纯铁冷却时在912ε 发生同素异晶转变是从 结构转变为 结构,配位数 ,致密度降低 ,晶体体积 ,原子半径发生 。
4. 在面心立方晶胞中画出)(211晶面和]211[晶向,指出﹤110﹥中位于(111)平面上的方向。
在hcp 晶胞的(0001)面上标出)(0121晶面和]0121[晶向。
5. 求]111[和]120[两晶向所决定的晶面。
6 在铅的(100)平面上,1mm 2有多少原子?已知铅为fcc 面心立方结构,其原子半径R=0.175×10-6mm 。
第二章 合金相结构一、 填空1) 随着溶质浓度的增大,单相固溶体合金的强度 ,塑性 ,导电性 ,形成间隙固溶体时,固溶体的点阵常数 。
2) 影响置换固溶体溶解度大小的主要因素是(1) ;(2) ;(3) ;(4) 和环境因素。
3) 置换式固溶体的不均匀性主要表现为 和 。
4) 按照溶质原子进入溶剂点阵的位置区分,固溶体可分为 和 。
5) 无序固溶体转变为有序固溶体时,合金性能变化的一般规律是强度和硬度 ,塑性 ,导电性 。
6)间隙固溶体是 ,间隙化合物是 。
二、 问答1、 分析氢,氮,碳,硼在α-Fe 和γ-Fe 中形成固溶体的类型,进入点阵中的位置和固溶度大小。
已知元素的原子半径如下:氢:0.046nm ,氮:0.071nm ,碳:0.077nm ,硼:0.091nm ,α-Fe :0.124nm ,γ-Fe :0.126nm 。

材料科学基础习题参考答案 第一章材料结构的基本知识8.计算下列晶体的离于键与共价键的相对比例。
(1) NaF (2) CaO (3) ZnS解:(1)查表得:X Na =0.93,X F =3.98--(0.93-3.98)2根据鲍林公式可得NaF 中离子键比例为:[1-e 4 ]x 100% = 90.2%共价键比例为:1-90.2%=9.8%--(1.00-3.44 )2(2) 同理,CaO 中离子键比例为:[1-e 4 ]x 100% = 77.4%共价键比例为:1-77.4%=22.6%(3) ZnS 中离子键比例为:Z“S 中离子键含量=[1 -£-1/4'2-58-165)2]x 100% = 19.44% 共价键比例为:1-19.44%=80.56%10说明结构转变的热力学条件与动力学条件的意义.说明稳态结构与亚稳态结构之间的关 系。
答:结构转变的热力学条件决定转变是否可行,是结构转变的推动力,是转变的必要条件; 动力学条件决定转变速度的大小,反映转变过程中阻力的大小。
稳态结构与亚稳态结构之间的关系:两种状态都是物质存在的状态,材料得到的结构是 稳态或亚稳态,取决于转变过程的推动力和阻力(即热力学条件和动力学条件),阻力小时得 到稳态结构,阻力很大时则得到亚稳态结构。
稳态结构能量最低,热力学上最稳定;亚稳态 结构能量高,热力学上不稳定,但向稳定结构转变速度慢,能保持相对稳定甚至长期存在。
但在一定条件下,亚稳态结构向稳态结构转变。
1.第二章九材料中的騒須勾)与[2廊1)与[112], (110)与[111], (132)与[123], (322)与[236]指数。
题: 系的 (21 在立方晶系的一个晶胞虫画出(111丄和丄112、日面.才晶系的画出同M1)、■'朋两晶面交钱亠 1]晶向。
112) d2. 有一正交点阵的a=b, c=a/2o 某晶面在三个晶轴上的截距分别为6个、2个和4个原子 间距,求该晶面的密勒指数。

第1章 习题
1-10 纯铁点阵常数0.286nm ,体心立方结构,求1cm 3中有多少铁原子。
解:体心立方结构单胞拥有两个原子,单胞的体积为
V =(0.286×10-8)3 cm 3,所以1cm 3中铁原子的数目为
2283128.5510(2.8610)Fe n -=
⨯=⨯⨯
1-11 一个位错环能否各部分都是螺型位错,能否各部分都是刃型位错?为什么?
解:螺型位错的柏氏矢量与位错线平行,一根位错只有一个柏氏矢量,而一个位错环不可能与一个方向处处平行,所以一个位错环不能各部分都是螺型位错。
刃位错的柏氏矢量与位错线垂直,如果柏氏矢量垂直位错环所在的平面,则位错环处处都是刃型位错。
这种位错的滑移面是位错环与柏氏矢量方向组成的棱柱面,这种位错又称棱柱位错。
1-15 有一正方形位错线,其柏氏矢量及位错线的方向如图1-51所示。
试指出图中各段位错线的性质,并指出刃型位错额外串原子面所处的位置。
解:由柏氏矢量与位错线的关系可以知道,DC 是右螺型位错,BA 是左螺型位错。
由右手法则,CB 为正刃型位错,多余半原子面在纸面上方。
AD 为负刃型位错,多余半原子面在纸面下方。
A B
C
D。

第一章材料的结构一、解释以下基本概念空间点阵、晶格、晶胞、配位数、致密度、共价键、离子键、金属键、组元、合金、相、固溶体、中间相、间隙固溶体、置换固溶体、固溶强化、第二相强化。
二、填空题1、材料的键合方式有四类,分别是(),(),(),()。
2、金属原子的特点是最外层电子数(),且与原子核引力(),因此这些电子极容易脱离原子核的束缚而变成()。
3、我们把原子在物质内部呈()排列的固体物质称为晶体,晶体物质具有以下三个特点,分别是(),(),()。
4、三种常见的金属晶格分别为(),()和()。
5、体心立方晶格中,晶胞原子数为(),原子半径与晶格常数的关系为(),配位数是(),致密度是(),密排晶向为(),密排晶面为(),晶胞中八面体间隙个数为(),四面体间隙个数为(),具有体心立方晶格的常见金属有()。
6、面心立方晶格中,晶胞原子数为(),原子半径与晶格常数的关系为(),配位数是(),致密度是(),密排晶向为(),密排晶面为(),晶胞中八面体间隙个数为(),四面体间隙个数为(),具有面心立方晶格的常见金属有()。
7、密排六方晶格中,晶胞原子数为(),原子半径与晶格常数的关系为(),配位数是(),致密度是(),密排晶向为(),密排晶面为(),具有密排六方晶格的常见金属有()。
8、合金的相结构分为两大类,分别是()和()。
9、固溶体按照溶质原子在晶格中所占的位置分为()和(),按照固溶度分为()和(),按照溶质原子与溶剂原子相对分布分为()和()。
10、影响固溶体结构形式和溶解度的因素主要有()、()、()、()。
11、金属化合物(中间相)分为以下四类,分别是(),(),(),()。
12、金属化合物(中间相)的性能特点是:熔点()、硬度()、脆性(),因此在合金中不作为()相,而是少量存在起到第二相()作用。
13、CuZn、Cu5Zn8、Cu3Sn的电子浓度分别为(),(),()。
14、如果用M表示金属,用X表示非金属,间隙相的分子式可以写成如下四种形式,分别是(),(),(),()。

第一章材料的结构一、解释以下基本概念空间点阵、晶格、晶胞、配位数、致密度、共价键、离子键、金属键、组元、合金、相、固溶体、中间相、间隙固溶体、置换固溶体、固溶强化、第二相强化.二、填空题1、材料的键合方式有四类,分别是(),( ),(),().2、金属原子的特点是最外层电子数(),且与原子核引力(),因此这些电子极容易脱离原子核的束缚而变成( )。
3、我们把原子在物质内部呈( )排列的固体物质称为晶体,晶体物质具有以下三个特点,分别是(),( ),( ).4、三种常见的金属晶格分别为(),( )和().5、体心立方晶格中,晶胞原子数为( ),原子半径与晶格常数的关系为( ),配位数是(),致密度是( ),密排晶向为(),密排晶面为( ),晶胞中八面体间隙个数为(),四面体间隙个数为( ),具有体心立方晶格的常见金属有()。
6、面心立方晶格中,晶胞原子数为( ),原子半径与晶格常数的关系为(),配位数是( ),致密度是(),密排晶向为( ),密排晶面为(),晶胞中八面体间隙个数为( ),四面体间隙个数为(),具有面心立方晶格的常见金属有()。
7、密排六方晶格中,晶胞原子数为(),原子半径与晶格常数的关系为(),配位数是(),致密度是(),密排晶向为( ),密排晶面为(),具有密排六方晶格的常见金属有( )。
8、合金的相结构分为两大类,分别是()和( )。
9、固溶体按照溶质原子在晶格中所占的位置分为()和(),按照固溶度分为()和(),按照溶质原子与溶剂原子相对分布分为()和()。
10、影响固溶体结构形式和溶解度的因素主要有()、()、()、()。
11、金属化合物(中间相)分为以下四类,分别是( ),( ),( ),( )。
12、金属化合物(中间相)的性能特点是:熔点()、硬度( )、脆性(),因此在合金中不作为()相,而是少量存在起到第二相()作用。
13、CuZn、Cu5Zn8、Cu3Sn的电子浓度分别为(),( ),( ).14、如果用M表示金属,用X表示非金属,间隙相的分子式可以写成如下四种形式,分别是( ),(),( ),( ).15、Fe3C的铁、碳原子比为(),碳的重量百分数为(),它是( )的主要强化相。


第一章原子结构与键合1. 主量子数n、轨道角动量量子数l i、磁量子数m i和自旋角动量量子数S i。
2. 能量最低原理、Pauli不相容原理,Hund规则。
3. 同一周期元素具有相同原子核外电子层数,但从左→右,核电荷依次增多,原子半径逐渐减小,电离能增加,失电子能力降低,得电子能力增加,金属性减弱,非金属性增强;同一主族元素核外电子数相同,但从上→下,电子层数增多,原子半径增大,电离能降低,失电子能力增加,得电子能力降低,金属性增加,非金属性降低;4. 在元素周期表中占据同一位置,尽管它们的质量不同,然它们的化学性质相同的物质称为同位素。
由于各同位素的含中子量不同(质子数相同),故具有不同含量同位素的元素总的相对原子质量不为正整数。
5. 52.0576. 73% (Cu63); 27% (Cu65)8. a:高分子材料;b:金属材料;c:离子晶体10.a) Al2O3的相对分子质量为M=26.98×2+16×3=101.961mm3中所含原子数为1.12*1020(个)b) 1g中所含原子数为2.95*1022(个)11. 由于HF分子间结合力是氢键,而HCl分子间结合力是范德化力,氢键的键能高于范德化力的键能,故此HF的沸点要比HCl的高。
第2章固体结构1.每单位晶胞内20个原子2.CsCl型结构系离子晶体结构中最简单一种,属立方晶系,简单立方点阵,Pm3m空间群,离子半径之比为0.167/0.181=0.92265,其晶体结构如图2-13所示。
从图中可知,在<111> 方向离子相接处,<100>方向不接触。
每个晶胞有一个Cs+和一个Cl-,的配位数均为8。
3.金刚石的晶体结构为复杂的面心立方结构,每个晶胞共含有8个碳原子。
金刚石的密度(g/cm3)对于1g碳,当它为金刚石结构时的体积(cm3)当它为石墨结构时的体积(cm3)故由金刚石转变为石墨结构时其体积膨胀4.]101[方向上的线密度为1.6. 晶面族{123}=(123)+(132)+(213)+(231)+(321)+(312)+)231(+)321(+)132(+)312(+)213(+)123(+)321(+)231(+)312(+)132(+)123(+)213(+)312(+)213(+)321(+)123(+)132(+)231(晶向族﹤221﹥=[221]+[212]+[122]+]212[+]122[+]221[+]122[+]212[+]221[+]122[+]221[+]212[7. 晶带轴[uvw]与该晶带的晶面(hkl)之间存在以下关系:hu+kv+lw=0;将晶带轴[001]代入,则h×0+k×0+l×1=0;当l=0时对任何h,k取值均能满足上式,故晶带轴[001]的所有晶带面的晶面指数一般形式为(hk0)。


附:习题答案0 绪论一、填空题1. 结构,性能2. 原子结构、原子的空间排列、显微组织3.结构材料,功能材料;金属材料,无机非金属材料(陶瓷),高分子材料(聚合物)。
第1章一、填空题1、金属键,离子键、共价键,分子键、共价键2、金属键、离子键、共价键,_范德华键_、氢键。
3、共价键4、共价键5、共价键,氢键二、判断题(1)错。
离子键没有方向性和饱和性。
(2)对。
(3)错。
改为:S原子轨道的角度分布图为一个从原子核为球心的球面,而s电子云图是一个球体,其剖面图是个圆。
P轨道的角度分布图为两个在原点相切的球面。
而p电子云图像或几率分布是一个哑铃形体,其剖面图是∞字型。
(4)错。
改为:若用小黑点的疏度表示几率密度的大小,则黑点密的地方,表示|Ψ|2数值大,电子出现的几率大;黑点稀的地方表示|Ψ|2数值小,电子出现的几率小。
(5)错。
改为:主量子数为4时,有4s、4p、4d、4f四个亚层。
共16条轨道。
(6)错。
改为:多电子原子轨道能级与氢原子能级不同。
三、简答题1. H>He;Ba>Sr;Sc<Ca;Cu>N;Zr≈Hf;La>Gd;S2->S;Na>Al3+;Fe2+>Fe3+;Pb2+>Sn2+2.(1)金属性:Ge>Si>As (2) 电离能:As>Si>Ge(3) 电负性:As>Si≈Ge (4) 原子半径:Ge>As>Si3.因氢原子核外只有一个电子,核外运动的电子能量由主量子数n决定,n相同时能量相同。
而氯原子中核外有17个电子,核外运动的电子能量不仅与主量子数n有关,而且也与角量子数L有关,3s的穿透能力大于3p,故3s能级低于3p能级。
第2章综合习题----基础练习 一、填空题1、基元(原子团)以周期性重复方式在三维空间作有规则的排列的固体 。
2、是否在三维空间作周期性重复规则排列( 晶体原子排布长程有序,非晶体是长程无序短程有序) 。
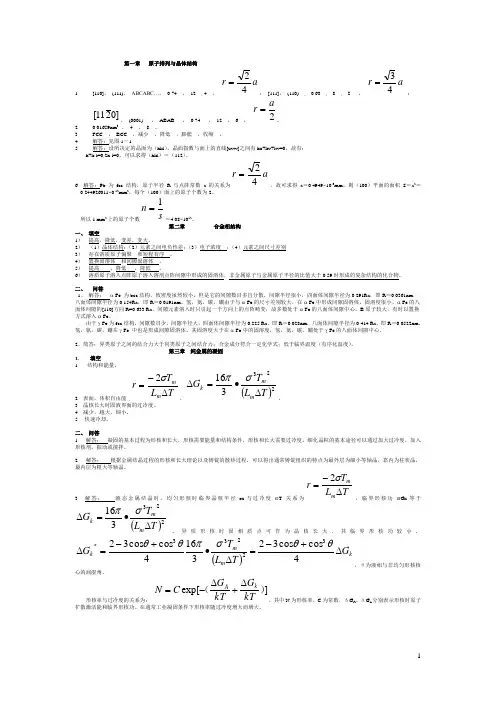
第一章 原子排列与晶体结构1.[110], (111), ABCABC…, 0.74 , 12 , 4 , a r 42=; [111], (110) , 0.68 , 8 , 2 , a r 43= ;]0211[, (0001) , ABAB , 0.74 , 12 , 6 , 2a r =。
2. 0.01659nm 3 , 4 , 8 。
3. FCC , BCC ,减少 ,降低 ,膨胀 ,收缩 。
4. 解答:见图1-15.解答:设所决定的晶面为(hkl ),晶面指数与面上的直线[uvw]之间有hu+kv+lw=0,故有: h+k-l=0,2h-l=0。
可以求得(hkl )=(112)。
6 解答:Pb 为fcc 结构,原子半径R 与点阵常数a 的关系为ar 42=,故可求得a =0.4949×10-6mm 。
则(100)平面的面积S =a 2=0.244926011×0-12mm 2,每个(100)面上的原子个数为2。
所以1 mm 2上的原子个数s n 1==4.08×1012。
第二章合金相结构一、 填空1) 提高,降低,变差,变大。
2) (1)晶体结构;(2)元素之间电负性差;(3)电子浓度 ;(4)元素之间尺寸差别 3) 存在溶质原子偏聚 和短程有序 。
4) 置换固溶体 和间隙固溶体 。
5) 提高 ,降低 ,降低 。
6) 溶质原子溶入点阵原子溶入溶剂点阵间隙中形成的固溶体,非金属原子与金属原子半径的比值大于0.59时形成的复杂结构的化合物。
二、 问答1、 解答: α-Fe 为bcc 结构,致密度虽然较小,但是它的间隙数目多且分散,间隙半径很小,四面体间隙半径为0.291Ra ,即R =0.0361nm ,八面体间隙半径为0.154Ra ,即R =0.0191nm 。
氢,氮,碳,硼由于与α-Fe 的尺寸差别较大,在α-Fe 中形成间隙固溶体,固溶度很小。
材料科学基础答案(精⼼整理)第1章晶体结构1.在⽴⽅晶系中,⼀晶⾯在x轴的截距为1,在y轴的截距为1/2,且平⾏于z 轴,⼀晶向上某点坐标为x=1/2,y=0,z=1,求出其晶⾯指数和晶向指数,并绘图⽰之。
2.画出⽴⽅晶系中下列晶⾯和晶向:(010),(011),(111),(231),(321),[010], [011],[111],[231],[321]。
3.纯铝晶体为⾯⼼⽴⽅点阵,已知铝的相对原⼦质量Ar(Al)=27,原⼦半径r=0.143nm,求铝晶体的密度。
4.何谓晶体?晶体与⾮晶体有何区别?5.试举例说明:晶体结构与空间点阵?单位空间格⼦与空间点阵的关系?6.什么叫离⼦极化?极化对晶体结构有什么影响?7.何谓配位数(离⼦晶体/单质)?8.何谓对称操作,对称要素?9.计算⾯⼼⽴⽅结构(111)与(100)晶⾯的⾯间距及原⼦密度(原⼦个数/单位⾯积)。
10.已知室温下α-Fe(体⼼)的点阵常数为0.286nm,分别求(100)、(110)、(123)的晶⾯间距。
11.已知室温下γ-Fe(⾯⼼)的点阵常数为0.365nm,分别求(100)、(110)、(112)的晶⾯间距。
12.已知Cs+半径为0.170nm,Cl-半径为0.181 nm,计算堆积系数。
13.MgO 属NaCl型结构,若rMg 2+=0.078nm,rO2-=0.132nm,(1)试⽤鲍林规则分析氧化镁晶体结构?(2)计算堆积密度?(3)画出氧化镁在(100)、(110)、(111)晶⾯上的结点和离⼦排布图?答案1.答:晶⾯指数为:(120),见图ABCD ⾯;晶向指数为:[102],见图OP 向。
2.答:3. 4. 5.6. 答:离⼦极化:在离⼦紧密堆积时,带电荷的离⼦所产⽣的电场必然要对另⼀离⼦的电⼦云发⽣作⽤(吸引或排斥),因⽽使这个离⼦的⼤⼩和形状发⽣了改变,这种现象叫离⼦极化。
极化会对晶体结构产⽣显著影响,主要表现为极化会导致离⼦间距离缩短,离⼦配位数降低,同时变形的电⼦云相互重叠,使键性由离⼦键向共价键过渡,最终使晶体结构类型发⽣变化。
材料科学基础课后习题答案材料科学基础课后习题答案第一章:晶体结构和晶体缺陷1. 什么是晶体?晶体的特点是什么?答:晶体是由有序排列的原子、离子或分子组成的固态材料。
晶体的特点包括有规则的、重复的、周期性的结构,具有明确的晶体面和晶面间角度。
2. 简述晶体中离子束缚以及普通共价键束缚的区别?答:晶体中离子束缚是指由电荷相反的离子通过电磁力相互吸引而形成的结合力,例如NaCl晶体。
普通共价键束缚是由共享电子对形成的,例如金刚石晶体。
离子束缚通常较为强烈,晶体具有高熔点和脆性;而共价键束缚相对较弱,晶体具有低熔点和韧性。
3. 什么是晶体缺陷?列举几种晶体缺陷并简要描述其影响。
答:晶体缺陷是指晶体中排列异常的原子、离子或分子。
常见的晶体缺陷包括点缺陷、线缺陷和面缺陷。
点缺陷指的是晶体中原子位置的缺失或替代,如空位、间隙原子和杂质原子;线缺陷是晶体中晶面上原子位置的错误,如位错和螺旋位错;面缺陷是指晶面之间的错配,如晶界和孪生界。
这些晶体缺陷会影响晶体的物理性质和力学性能。
4. 什么是晶体结构中的定义因素?它们的作用是什么?答:晶体结构中的定义因素包括晶胞和晶格参数。
晶胞是最小重复单元,由一定数量的晶体中的原子、离子或分子组成。
晶格参数描述晶胞的大小和形状。
晶胞和晶格参数共同定义了晶体的结构。
晶胞和晶格参数的作用是确定晶体的晶体面、晶面间角度以及晶体的物理性质。
5. 什么是晶格点?晶格点的种类有哪些?答:晶格点是位于晶体内部的原子、离子或分子的位置。
晶格点的种类包括普通晶格点、间隙晶格点和特殊晶格点。
普通晶格点是晶体中原子、离子或分子的晶格点,如AB型晶体中的A和B原子;间隙晶格点是晶体中没有原子、离子或分子的晶格点,如金刚石中的间隙晶格点;特殊晶格点是具有非普通晶格点性质的晶体中的晶格点,如晶体中的空位或杂质原子。
第二章:物质的结构与性能关系1. 简述晶体结构对物质性能的影响。
答:晶体结构直接影响物质的物理性质和化学性质。
材料科学基础经典习题及答案第一章 材料科学基础1.作图表示立方晶体的()()()421,210,123晶面及[][][]346,112,021晶向。
2.在六方晶体中,绘出以下常见晶向[][][][][]0121,0211,0110,0112,0001等。
3.写出立方晶体中晶面族{100},{110},{111},{112}等所包括的等价晶面。
4.镁的原子堆积密度和所有hcp 金属一样,为0.74。
试求镁单位晶胞的体积。
已知Mg 的密度3Mg/m 74.1=mg ρ,相对原子质量为24.31,原子半径r=0.161nm 。
5.当CN=6时+Na 离子半径为0.097nm ,试问:1) 当CN=4时,其半径为多少?2) 当CN=8时,其半径为多少?6. 试问:在铜(fcc,a=0.361nm )的<100>方向及铁(bcc,a=0.286nm)的<100>方向,原子的线密度为多少?7.镍为面心立方结构,其原子半径为nm 1246.0=Ni r 。
试确定在镍的(100),(110)及(111)平面上12mm 中各有多少个原子。
8. 石英()2SiO 的密度为2.653Mg/m 。
试问: 1) 13m 中有多少个硅原子(与氧原子)?2) 当硅与氧的半径分别为0.038nm 与0.114nm 时,其堆积密度为多少(假设原子是球形的)?9.在800℃时1010个原子中有一个原子具有足够能量可在固体内移动,而在900℃时910个原子中则只有一个原子,试求其激活能(J/原子)。
10.若将一块铁加热至850℃,然后快速冷却到20℃。
试计算处理前后空位数应增加多少倍(设铁中形成一摩尔空位所需要的能量为104600J )。
11.设图1-18所示的立方晶体的滑移面ABCD 平行于晶体的上、下底面。
若该滑移面上有一正方形位错环,如果位错环的各段分别与滑移面各边平行,其柏氏矢量b ∥AB 。
1) 有人认为“此位错环运动移出晶体后,滑移面上产生的滑移台阶应为4个b ,试问这种看法是否正确?为什么?2)指出位错环上各段位错线的类型,并画出位错运动出晶体后,滑移方向及滑移量。
第一章材料的结构一、解释以下基本概念空间点阵、晶格、晶胞、配位数、致密度、共价键、离子键、金属键、组元、合金、相、固溶体、中间相、间隙固溶体、置换固溶体、固溶强化、第二相强化。
二、填空题1、材料的键合方式有四类,分别是(),(),(),()。
2、金属原子的特点是最外层电子数(),且与原子核引力(),因此这些电子极容易脱离原子核的束缚而变成()。
3、我们把原子在物质内部呈()排列的固体物质称为晶体,晶体物质具有以下三个特点,分别是(),(),()。
4、三种常见的金属晶格分别为(),()和()。
5、体心立方晶格中,晶胞原子数为(),原子半径与晶格常数的关系为(),配位数是(),致密度是(),密排晶向为(),密排晶面为(),晶胞中八面体间隙个数为(),四面体间隙个数为(),具有体心立方晶格的常见金属有()。
6、面心立方晶格中,晶胞原子数为(),原子半径与晶格常数的关系为(),配位数是(),致密度是(),密排晶向为(),密排晶面为(),晶胞中八面体间隙个数为(),四面体间隙个数为(),具有面心立方晶格的常见金属有()。
7、密排六方晶格中,晶胞原子数为(),原子半径与晶格常数的关系为(),配位数是(),致密度是(),密排晶向为(),密排晶面为(),具有密排六方晶格的常见金属有()。
8、合金的相结构分为两大类,分别是()和()。
9、固溶体按照溶质原子在晶格中所占的位置分为()和(),按照固溶度分为()和(),按照溶质原子与溶剂原子相对分布分为()和()。
10、影响固溶体结构形式和溶解度的因素主要有()、()、()、()。
11、金属化合物(中间相)分为以下四类,分别是(),(),(),()。
12、金属化合物(中间相)的性能特点是:熔点()、硬度()、脆性(),因此在合金中不作为()相,而是少量存在起到第二相()作用。
13、CuZn、Cu5Zn8、Cu3Sn的电子浓度分别为(),(),()。
14、如果用M表示金属,用X表示非金属,间隙相的分子式可以写成如下四种形式,分别是(),(),(),()。
材料科学基础第一章习题答案1. (P80 3-3) Calculate the atomic radius in cm for the following:(a) BCC metal with a 0=0.3294nm and one atom per lattice point; and(b) FCC metal with a 0=4.0862Å and one atom per lattice point.Solution:(a) In BCC structures, atoms touch along the body diagonal, which is 3a 0 in length. There are two atomic radii from the center atom and one atomic radius from each of the corner atoms on the body diagonal,so 340ra =430a r ==0.14263nm=1.4263810-⨯cm (b) In FCC structures, atoms touch along the face diagonal of the cube, which is02a in length. There are four atomic radii along this length —two radii from the face-centered atom and one radius from each corner, so240r a =, 420a r ==1.44447 Å=1.44447810-⨯cm2.(P80 3-4) determine the crystal structure for the following:(a) a metal with a0=4.9489Å, r=1.75Å, and one atom per lattice point; and (b) a metal with a0=0.42906nm, r=0.1858nm, and one atom per lattice point.Solution:We know the relationships between atomic radii and lattice parameters are 430a r =in BCC and 420a r =in FCC. (a) 420a r ==⨯=49489.42 1.75 so its crystal structure is FCC;(b) 430a r ==44296.03⨯=0.186nm so its crystal structure is BCC.3. (P80 3-5) the density of potassium, which has the BCC structure and oneatom per lattice point, is 0.855g/cm 3.the atomic weight of potassium is 39.09g/mol. Calculate(a) the lattice parameter; and(b) the atomic radius of potassium.Solution(a) For a BCC unit cell, there are twoatoms in per unit cell,atomic mass is 39.09g/mol,density ρ=0.855g/cm 3Avogadro ’s number N A =6.022310⨯atoms/mol())'()()(/snumber Avogadro cell unit of volume mass atomic cell atoms of numbers =ρ0.855g/cm 3=)/()1002.6()/(09.392233mol atoms a mol g atoms ⨯⨯⨯ So a=3231002.6855.009.392⨯⨯⨯=0.53cm710-⨯=5.3Å(b)then r=a 43=0.229710-⨯cm=2.29Å4. (P81 3-20) determine the indices forthe directions in the cubic unit cell shown in Figure 3-32.The procedure for finding the Miller indices for directions is as follows:ing a right-handed coordinatesystem, determine the coordinates of two points, which lie on the direction.2.Subtract the coordinates of the “tail”point from the coordinates of the “head”point to obtain the number oflattice parameters traveled in the direction of each axis of the coordinate system.3.Clear fractions and/or reduce the results obtained from the subtraction to lowest integers.4.Enclose the number in square brackets[ ]. If a negative sign is produced, represent the negative sign with a bar over the number.SolutionDirection A1.Two points are 0,0,1 and 1,0,02.0,0,1-1,0,0=-1,0,13.no fraction to clear or integers to reduce4.[]011Direction B 1.Two points are 1,0,1 and 21,1,0 2.1,0,1-21,1,0=21,-1,1 3.2(21,-1,1)=1,-2,24.[]221Direction C 1.Two points are 1,0,0 and 0,43,1 2.1,0,0-0,43,1=1, -43,-1 3.4(1, -43,-1)=4, -3, -4 4.[]434Direction D 1.Two points are 0,1,21 and 1,0,0 2.0,1,21 -1,0,0=-1,1, 21 3.2(-1,1, 21)=-2,2,1 4.[]2125.(P82 3-22) Determine the indices for the planes in the cubic unit cell shown in Figure 3-34.The procedure for finding the Miller indices for planes is as follows:1. Identify the points at which the plane intercepts the x, y, and z coordinates in terms of the number of lattice parameters. If the plane passes throughthe origin, the origin of the coordinate system must be moved!2. Take reciprocals of these intercepts.3. Clear fractions but not reduce to lowest integers.4. Enclose the resulting numbers in parentheses (). Again, negative numbers should be written with a bar over the number.SolutionPlane A 1. x=-1, y=21, z=43 2. 341,21,11==-=z y x3. Clear fractions: -3, 6, 44. (643)Plane B 1. x=1, y=-43, z=∞ 2. 01,341,11=-==z y x3. Clear fractions: 3, -4, 04. ()043Plane C 1. x=2, y=23, z=1 2. 11,321,211===z y x3. Clear fractions: 3, 4, 64. (346)6.(P82 3-23) Sketch the following planes and directions within a cubic unit cell:(a) [101] (b) [010] (c) [122] (d)[301] (e) [201] (f) [213](g) (011) (h) (102) (i) (002) (j) (130) (k) (212) (l) (321)7. Calculate the angle between [100] and [111] in Al.Solution: The crystal structure of Al is Fcc. We can calculate the angle between [100] and [111] as31111001101011''''''cos 222222222222=+++++⨯+⨯+⨯=+++++++=w v u w v u ww vv uu θ73.54=θ8. Use a calculation to verify that theatomic packing factor for the FCC structure is 0.74.Solution:In an FCC, there are four lattice points per cell: if there is one atom per lattice point, there are also four atoms per cell. Thevolume of one atom is 4πr 3/3 and thevolume of the unit cell is a 3:Packing factor = 4×4πr 3/3 a 3Since for FCC unit cell, a=4r/2, packingfactor =o.749. 写出溶解在γ-Fe 中碳原子所处的位置,若此类位置全部被碳原子占据,那么试问在这种情况下,γ-Fe 能溶解多少重量百分数的碳?而实际上在γ-Fe 中最大溶解度是多少?两者在数值上有差异的原因是什么?解答:γ-Fe 是fcc ,八面体间隙尺寸大,故C 的存在位置为八面体间隙。