合肥工业大学大学物理C期末考试题库

考核人数______ 考核班次_______________ 任课教员_________ 出题教员签名________ 任课教研室主任签名_______日期_______ 队别__________ 教学班次___________ 学号___________ 姓名____________…………………………密………………………………封………………………………线………………………………………太原理工大学《大学物理》期末考试试卷（C 卷)一、选择题（10小题，每小题3分，共30分）1、甲、乙二卡车在狭窄的公路上同向行驶，甲车以1sm 10-•速度匀速行驶，乙车在后.当乙车发现甲车时,车速为1ms 15-,相距m 1000。

为避免相撞，乙车立即作匀减速行驶,其加速度至少应为（ ）A 、 2ms 01250-. B 、 2ms 02500-. C 、 2ms 06250-.2、一质点沿半径为R 的圆周按规律221ct bt s -=运动,其中b 、c 是正的常量。

在切向加速度与法向加速度的大小第一次相等前，质点运动经历的时间为多少( ）。

A 、c b +c R B 、c R c b - C 、cR c b - 2 D 、cR cb+3、质量分别为1m 和2m 的两个物体迭放在光滑水平桌面上，如图所示。

若两物体间摩擦系数为μ，要它们不产生相对运动,那么推力最大不应超过多少( ) A 、μ1m g B 、 21(m m +μ）g C 、 g m m m m 2211）（+μD 、 g m m m m 2121+μ4、质量为10kg 的物体受一变力作用沿直线运动，力随位置变化如图所示。

若物体以1m ·s－1从原点出发，那么物体运动到16m 处的速率等于（ ）A 、22m ·s －1 B 、 3 m ·s －1 C 、 4 m ·s －1 D 、17 m ·s－15、三个相同的力分别作用在三根相同细杆的不同部位,如图所示。

2008-2009年 《大学物理C1》试卷A班级 学号 姓名一、单项选择题（本大题10小题，每题空2分，共20分）1．根据瞬时速度矢量v 的定义，在直角坐标系下，其大小||v可表示为（ ）(A)dr dt ； (B)dx dy dz dt dt dt++； (C)||||||dx dy dz i j k dt dt dt++； (D)222()()()dx dy dz dt dt dt++。

2. 有三辆质量均匀为m 的车厢a 、b 、c ，被车头牵引着在轨道上作无摩擦的直线运动。

若已知车厢a 受到的牵引力为F，则车厢b 受到的合力为（ ）(A) 0； (B) F ； (C) F/2； (D) F/33. 一子弹以水平速度0υ射入一静止于光滑水平面上的木块后，随木块一起运动。

对于这一过程正确的分析是( ) (A) 子弹、木块组成的系统机械能守恒； (B) 子弹、木块组成的系统水平方向的动量守恒； (C) 子弹所受的冲量等于木块所受的冲量； (D) 子弹动能的减少等于木块动能的增加。

4. 质量和长度都相同的均匀铝细圆棒a 和铁细圆棒b ，它们对穿过各自中心且垂直于棒的轴的转动惯量分别为a J 、b J ，那么（ ）（A ） a b J J >； （B ） a b J J <； （C ） a b J J =； （D ） 不能确定。

5. 一质点作匀速率圆周运动时，（ ）(A) 它的动量不变，对圆心的角动量也不变。

(B) 它的动量不变，对圆心的角动量不断改变。

(C) 它的动量不断改变，对圆心的角动量不变。

(D) 它的动量不断改变，对圆心的角动量也不断改变。

6 关于点电荷电场强度的计算公式304q E r rπε=，以下说法正确的是（ ） (A) 0r →时，E →∞；acFb(B) 0r →时，q 不能作为点电荷，公式不适用； (C) 0r →时，q 仍是点电荷，但公式无意义；(D) 0r →时，q 已成为球形电荷，应用球对称电荷分布来计算电场。

大学物理c的试题及答案一、选择题（每题2分，共20分）1. 下列哪个选项是牛顿第一定律的描述？A. 物体在没有外力作用下，总保持静止或匀速直线运动状态B. 物体的加速度与作用力成正比，与质量成反比C. 物体的加速度与作用力成正比，与质量成正比D. 物体在任何情况下都保持静止或匀速直线运动状态答案：A2. 光在真空中的传播速度是多少？A. 299,792,458 m/sB. 299,792,458 km/sC. 299,792,458 km/hD. 299,792,458 m/h答案：A3. 以下哪个是电场强度的定义？A. 电场力与电荷的比值B. 电荷与电场力的比值C. 电场力与电场强度的比值D. 电场强度与电荷的比值答案：A4. 根据热力学第一定律，系统内能的增加等于系统吸收的热量与对外做的功之和。

A. 正确B. 错误答案：A5. 电磁波的频率与波长的关系是？A. 频率与波长成正比B. 频率与波长成反比C. 频率与波长无关D. 频率与波长成正比，但只在特定条件下成立答案：B6. 根据麦克斯韦方程组，变化的磁场会产生什么？A. 变化的电场B. 恒定的电场C. 恒定的磁场D. 没有影响答案：A7. 欧姆定律描述的是电流、电压和电阻之间的关系，其表达式为？A. I = V/RB. I = R/VC. V = I * RD. R = V/I答案：A8. 以下哪个选项是描述波的干涉现象？A. 两个波相遇时，振幅相加B. 两个波相遇时，振幅相减C. 两个波相遇时，振幅不变D. 两个波相遇时，振幅消失答案：A9. 根据量子力学，电子在原子中的运动状态是由什么决定的？A. 电子的电荷B. 电子的质量C. 电子的能级D. 电子的动量答案：C10. 根据相对论，当物体的速度接近光速时，其质量会如何变化？A. 质量不变B. 质量增加C. 质量减少D. 质量消失答案：B二、填空题（每题2分，共20分）1. 根据牛顿第二定律，物体的加速度与作用力成_______，与物体的质量成_______。

1. S: 2kv dtdva -==2kv dxdvv dt dx dx dv -==k d x v dvxx vv -=⎰⎰)(ln00x x k v v--= )(00x x k e v v --= (answer)2. S: j t i t dt rd v )3cos 15()3sin 15(+-== jt i t dtv d a )3sin 45()3cos 45(-+-==()()j t i t j t i t v r)3cos 15()3sin 15()3sin 5()3cos 5(+-⋅+=⋅j j t t i i t t⋅⋅+⋅⋅-=)3c o s 3s i n 75()3sin 3cos 75( 0= (proved c)3. S: dtdv v m k m f a =-==dt mkv dv t t v v -=⎰⎰0)(0t mkv t v -=0)(ln t m ke v t v -=0)( (answer) D: t m k e v dtdxv -==0dt e v dx t m k tt x -⎰⎰=00)(0kmv x e kmv ekmv t x t m k t t mk 0max 00),1()(=-=-=--4. S: )()32(j y d i dx j i x r d f dw+⋅+=⋅=dy xdx dw w fi32+==⎰⎰dy xdx 323342⎰⎰--+== -6 J (answer)5. S: 23230.60.4)0.30.4(t t t t t dtddt d +-=+-==θω, t t t dtddt d 60.6)30.60.4(2+-=+-==ωα 0.40300.60.4)0(2=⨯+⨯-=ω (answer of a)0.28)0.4(30.40.60.4)0.4(2=⨯+⨯-=ω rad/s (answer of a ) 60.266)0.2(=⨯+-=α rad/s 2 (answer of b )t t 60.6)(+-=α is time varying not a constant (answer of c) 6. S: ω20031222ML L v m L mv +⋅= MLmv ML L mv 4343020==ω (answer a))c o s 1(2)31(21m a x 22θω-=LMg ML ]1631[cos 2221maxgLM v m -=-θ (answer b) 7. G: m =1.0g, M =0.50kg, L =0.60m, I rod =0.0602m kg ⋅,s rod /5.4=ωR:I sys , v 0S: I sys =I rod +(M+m)L2=0.060+(0.50+0.0010)×0.602= 0.24 2m kg ⋅(answer)the system ’s angular momentum about rotating axis is conservative in the collision.sysI L mv ω=0s m mL I v sys/108.160.00010.024.05.430⨯=⨯⨯==ω (answer )D: The bullet momentum 0v m p=(before impact), its angular momentumabout rotating axis can be expressed as L mv 0(a scalar) 8. S:γ==00.800x xt v c -∆==0811800.600 3.0010t t γ∆=∆=⨯⨯ 9. S: 202202)(mc E cp E E γγ==+=222c p m c m c m c =10. S: 0i n t =-=∆n e t n e t W Q E n e t n e t W Q = 1(3010)(4.0 1.0)2=-- J 30= (answer)11. S: from nRT PV =and K T A 300= we can get:KT K T C B 100300== (answer of a)Change of internal energy between A and B:0)(23int =-=∆A B T T k n E (answer of b)The net work of the cycle:))(100300()13(2121m N AC BC W ⋅-⋅-=⋅=J 200= (answer of c) From the first law : W E Q +∆=int we can derive:the net heat of the whole cycle is J W Q 200== (answer)12. S: 131)(320===⎰⎰∞F v Av dv Av dv v p F33FvA =(answer of a ) F F v a v g v Av dv vAv v F4341420===⎰13. G: T 1=T 2=T , m 1, p 1, v rms,1, m 2, p 2=2p 1, v avg,2 = 2v rms,1 R: m 1 / m 2 S: v avg,2 =1.602m kTv rms,1 = 1.731m kTv avg,2 = 2v rms,167.4)60.173.12(221=⨯=m m (answer) 14. S: dE int =dQ – dWd Q = dE int + dW = n C v dT+pdV VdVnR T dT nC dV T p T dT nC T dQ dS v v +=+==if i f v VV v T T V V nR T T nC V dVnR T dT nC ds S f i filnln +=+==∆⎰⎰⎰ 15. S: dA E q θεcos 0⎰=212100)0.60100(1085.8⨯-⨯⨯=- C 61054.3-⨯= 16. S: 2041)(r Qr E πε=(R < r <∞) dr rQ dr r E udV dU 2022208421πεπε=⋅== RQ r dr Q udV U R0220288πεπε===⎰⎰∞(answer) RQ r dr Q U r r Rεπεεπε02202*88==⎰∞(answer ) 18. S: in the shell of r – r + drdr r R r dV r dq 204)/1()(πρρ-==)34(31)/(4)(4303200r Rr dr R r r dq r q r-=-==⎰⎰πρπρfrom the shell theorems , within the spherical symmetry distribution )34(12)(41)(20020r Rr Rr r q r E -==ερπε (answer of b)R r r R Rdr dE 320)64(12*00=⇒=-=ερ 00200*max 9])32(3324[12)(ερερRR R R R r E E =-⨯== 19. S: j yV i x V V gradV y x E∂∂-∂∂-=-∇=-=),( )0.20.2(y x x VE x +-=∂∂-= x yV E y 0.2-=∂∂-= )/(480.2)0.20.2()0.2,0.2(m V j i j x i y x E--=-+-=20. S: Q in = - q , Q out = q (answer ) 1010241241)0(R qq V q πεπε==104)0(R qV in πε-=204)0(R q V o u t πε=)0()0()0()0(out in q V V V V ++= )11(4210R R q +=πε21. S: from the planar symmetry and superposition principle, Emust in normal direction of the plates and 1σ,2σ,3σ,4σ must be const. Fromcharge conservationA Q S =+)(21σσ ⇒ SQ A=+21σσ (1) B Q S =+)(43σσ ⇒ SQ B=+43σσ (2) Apply Gauss ’ law in the closed surface shown in Fig. 032=+σσ (3)within the metal, 0=p Ewhich leads to002222432104030201=-++⇒=-++σσσσεσεσεσεσFrom(1), (2), (3), (4) yield:⎪⎪⎩⎪⎪⎨⎧-=-=+==S Q Q SQ Q B AB A 223241σσσσ (answer of a) (6 points) 004030201122222εεσεσεσεσS Q Q E BA p -=--+= (1 point) 004030201222222εεσεσεσεσS Q Q E BA p +=+++=(1 point) (answer of b) d S Q Q d E d E V BA p AB 012ε-==⋅= (2 points) (answer of c)。

Exercise:1. A particle moving along x axis starts from x 0 with initial velocity v 0. Its acceleration can be expressed in a =-kv 2 where k is a known constant. Find its velocity function v =v (x ) with the coordinate x as variable.2. A particle moves in xy plane with the motion function asj t i t t r )3sin 5()3cos 5()(+=(all in SI). Find (a) its velocity )(t v and (b)acceleration )(t ain the unit-vector notation. (c) Show that v r ⊥. 3. A bullet of mass m is shot into a sand hill along a horizontal path, assume that the drag of the sand is kv f -=, find the velocity function v(t) if 0)0(v v = and the gravitation of the bullet can beignored.4. what work is done by a conservative force j i x f 32+= thatmoves a particle in xy plane from the initial position j i r i 32+= tothe final position j i r f 34--=. All quantities are in SI.5. The angular position of a point on the rim of a rotating wheel is given by 320.30.4t t t +-=θ, where θ is in radians and t is in seconds. Find (a) its angular velocities at t=0s and t =4.0s? (b) Calculate its angular acceleration at t =2.0s. (c) Is its angular acceleration constant?6. A uniform thin rod of mass M and length L can rotate freely about a horizontal axis passing through its top end o (231ML I =). Abullet of mass m penetrates the rod passing its center of mass when the rod is in vertical stationary. If the path of the bullet is horizontal with an initial speed v o before penetration and 20v after penetration . Show that (a) the angular velocity of the rod just after the penetration is MLmv 430=ω. (b) Find the maximum angular max θ the rod will swing upward after penetration.7. A 1.0g bullet is fired into a block (M=0.50kg) that is mounted on the end of a rod (L=0.60m). The rotational inertia of the rod alone about A is 206.0m kg ⋅. The block-rod-bullet system then rotates about a fixed axis at point A. Assume the block is small enough to treat as a particle on the end of the rod. Question: (a) What is the rotational inertia of the block-rod-bullet system about A? (b) If the angular speed of the system about A just after the bullet ’s impact is 4.5rad/s , What is the speed of the bullet just before the impact? γ between the rest frame S and the frame S* in which the clock is rest. (b) what time does the clock read as it passes x =180m ?9. What must be the momentum of a particle with mass m so that its total energy is 3 times rest energy?10. Ideal gas within a closed chamber undergoes the cycle shownthe net energy added to the gas as heat shown in the Fig. temperature at state A is 300K.(a). calculate the temperature of state B and C.(b). what is the change in internal energy of the gas between stateA and state B? (int E ∆)(c). the work done by the gas of the whole cycle .(d). the net heat added to the gas during one complete cycle.12. The motion of the electrons in metals is similar to the motion of molecules in the ideal gases. Its distribution function of speed is not Maxwell ’s curve but given by.⎩⎨⎧=0)(2Av v p the possible maximum speed v F is called Fermi speed. (a)。

大学物理C 思考题5-1-2气体在平衡状态时有何特征？这时气体中有分子热运动吗？热力学中的平衡与力学中的平衡有何不同？5-4-1对一定量的气体来说，当温度不变时，气体的压强随体积的减小而增大；当体积不变时，压强随温度的升高而增大。

就微观来看，他们有何区别？5-5-5如盛有气体的容器相对于某坐标系从静止开始运动，容器内的分子速度相对于这坐标系也将增大，则气体的温度会不会因此升高呢？5-5-6速率分布函数的物理意义是什么？试说明下列各量的意义：（1）dv v f )(；（2）dv v Nf )(；（3）⎰21)(v v dv v f ；（4）⎰21)(v v dv v Nf ；（5）⎰21)(v v dv v vf ；（6）⎰21)(v v dv v Nvf 。

5-7-2平均自由程与气体的状态以及分子本身的性质有何关系？在计算平均自由程时，什么地方体现了统计平均？5-0-1理想气体的微观模型？5-0-2能量均分定理及含义？6-2-1为什么气体热容的数值可以有无穷多个？什么情况下，气体的摩尔热容是零？什么情况下，气体的摩尔热容是无穷大？什么情况下是正值？什么情况下是负值？6-3-2有两个热气分别用不同的热源作卡诺循环，在V p -图上，它们的循环曲线所包围的面积相等，但形状不同，如图所示，它们吸热和放热的差值是否相同？对所作的净功是否相同？效率是否相同？6-6-2在日常生活中，经常遇到一些单方向的过程，如：（1）桌上热餐变凉；（2）无支持的物体自由下落；（3）木头或其他燃料的燃烧。

它们是否都与热力学第二定律有关？在这些过程中熵变是否存在？如果存在，则是增大还是减小？7-2-2根据点电荷的电场强度公式r e q E 201πε=，当所考察的场点和点电荷的距离0→r 时，电场强度∞→E ，这是没有物理意义的，对此似是而非的问题应如何解释？7-3-1如果在高斯面上的E处处为零，能否肯定此高斯面内一定没有净电荷？反过来，如果高斯面内没有净电荷，能否肯定面上所有各点的E 都等于零？7-5-1（1）已知电场中某点的电势，能否计算出该点的场强？（2）已知电场中某点附近的电势分布，能否计算出该点的场强？7-6-5一带点导体放在封闭的金属壳内部。

大学物理c下试题及答案一、选择题（每题2分，共20分）1. 下列关于光的偏振现象描述正确的是：A. 光的偏振只发生在自然光中B. 光的偏振只发生在偏振光中C. 光的偏振是光的电磁波性质的表现D. 光的偏振与光的传播方向无关答案：C2. 根据麦克斯韦方程组，下列说法错误的是：A. 变化的磁场会产生电场B. 恒定的电流不会产生磁场C. 变化的电场会产生磁场D. 恒定的电荷分布不会产生电场答案：D3. 在理想气体状态方程中，下列哪个物理量是温度的函数？A. 体积B. 压力C. 气体常数D. 摩尔质量答案：B4. 根据热力学第一定律，下列说法正确的是：A. 系统对外做功，内能一定增加B. 系统吸收热量，内能一定增加C. 系统对外做功且吸收热量，内能可能不变D. 系统对外做功且吸收热量，内能一定减少答案：C5. 根据量子力学的波粒二象性，下列说法正确的是：A. 光子具有波动性，电子不具有波动性B. 电子具有波动性，光子不具有波动性C. 光子和电子都具有波动性D. 光子和电子都不具有波动性答案：C6. 在电磁波谱中，波长最长的是：A. 无线电波B. 微波C. 红外线D. 可见光答案：A7. 根据狭义相对论，下列说法错误的是：A. 运动的物体长度会变短B. 运动的物体质量会增加C. 运动的物体时间会变慢D. 光速在所有惯性参考系中都是相同的答案：D8. 在电磁感应现象中，下列说法正确的是：A. 只有变化的磁场才能产生感应电动势B. 恒定的磁场也能产生感应电动势C. 变化的电场不能产生感应电动势D. 恒定的电场也能产生感应电动势答案：A9. 根据热力学第二定律，下列说法正确的是：A. 热量可以从低温物体自发地传递到高温物体B. 热量不能自发地从低温物体传递到高温物体C. 所有自然过程都是可逆的D. 所有自然过程都是不可逆的答案：B10. 在量子力学中，下列说法错误的是：A. 电子在原子中的运动是确定的B. 电子在原子中的运动是概率性的C. 电子的波动性与粒子性是不可分割的D. 电子的波动性与粒子性是相互独立的答案：A二、填空题（每题2分，共20分）1. 光的波长、频率和速度之间的关系是：波长= __________ × 频率。

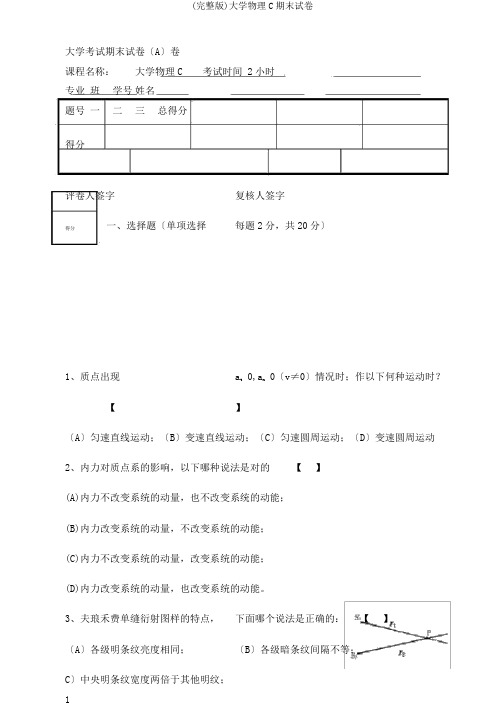
大学考试期末试卷〔A〕卷课程名称：大学物理C 考试时间 2小时专业班学号姓名题号一二三总得分得分评卷人签字复核人签字得分一、选择题〔单项选择每题2分，共20分〕1、质点出现a t 0,a n 0〔v≠0〕情况时；作以下何种运动时？【】〔A〕匀速直线运动；〔B〕变速直线运动；〔C〕匀速圆周运动；〔D〕变速圆周运动2、内力对质点系的影响，以下哪种说法是对的【】(A)内力不改变系统的动量，也不改变系统的动能；(B)内力改变系统的动量，不改变系统的动能；(C)内力不改变系统的动量，改变系统的动能；(D)内力改变系统的动量，也改变系统的动能。

3、夫琅禾费单缝衍射图样的特点，下面哪个说法是正确的：【】〔A〕各级明条纹亮度相同；〔B〕各级暗条纹间隔不等；C〕中央明条纹宽度两倍于其他明纹；D〕当用白光照射时，中央明纹两侧为由红到紫的彩色条纹.4、在同一媒质中两列相干的平面简谐波强度之比是I1:I24，那么两列波的振幅之比A1:A2为【】〔A〕4；〔B〕2；〔C〕16；〔D〕1/4。

5、在下面几种说法中，正确的选项是：【】〔A〕波源不移动位置时，波源的振动周期与波动的周期在数值上是不同的；〔B〕波源振动的速度与波速相同；〔C〕在波传播方向上，任一质点的振动相位总是比波源的相位滞后；〔D〕在波传播方向上，任一质点的振动相位总是比波源的相位超前。

6、图中S1和S2是两相干波源，其振动的运动方程分别y1A1cos(t 1)和y2A2cos(t 2)，其发出波长为λ的两列波在P点相遇.假设S1发出的波在P、S1两点间的相差位第1页〔共6页〕为1 2在P 点引起的两个振动的相位差为，那么二者的值分别为S 1P ；波源S 和S【】〔A 〕〔C 〕s1ps1p2r 1, 2r 1r 2；〔B 〕2r2,2 r 1r 2；〔D 〕sp2r 1,121sp2r2,1212r 1r2；2r 1r2。

7、在双缝干预实验中，假设单色光源S 到两缝S 1、S 2 距离相等，那么观察屏上中央明纹中心位于图中O 处，现将光源S 向下移动到示意图中的S 位置，那么【】〔A 〕中央明条纹向下移动，且条纹间距不变；〔B 〕中央明条纹向上移动，且条纹间距增大；SS 1O〔C 〕中央明条纹向下移动，且条纹间距增大；S 2S〔D 〕中央明条纹向上移动，且条纹间距不变。

1. S: 2kv dtdva -==2kv dxdvv dt dx dx dv -== kdx v dvxx vv -=⎰⎰)(ln00x x k v v--=(answer) )(00x x k e v v --=2. S: j t i t dt rd v )3cos 15()3sin 15(+-==j t i t dtv d a )3sin 45()3cos 45(-+-==()()j t i t j t i t v r)3cos 15()3sin 15()3sin 5()3cos 5(+-⋅+=⋅ j j t t i i t t⋅⋅+⋅⋅-=)3cos 3sin 75()3sin 3cos 75((proved c)0=3. S:dtdvv m k m f a =-==dt mkv dv t t v v -=⎰⎰0)(0(answer)t mk v t v -=0)(ln tm ke v t v -=0)(D: t m k e v dtdxv -==0dte v dx t m k tt x -⎰⎰=00)(0kmv x e kmv ekmv t x t m k t t mk 0max 00),1()(=-=-=--4. S:)()32(j y d i dx j i x r d f dw+⋅+=⋅=dy xdx dw w fi32+==⎰⎰= -6 J (answer)dy xdx 323342⎰⎰--+=5. S: ,23230.60.4)0.30.4(t t t t t dtddt d +-=+-==θω t t t dtddt d 60.6)30.60.4(2+-=+-==ωα (answer of a)0.40300.60.4)0(2=⨯+⨯-=ωrad/s (answer of a ) 0.28)0.4(30.40.60.4)0.4(2=⨯+⨯-=ω rad/s 2 (answer of b )60.266)0.2(=⨯+-=α is time varying not a constant (answer of c)t t 60.6)(+-=α6. S: ω20031222ML L v m L mv +⋅=(answer a)MLmv ML L mv 4343020==ω )cos 1(2)31(21max 22θω-=LMg ML (answer b)]1631[cos 2221maxgLM v m -=-θ7.G: m =1.0g, M =0.50kg,L =0.60m,I rod =0.060,2m kg ⋅srod /5.4=ωR:I sys , v 0S: I sys =I rod +(M+m)L 2=0.060+(0.50+0.0010)×0.602= 0.24 (answer)2mkg ⋅the system’s angular momentum about rotating axis is conservative in the collision.sys I L mv ω=0 (answer )s m mL I v sys/108.160.00010.024.05.430⨯=⨯⨯==ωD: The bullet momentum (before impact), its angular momentum0v m p=about rotating axis can be expressed as (a scalar)L mv 08. S:γ==00.800x xt v c -∆==0811800.600 3.0010t t γ∆=∆=⨯⨯9. S: 202202)(mc E cp E E γγ==+=222cp ===10. S: 0int =-=∆net net W Q E(answer)net net W Q =1(3010)(4.0 1.0)2=--J 30=11. S: from and we can get:nRT PV =K T A 300=(answer of a)KT K T C B 100300==Change of internal energy between A and B:(answer of b)0)(23int =-=∆A B T T k n E The net work of the cycle:))(100300()13(2121m N AC BC W ⋅-⋅-=⋅=(answer of c)J 200=From the first law : we can derive:W E Q +∆=int the net heat of the whole cycle is (answer)J W Q 200==12. S: 131)(320===⎰⎰∞F v Av dv Av dv v p F(answer of a )33Fv A =F F v avg v Av dv vAv v F4341420===⎰13. G: T 1=T 2=T , m 1, p 1, v rms,1, m 2, p 2=2p 1, v avg,2 = 2v rms,1 R: m 1 / m 2 S: v avg,2 =1.602m kTv rms,1 = 1.731m kTv avg,2 = 2v rms,1(answer) 67.4)60.173.12(221=⨯=m m 14. S: dE int =dQ – dWd Q = dE int + dW = n C v dT+pdV VdVnR T dT nC dV T p T dT nC T dQ dS v v +=+==i f i f v V V v T T V V nR T T nC V dVnR T dT nC ds S fifilnln +=+==∆⎰⎰⎰15. S:dA E q θεcos 0⎰=212100)0.60100(1085.8⨯-⨯⨯=-C 61054.3-⨯=16. S: (R < r <∞)2041)(r Qr E πε= dr rQ dr r E udV dU 2022208421πεπε=⋅== (answer)RQ r dr Q udV U R0220288πεπε===⎰⎰∞(answer )RQ r dr Q U r r Rεπεεπε02202*88==⎰∞18. S: in the shell of r – r + drdr r R r dV r dq 204)/1()(πρρ-==)34(31)/(4)(4303200r R r dr R r r dq r q r-=-==⎰⎰πρπρfrom the shell theorems , within the spherical symmetry distribution (answer of b) )34(12)(41)(20020r Rr Rr r q r E -==ερπεR r r R Rdr dE 320)64(12*00=⇒=-=ερ 00200*max 9]32(3324[12)(ερερRR R R R r E E =-⨯==19. S: j yV i x V V gradV y x E∂∂-∂∂-=-∇=-=),( )0.20.2(y x x VE x +-=∂∂-=x yV E y 0.2-=∂∂-=)/(480.2)0.20.2()0.2,0.2(m V j i j x i y x E--=-+-=20. S: Q in = - q , Q out = q (answer )1010241241)0(R qR q V q πεπε==104)0(R q V in πε-=204)0(R q V out πε=)0()0()0()0(out in q V V V V ++=)11(4210R R q +=πε21. S: from the planar symmetry and superposition principle, must in Enormal direction of the plates and ,,, must be const. From 1σ2σ3σ4σcharge conservation (1)A Q S =+)(21σσ⇒S Q A=+21σσ(2)B Q S =+)(43σσ⇒SQ B=+43σσ Apply Gauss’ law in the closed surface shown in Fig.(3)032=+σσ within the metal, which leads to 0=p E002222432104030201=-++⇒=-++σσσσεσεσεσεσFrom(1), (2), (3), (4) yield:(answer of a) (6 points)⎪⎪⎩⎪⎪⎨⎧-=-=+==S Q Q SQ Q B AB A 223241σσσσ (1 point)004030201122222εεσεσεσεσS Q Q E BA p -=--+= (1 point) (answer of b)004030201222222εεσεσεσεσS Q Q E BA p +=+++=(2 points) (answer of c)d S Q Q d E d E V BA p AB 012ε-==⋅=。

大学物理学专业《大学物理（下册）》期末考试试卷C卷含答案姓名：______ 班级：______ 学号：______考试须知：1、考试时间：120分钟，本卷满分为100分。

2、请首先按要求在试卷的指定位置填写您的姓名、班级、学号。

一、填空题（共10小题，每题2分，共20分）1、刚体绕定轴转动时，刚体的角加速度与它所受的合外力矩成______，与刚体本身的转动惯量成反比。

（填“正比”或“反比”）。

2、花样滑冰运动员绕通过自身的竖直轴转动，开始时两臂伸开，转动惯量为，角速度为；然后将两手臂合拢，使其转动惯量变为，则转动角速度变为_______。

3、如图所示，一静止的均匀细棒，长为、质量为，可绕通过棒的端点且垂直于棒长的光滑固定轴在水平面内转动，转动惯量为。

一质量为、速率为的子弹在水平面内沿与棒垂直的方向射出并穿出棒的自由端，设穿过棒后子弹的速率为，则此时棒的角速度应为______。

4、一束光线入射到单轴晶体后，成为两束光线，沿着不同方向折射．这样的现象称为双折射现象．其中一束折射光称为寻常光，它______________定律；另一束光线称为非常光，它___________定律。

5、一个半径为、面密度为的均匀带电圆盘，以角速度绕过圆心且垂直盘面的轴线旋转；今将其放入磁感应强度为的均匀外磁场中，的方向垂直于轴线。

在距盘心为处取一宽度为的圆环，则该带电圆环相当的电流为________，该电流所受磁力矩的大小为________ ，圆________盘所受合力矩的大小为________。

6、已知质点的运动方程为，式中r的单位为m，t的单位为s。

则质点的运动轨迹方程，由t=0到t=2s内质点的位移矢量______m。

7、两个同振动方向、同频率、振幅均为A的简谐振动合成后振幅仍为A，则两简谐振动的相位差为_______ 。

8、一圆盘正绕垂直于盘面的水平光滑固定轴O转动，如图射来两个质量相同，速度大小相同，方向相反并在一条直线上的子弹，子弹射入圆盘并留在盘内，则子弹射入后的瞬间，圆盘的角速度_____。

大学物理c试题及答案一、选择题（每题2分，共20分）1. 光在真空中的传播速度是（）。

A. 2.998×10^8 m/sB. 3.0×10^8 m/sC. 3.0×10^5 km/sD. 3.0×10^8 km/s2. 根据能量守恒定律，下列说法正确的是（）。

A. 能量可以在不同形式之间转换B. 能量可以在不同物体之间转移C. 能量的总量是恒定的D. 所有以上说法3. 牛顿第一定律也被称为（）。

A. 惯性定律B. 作用力与反作用力定律C. 万有引力定律D. 动量守恒定律4. 电磁波谱中，波长最长的是（）。

A. 无线电波B. 微波C. 红外线D. 可见光5. 以下哪个选项是描述电流的（）。

A. 电荷的流动B. 电荷的静止C. 电荷的累积D. 电荷的消散6. 根据热力学第二定律，下列说法正确的是（）。

A. 热量可以自发地从低温物体传递到高温物体B. 热量不能自发地从低温物体传递到高温物体C. 所有自发过程都是不可逆的D. 所有以上说法7. 根据库仑定律，两个电荷之间的力与它们之间的距离成（）。

A. 正比B. 反比C. 无关D. 指数关系8. 光的折射定律，即斯涅尔定律，表明入射角和折射角之间的关系是（）。

A. 入射角增大，折射角也增大B. 入射角增大，折射角减小C. 入射角和折射角成正比D. 入射角和折射角成反比9. 根据麦克斯韦方程组，变化的磁场会产生（）。

A. 电场B. 磁场C. 引力场D. 以上都不是10. 根据量子力学，一个粒子的波函数可以描述（）。

A. 粒子的位置B. 粒子的速度C. 粒子的动量D. 粒子的概率分布二、填空题（每题2分，共20分）1. 根据牛顿第二定律，力等于质量乘以_________。

2. 光的波长、频率和速度之间的关系可以用公式_________表示。

3. 一个物体的动能可以表示为_________，其中m是质量，v是速度。

4. 电流的单位是_________，符号为A。

大学数学专业《大学物理（二）》期末考试试题C卷附答案姓名：______ 班级：______ 学号：______考试须知：1、考试时间：120分钟，本卷满分为100分。

2、请首先按要求在试卷的指定位置填写您的姓名、班级、学号。

一、填空题（共10小题，每题2分，共20分）1、长为、质量为的均质杆可绕通过杆一端的水平光滑固定轴转动，转动惯量为，开始时杆竖直下垂，如图所示。

现有一质量为的子弹以水平速度射入杆上点，并嵌在杆中. ，则子弹射入后瞬间杆的角速度___________。

2、一圆锥摆摆长为I、摆锤质量为m，在水平面上作匀速圆周运动，摆线与铅直线夹角，则：(1) 摆线的张力T＝_____________________；(2) 摆锤的速率v＝_____________________。

3、一小球沿斜面向上作直线运动，其运动方程为：，则小球运动到最高点的时刻是=_______S。

4、动方程当t=常数时的物理意义是_____________________。

5、图示曲线为处于同一温度T时氦（原子量4）、氖（原子量20）和氩（原子量40）三种气体分子的速率分布曲线。

其中曲线（a）是________气分子的速率分布曲线；曲线（c）是________气分子的速率分布曲线。

6、动量定理的内容是__________，其数学表达式可写__________，动量守恒的条件是__________。

7、某一波长的X光经物质散射后，其散射光中包含波长________和波长________的两种成分，其中_________的散射成分称为康普顿散射。

8、刚体绕定轴转动时，刚体的角加速度与它所受的合外力矩成______，与刚体本身的转动惯量成反比。

（填“正比”或“反比”）。

9、四根辐条的金属轮子在均匀磁场中转动，转轴与平行，轮子和辐条都是导体，辐条长为R，轮子转速为n，则轮子中心O与轮边缘b之间的感应电动势为______________，电势最高点是在______________处。

大学物理C 思考题5-1-2气体在平衡状态时有何特征？这时气体中有分子热运动吗？热力学中的平衡与力学中的平衡有何不同？5-4-1对一定量的气体来说，当温度不变时，气体的压强随体积的减小而增大；当体积不变时，压强随温度的升高而增大。

就微观来看，他们有何区别？5-5-5如盛有气体的容器相对于某坐标系从静止开始运动，容器内的分子速度相对于这坐标系也将增大，则气体的温度会不会因此升高呢？5-5-6速率分布函数的物理意义是什么？试说明下列各量的意义：（1）dv v f )(；（2）dv v Nf )(；（3）⎰21)(v v dv v f ；（4）⎰21)(v v dv v Nf ；（5）⎰21)(v v dv v vf ；（6）⎰21)(v v dv v Nvf 。

5-7-2平均自由程与气体的状态以及分子本身的性质有何关系？在计算平均自由程时，什么地方体现了统计平均？5-0-1理想气体的微观模型？5-0-2能量均分定理及含义？6-2-1为什么气体热容的数值可以有无穷多个？什么情况下，气体的摩尔热容是零？什么情况下，气体的摩尔热容是无穷大？什么情况下是正值？什么情况下是负值？6-3-2有两个热气分别用不同的热源作卡诺循环，在V p -图上，它们的循环曲线所包围的面积相等，但形状不同，如图所示，它们吸热和放热的差值是否相同？对所作的净功是否相同？效率是否相同？6-6-2在日常生活中，经常遇到一些单方向的过程，如：（1）桌上热餐变凉；（2）无支持的物体自由下落；（3）木头或其他燃料的燃烧。

它们是否都与热力学第二定律有关？在这些过程中熵变是否存在？如果存在，则是增大还是减小？7-2-2根据点电荷的电场强度公式r e q E 201πε=，当所考察的场点和点电荷的距离0→r 时，电场强度∞→E ，这是没有物理意义的，对此似是而非的问题应如何解释？7-3-1如果在高斯面上的E处处为零，能否肯定此高斯面内一定没有净电荷？反过来，如果高斯面内没有净电荷，能否肯定面上所有各点的E 都等于零？7-5-1（1）已知电场中某点的电势，能否计算出该点的场强？（2）已知电场中某点附近的电势分布，能否计算出该点的场强？7-6-5一带点导体放在封闭的金属壳内部。

Exercise:1. A particle moving along x axis starts from x 0 with initial velocity v 0. Its acceleration can be expressed in a =-kv 2 where k is a known constant. Find its velocity function v =v (x ) with the coordinate x as variable.2. A particle moves in xy plane with the motion function asj t i t t r )3sin 5()3cos 5()(+=(all in SI). Find (a) its velocity )(t v and (b)acceleration )(t ain the unit-vector notation. (c) Show that v r ⊥. 3. A bullet of mass m is shot into a sand hill along a horizontal path, assume that the drag of the sand is kv f -=, find the velocity function v(t) if 0)0(v v = and the gravitation of the bullet can beignored.4. what work is done by a conservative force j i x f 32+= thatmoves a particle in xy plane from the initial position j i r i 32+= tothe final position j i r f 34--=. All quantities are in SI.5. The angular position of a point on the rim of a rotating wheel is given by 320.30.4t t t +-=θ, where θ is in radians and t is in seconds. Find (a) its angular velocities at t=0s and t =4.0s? (b) Calculate its angular acceleration at t =2.0s. (c) Is its angular acceleration constant?6. A uniform thin rod of mass M and length L can rotate freely about a horizontal axis passing through its top end o (231ML I =). Abullet of mass m penetrates the rod passing its center of mass when the rod is in vertical stationary. If the path of the bullet is horizontal with an initial speed v o before penetration and 20v after penetration . Show that (a) the angular velocity of the rod just after the penetration is MLmv 430=ω. (b) Find the maximum angular max θ the rod will swing upward after penetration.7. A 1.0g bullet is fired into a block (M=0.50kg) that is mounted on the end of a rod (L=0.60m). The rotational inertia of the rod alone about A is 206.0m kg ⋅. The block-rod-bullet system then rotates about a fixed axis at point A. Assume the block is small enough to treat as a particle on the end of the rod. Question: (a) What is the rotational inertia of the block-rod-bullet system about A? (b) If the angular speed of the system about A just after the bullet ’s impact is 4.5rad/s , What is the speed of the bullet just before the impact?8. A clock moves along the x axis at a speed of 0.800c and reads zero as it passes the origin. (a) Calculate the Lorentz factor γ between the rest frame S and the frame S* in which the clock is rest. (b) what time does the clock read as it passes x =180m ?9. What must be the momentum of a particle with mass m so that its total energy is 3 times rest energy?10. Ideal gas within a closed chamber undergoes the cycle shownthe Fig. Calculate Q net the net energy added to the gas as heat during one complete cycle.11. One mole of a monatomic ideal gas undergoes the cycle shown in the Fig. temperature at state A is 300K.(a). calculate the temperature of state B and C.(b). what is the change in internal energy of the gas between stateA and state B? (int E )(c). the work done by the gas of the whole cycle .(d). the net heat added to the gas during one complete cycle.12. The motion of the electrons in metals is similar to the motion of molecules in the ideal gases. Its distribution function of speed is not Maxwell ’s curve but given by.⎩⎨⎧=0)(2Av v pthe possible maximum speed v F is called Fermi speed. (a) plot the distribution curve qualitatively. (b) Express the coefficientA in terms of v F . (c) Find its average speed v avg .13. Two containers are at the same temperature. The first contains gas with pressure 1p , molecular mass 1m , and rmsspeed 1rms v . The second contains gas with pressure 12p , molecularmass 2m , and average speed 122rms avg v v =. Find the mass ratio21m m .14. In a quasi-static process of the ideal gas, dW =PdV and d E int =nC v dT . From the 1st law of thermodynamics show that the change of entropy i f v i fT T nC V V nR S ln ln +=∆ .Where n is the numberof moles, C v is the molar specific heat of the gas at constant volume, R is the ideal gas constant, (V i , T i ) and (V f , T f ) . are the initial and final volumes and temperatures respectively.15. It is found experimentally that the electric field in a certain region of Earth ’s atmosphere is directed vertically down. At an altitude of 300m the field is 60.0 N /C ; at an altitude of 200m , thefield is 100N /C . Find the net charge contained in a cube 100m on edge, with horizontal faces at altitudes of 200m and 300m . Neglect the curvature of Earth.16. An isolated sphere conductor of radius R with charge Q . (a) Find the energy U stored in the electric field in the vacuum outside the conductor. (b) If the space is filled with a uniform dielectrics of known r ε what is U * stored in the field outside the conductorthen?17. Charge is distributed uniformly throughout the volume of an infinitely long cylinder of radius R. (a) show that, at a distance r from the cylinder axis (r<R), r E 02ερ=, where ρis the volume charge density. (b) write the expression for E when r>R .18. A non-uniform but spherically symmetric distribution of charge has a volume density given as follow:⎩⎨⎧-=0)/1()(0R r r ρρwhere 0ρ is a positive constant, r is the distance to the symmetric center O and R is the radius of the charge distribution. Within the charge distribution (r < R ), show that (a) the charge contained in the co-center sphere of radius r is )34(31)(430r R r r q -=πρ, (b) Find the magnitude of electric field E (r ) within the charge (r < R ). (c) Find the maximum field E max =E (r *) and the value of r *.19. In some region of space, the electric potential is the following function of x,y and z: xy x V 22+=, where the potential is measured in volts and the distance in meter . Find the electric field at the point x=2m, y=2m . (express your answer in vector form)20. The Fig. shows a cross section of an isolated spherical metal shell of inner radius R 1 and outer radius R 2. A point charge q is located at a distance 21R from the center of the shell. If the shell is electrically neutral, (a) what are the induced charges (Q in , Q out ) on both surfaces of the shell? (b) Find the electric potential V(0) at the center O assume V (∞)=0.21. Two large metal plates of equal areaare parallel and closed to each other with charges Q A , Q B respectively. Ignore the fringing effects, find (a) the surface charge density on each side of both plates, (b) the electric field at p1, p 2 . (c) the electric potential A and B)。

2015-2016(二)大学物理C 练习题一 判断题1、 理想流体是指绝对不可压缩且完全没有黏性的气体和液体。

2、 液体的表面张力系数与液体中所含杂质无关。

3、 气体处于平衡态时，气体中每个分子的动能都相等。

4、 气体分别经过等压、等温和绝热膨胀了相同的体积，其中绝热过程对外做功最大。

5、 在双缝干涉的实验中，中央明纹的宽度是其它各级明纹宽度的两倍。

6、 空间某点的电势与试探电荷的大小无关，而电势能则与试探电荷的大小有关。

7、 不管磁场为何种形式，通过闭合曲面的磁通量始终为零。

8、 水平流管内稳定流动的理想流体，截面积大处流速小，流速大处压强小。

9、 在毛细管中，液面上升的高度仅与液体的性质和管的内径有关。

10、 在温度和压强相同的条件下，相同体积中含有的分子数是相等的，且与气体的种类有关。

11、 绝热线比等温线要陡一些的原因是因为绝热膨胀除了分子数密度减小外，温度也要降低。

12、 在光的偏振中，自然光和偏振光经过一个偏振片后光强都变为原来的一半。

13、 一个均匀带电的球面，在球面外产生的电场与电势与等量的点电荷放置在球心所产生的效果是一样的。

14、 通过闭合曲面的磁通量取决于曲面的形状和大小以及磁场在空间的分布。

二填空题1、若水的表面张力系数为37310N/m α-=⨯，则当半径为51.010m r -=⨯的许多小水滴融合成半径为31.010m R -=⨯的大水滴时，所释放出的表面能为 。

2、若氢气和氦气的压强、体积和温度均相等，则它们的内能之比2H He /E E = 。

3、一小型热电厂内，一台利用地热发电的热机工作于温度为227℃的地下热源和温度为27℃的地表之间，假定热机以卡诺循环的效率运行，并每小时能从地下热源获取111.810J ⨯的热量，则该热机的功率为 。

4、设有一定质量的氦气（He ），在如左图所示的 绝热过程中，外界对氦气所做的功为 。

5、一物体沿x 轴做简谐振动，振幅为0.12m ，周期为2s 。

实用文档大学期末考试试卷（A 卷）20 学年第二学期 考试科目： 大学物理Ｃ 考试类型：（闭卷） 考试时间： 120 分钟学号 姓名 年级专业物理学常数：18103-⋅⨯=s m c ，12310381--⋅⨯=K J .k ，1318-⋅⋅=K mol J .R ，2212010858/Nm C .ε-⨯=，693.02ln =注意:答案必须写在答题纸上,写在试题纸上的无效 一、填空题（每空2分，共30分）1. ____________是表征液体表面张力大小的特征量。

液体表面张力系数2. ____________提供了一个判断流动类型的标准。

雷诺数3.根据拉普拉斯公式，液膜很薄，半径为R ，表面张力系数为γ的球形肥皂泡内、外压强差=-外内p p ____________。

实用文档R4 4.图中为室温下理想气体分子速率分布曲线，)(p v f 表示速率在最概然速率p v 附近单位速率区间内的分子数占总分子数的百分比，那么当气体的温度降低时p v ____________、)(p v f ___________。

（填变小、变大）变小，变大5. 温度为T 时，mol 1刚性双原子分子理想气体的内能表达式为___________。

RT 25实用文档6. 理想气体的定压摩尔热容量和等体摩尔容量的关系为_______________。

R C C m V m p +=,,7. 当导体处于静电平衡状态时，其内部电场强度等于_______________。

08. 静电场的安培环路定理的数学表达式为_________________________。

⎰=⋅ldl E 09. 如右图所示为一个假象的球面，其中心有一个运动电荷，速度方向如图所 示，则图中所标注的三个球面上的点，哪个点具有最大的磁场__________。

B10. 一物体沿x 轴作简谐振动，振幅为m 12.0，周期为s 2，当0=t 时位移为m 06.0，且向x 轴正方向运动，则该简谐振动的初相位为___________。