南理工物理光学02-05

光学工程Optical Engineering（领域代码：085202）一、培养目标光学工程领域全日制工程硕士培养基础扎实、素质全面、工程实践能力强，并具有一定的创新能力的应用型、复合型高层次工程技术和工程管理人才。

具体要求为：1、在思想上应拥护党的基本路线和方针政策，热爱祖国，遵纪守法，具有良好的职业道德和敬业精神，具有科学严谨和求真务实的学习态度和工作作风。

2、在业务上应掌握光学工程领域较坚实的基础知识，宽广的专门知识，以及必要的管理知识；掌握解决光学工程领域工程问题的先进方法和现代技术手段；具有独立从事科学研究、项目开发、工程设计和工程管理的能力；能够承担解决光学工程领域及其相关技术中的工程实际问题。

3、掌握一门外国语，较熟练地查阅本领域的国内外科技资料和文献，了解本领域的技术现状和发展趋势。

二、学制和学分全日制工程硕士研究生实行为以两年半为主的弹性学制，原则上不超过五年。

工程硕士生学习计划总学分不得少于80学分，其中课程学习不少于34学分，专业实践15学分，论文选题开题1学分，学位论文30学分。

三、研究方向光学工程领域是一个口径宽、覆盖面广、知识与技术密集，覆盖光电子技术与光子学技术、光电信息技术与工程、光学仪器及技术等多个工程技术领域，是信息社会的支柱性工程领域。

本领域主要研究方向有：1、光电子技术与光子学技术2、光电信息技术与工程3、光学仪器及技术四、培养方式1、采用课程学习、实践教学与学位论文相结合的培养方式。

2、课程学习和实践教学实行学分制。

鼓励工程硕士研究生到合作单位进行专业实践，可采取集中实践和分段实践相结合的方式，实践教学原则上在半年以上。

五、课程设置课程学习总学分应不少于34学分，具体设置及要求详见工程领域课程设置表。

六、专业实践专业实践注重培养研究生了解光学工程领域现实技术水平及企业运作的管理方式，通过参加实际课题的研究或企事业的具体工作，培养研究生发现问题、解决工程技术问题及管理问题的能光学工程领域课程设置表力，提高专业素养及就业创业能力。

研途宝考研/ 2017南京理工光学工程考研资料与专业综合解析——研途宝专业名称、代码：光学工程[080300]专业所属门类、代码：工学（08）所属院系：电光学院光学工程专业介绍：光学工程是一门历史悠久而又年轻的学科。

它的发展表征着人类文明的进程。

它的理论基础--光学，作为物理学的主干学科经历了漫长而曲折的发展道路，铸造了几何光学、波动光学、量子光学及非线性光学，揭示了光的产生和传播的规律和与物质相互作用的关系。

在早期，主要是基于几何光学和波动光学拓宽人的视觉能力，建立了以望远镜、显微镜、照相机、光谱仪和干涉仪等为典型产品的光学仪器工业。

考试科目：[101] 思想政治理论[201] 英语一[301] 数学一819 光学工程820 光电基础845 普通物理B研究方向：01、光电探测与图像工程02、光电信息与混合图像处理03、精密光学测试理论与技术04、红外物理与红外工程05、光电系统设计理论与技术06、光电信号处理与数字视频技术07、光电子信息技术与系统08、光通信与光纤应用技术2017光学工程专业课考研参考书目：《应用光学》张以漠机械工业出版社修订版；《光电子器件》王君容、薛君南国防工业出版社第二版；《光电信号处理》何兆湘华中科技大学出版社第一版；《应用光学与光学设计基础》迟泽英、陈文建东南大学出版社第一版；2017光学工程考研专业课资料：《2017南京理工大学普通物理B考研复习精编》《2017南京理工大学普通物理B考研冲刺宝典》研途宝考研/《2017南理工普通物理B考研模拟五套卷与答案解析》《南京理工大学普通物理（B）2008-2011考研真题试卷（电子版）》专业课资料地址: /zykzl历年考研复试分数线：2014年总分：285，政治/外语：38；业务1/业务2：57；2015年总分：295，政治/外语：40；业务1/业务2：60；【17光学工程考研辅导】2017南京理工大学考研高端保录班2017南理工专业课考研无忧通关班2017南京理工大学专业课考研一对一班2017南理工专业课考研面授集训班南理工光学工程考研经验与技巧分享（15高分学长）又是一年考研时，回首往事，我可以理解通过初试，焦急的准备复试的学弟、学妹的心情，尤其是对于外校的考研生。

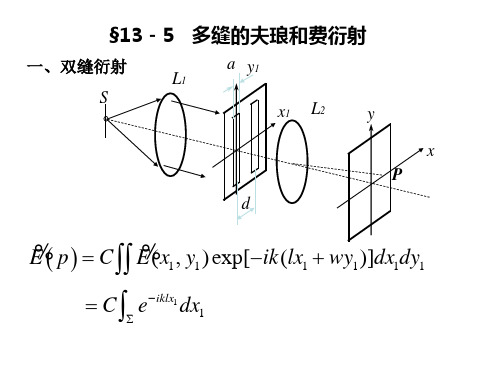
物理光学与应⽤光学习题解第⼆章概要第⼆章习题2-1. 如图所⽰，两相⼲平⾏光夹⾓为α，在垂直于⾓平分线的⽅位上放置⼀观察屏，试证明屏上的⼲涉亮条纹间的宽度为： 2 sin2αλ=l 。

2-2. 如图所⽰，两相⼲平⾯光波的传播⽅向与⼲涉场法线的夹⾓分别为0θ和R θ，试求⼲涉场上的⼲涉条纹间距。

2-3. 在杨⽒实验装置中，两⼩孔的间距为0.5mm ，光屏离⼩孔的距离为50cm 。

当以折射率为1.60的透明薄⽚贴住⼩孔S2时，发现屏上的条纹移动了1cm ，试确定该薄⽚的厚度。

2-4. 在双缝实验中，缝间距为0.45mm ，观察屏离缝115cm ，现⽤读数显微镜测得10个条纹（准确地说是11个亮纹或暗纹）之间的距离为15mm ，试求所⽤波长。

⽤⽩光实验时，⼲涉条纹有什么变化？2-5. ⼀波长为0.55m µ的绿光⼊射到间距为0.2mm 的双缝上，求离双缝2m 远处的观察屏上⼲涉条纹的间距。

若双缝距离增加到2mm ，条纹间距⼜是多少？2-6. 波长为0.40m µ～0.76m µ的可见光正⼊射在⼀块厚度为1.2×10-6 m 、折射率为1.5的薄玻璃⽚上，试问从玻璃⽚反射的光中哪些波长的光最强？2-7. 题图绘出了测量铝箔厚度D 的⼲涉装置结构。

两块薄玻璃板尺⼨为75mm ×25mm 。

在钠黄光（λ=0.5893m µ）照明下，从劈尖开始数出60个条纹（准确地说是从劈尖开始数出61个明条纹或暗条纹），相应的距离是30mm ，试求铝箔的厚度D = ？若改⽤绿光照明，从劈尖开始数出100个条纹，其间距离为46.6 mm ，试求这绿光的波长。

2-8. 如图所⽰的尖劈形薄膜，右端厚度h 为0.005cm ，折射率n = 1.5，波长为0.707m µ的光以30°⾓⼊射到上表2-1题⽤图2-2题⽤图2-7题⽤图2-8题⽤图⾯，求在这个⾯上产⽣的条纹数。

南京理工大学课程考试试卷（学生考试用）第 1 页共 1 页南京理工大学课程考试答案及评分标准南京理工大学课程考试试卷（学生考试用）6. Suppose we spread white light out into a fan of wavelengths by means of a diffraction grating and then pass a small select region of that spectrum out through a slit. Because of the slit, a band of wavelengths 1.2 nm wide centeredDetermine the frequency bandwidth and the coherence length of this light. (7. What is the general expression for the separation of the fringes of a Fresnel biprism of第 1 页共 1 页08A1. Given the wavefunctions 14sin 2(0.23)x t ψπ=-, and 2[sin(7 3.5)]/2.5x t ψ=+, determine in each case the values of (a) frequency, (b)wavelength, (c) period, (d)amplitude, (e)phase velocity, and (f) direction of motion. Time is in seconds and x is in meters. (12 points)2. Write an expression for the E -and B -fields that constitute a plane harmonic wave traveling in the +z-direction. The wave is linearly polarized with its plane of vibration at 450 to the yz -plane. (8 points)3. A 3.0-V flashlight bulb draws 0.25A, converting about 1.0% of the dissipated power into light(550nm λ≈). If the beam has a cross-sectional area of 10cm 2 and is approximately cylindrical, (a) How many photons are emitted per second? (b) How many photons occupy each meter of the beam? (c) What is the flux density of the beam as it leaves the flashlight? (346.62610h J s -=⨯⋅) (9 points)4. A ray of yellow light from a sodium discharge lamp falls on the surface of a diamond in air at 450. If at that frequency 2.42d n =, compute the angular deviation suffered upon transmission. (8 points)5. A beam of light in air strikes the surface of a smooth piece of plastic having an index of refraction of 1.55 at an angle with the normal of 20.00. The incident light has component E-field amplitudes parallel and perpendicular to the plane-of-incidence of 10.0V/m and 20.0V/m, respectively. Determine the corresponding reflected field amplitudes. (10 points)6. A magnetic-field technique for stabilizing a He-Ne laser to 2 parts in 1010 has been patented. At 632.8nm, what would be the coherence length of a laser with such a frequency stability? (8 points)7. An expanded beam of red light from a He-Ne laser (0632.8nm λ=) is incident on a screen containing two very narrow horizontal slits separated by 0.200mm. A fringe pattern appears on a white screen held 1.00m away. (a) How far (in radians and millimeters) above and below the central axis are the first zeros of irradiance? (b) How far (in mm) from the axis is the fifth bright band? (c) Compare these two results. (12 points)8. One of the mirrors of a Michelson Interferometer is moved, and 1000 fringe-pairs shift past the hairline in a viewing telescope during the process. If the device is illuminated with 500-nm light, how far was the mirror moved? (8 points)9. Suppose that we have a laser emitting a diffraction-limited beam (0632.8nm λ=) with a 2-mm diameter. How big a light spot would be produced on the surface of the Moon a distance of 337610km ⨯ away from such a device? Neglect any effects of the Earth ’s atmosphere. (7 points)10. Sunlight impinges on a transmission grating that is formed with 5000 lines per centimeter. Does the third-order spectrum overlap the second-order spectrum? Take red to be 780nm and violet to be 390 nm. (10 points)11. Imagine that we have randomly polarized room light incident almost normally on the glass surface of a radar screen. A portion of it would be specularly reflected back toward the viewer and would thus tend to obscure the display. Suppose now that we cover the screen with a right-circular polarizer, as shown in the Figure. Trace the incident and reflected beams, indicating their polarization states. What happens to the reflected beam? (8 points)08A答案07a1. Consider a lightwave having a phase velocity of 8310/m s ⨯ and a frequency of14610Hz ⨯. What is the shortest distance along the wave between any two points that have aphase difference of 300? What phase shift occurs at a given point in 10-6s, and how many waves have passed by in that time? (12 points)2. The electric field of an electromagnetic wave traveling in the positive x -direction is given by 00ˆE jsin()cos()E z z kx t πω=-, (a) Describe the field verbally. (b) Determine an expression for k . (c) Find the phase speed of the wave. (7 points)3. How many photons per second are emitted from a 100-W yellow lightbulb if we assume negligible thermal losses and a quasi-monochromatic wavelength of 550nm ? In actuality only about 2.5% of the total dissipated power emerges as visible radiation in an ordinary 100-W lamp. (346.62610h J s -=⨯⋅) (8 points)4. A laserbeam impinges on an air-liquid interface at an angle of 550. The refracted ray is observed to be transmitted at 400. What is the refractive index of the liquid? (7 points)5. Light is incident in air perpendicularly on a sheet of crown glass having an index of refraction of 1.522. Determine both the reflectance and the transmittance. (12 points)6. Imagine that we chop a continuous laserbeam (assumed to be monochromatic at0632.8nm λ=) into 0.1-ns pulses, using some sort of shutter. Compute the resultantlinewidth λ∆, bandwidth, and coherence length. Find the bandwidth and linewidth that would result if we could chop at 1015Hz . (8 points)7. With regard to Young ’s Experiment, derive a general expression for the shift in the vertical position of the m th maximum as a result of placing a thin parallel sheet of glass of index n and thickness d directly over one of the slits. Identify your assumptions. (10 points)8. Suppose we place a chamber 10.0cm long with flat parallel windows in one arm of a Michelson Interferometer that is being illuminated by 600-nm light. If the refractive index of air is 1.00029 and all the air is pumped out of the cell, how many fringe-pairs will shift by in the process? (10 points)9. If you peered through a 0.75-mm hole at an eye chart, you would probably notice a decrease in visual acuity. Compute the angular limit of resolution, assuming that it ’s determined only by diffraction; take 0550nm λ=. Compare your results with the value of41.710rad -⨯, which corresponds to a 4.0-mm pupil. (10 points)10. Light having a frequency of 144.010Hz ⨯ is incident on a grating formed with 10000 lines per centimeter. What is the highestorder spectrum that can be seen with this device? Explain. (8 points)11. A Babinet compensator is positioned at 450 between crossed linear polarizers and is being illuminated with sodium light. When a thin sheet of mica (indices 1.599 and 1.594) is placed on the compensator, the black bands all shift by 1/4 of the space separating them. Compute the retardance of the sheet and its thickness. (8 points)1. Solution:814/310/5100.6c m λνμ==⨯⨯= 83/310/60510c km λν==⨯=⨯2. Solution:The number of waves is 0/AB λ. With the glass in place, there are 0()/AB L λ- waves in vacuum and an additional /L λwaves in glass for a total of 00(/)(1/1/)AB L λλλ-. The difference in number is 0(1/1/)L λλ-, giving a phase shift of φ∆ of 2π for each wave; hence , 0002(1/1/)2(/1/)2/22000L L n L πλλπλλπλπ-=-==.3. Solution:(a) The phase angle is retarded by an amount (2/)2/n y y πλπλ∆-∆ or (1)/n y c ω-∆. Thus0exp [(1)//]p E E i t n y c y c ω=--∆- or 0exp[(1)/]exp (/)p E E i n y c i t y c ωω=--∆- (b) Since 1x e x ≈+ for small x, if 1n ≈ of 1y ∆ , exp[(1)/]1(1)/i n y c i n y c ωω--∆≈--∆ and since exp(/2)i i π-=-, (1)(/)exp(/2)p u u E E n y E c i ωπ=+-∆-4. Solution:/t i t i r n n n n -+ . Air-water: 4/311/70.144/31r -===+. Air-crown glass:3/211/50.203/21r -===+.More reflectance for glass. 2/r i I I R r ==.Air-water: 2(1/7)0.02R ==. Air-crown glass: 2(1/5)0.04R ==5. Solution:/21sin sin it ti n n θθθθ==/2/1sin sin t i n n θθ=/21sin sin t i n n θθ= and /t i i θθ=__/cos AB d t =θ ___/)sin(AB a t i =-θθ t t i daθθθcos )sin(=- a d t t i =-θθθcos )sin(6. Solution:99//(1.210)/(50010)0.0024m m ννλλ--∆=∆=⨯⨯=c νλ=, so 8914/(310/)/(50010) 6.0010c m s m Hz νλ-==⨯⨯=⨯1412(0.0024)(6.0010) 1.4410Hz ν∆=⨯=⨯131/ 6.9410c t s ν-∆∆=⨯8134(310/)(6.9410) 2.0810c c l c t m s s m --∆∆=⨯⨯=⨯7. Solution:)(2//0n n d s y -=∆αλ8.Solution ：λ=nd 2m nd 71084.12-⨯==λ9. Solution:θαsin 2k a =，θβsin 2k b=，mb a =，πβαm m 2==N=number of fringes=m m a 2/2/==πππ10. Solution:sin m a m θλ=sin /m m Y R θ6(/)10,000/10/m Y m a R lines cm lines m λ=== So 610a m -=761(589.5923)[1(5.89592310)/10](1.00)0.5895923Y nm m m m m--=⨯='761(588.9953)[1(5.88955310)/10](1.00)0.5889953Y nm m m m m--=⨯='411 5.9710Separation Y Y m -=-=⨯11. Solutionsin /sin i t ti n θθ=; sin sin /sin(40)/1.5t i ti n θθ== ; 25.4t θ= .2222tan ()/tan ()tan (14.6)/tan (65.4)0.014i t i t R θθθθ=-+=-=2222sin ()/sin ()sin (14.6)/sin (65.4)0.077i t i t R θθθθ⊥=-+=-=1()0.04552R R R ⊥=+= /()()/()67%p p n V I I I R R R R R ⊥⊥=+=+++=。