函数模型及其应用教案
- 格式:doc
- 大小:854.50 KB
- 文档页数:4

《函数模型的应用实例》一、教学内容分析:本节课选自人民教育出版社A版的普通高中课程标准实验教科书·数学必修1中3.2.2函数模型的应用实例(第二课时).函数基本模型的应用是本章的重点内容之一,函数模型本身就来源于现实,并用于解决实际问题.本节课的内容是在《几类不同增长的函数模型》和《函数模型的应用实例(一)》内容之后,对于纯数学知识的几类函数及其性质和给定的函数模型应用有了一定的学习,本节课是对以上两节内容的延续与拓展,研究没有给定函数模型或没有确定性函数模型的实际问题进行建模和应用.这节课的内容继续通过一些实例来感受函数模型的建立和应用,逐步体会实际问题中构建函数模型的过程,本节课的函数模型的应用实例主要包括建立确定性函数模型解决问题及选择或建立拟合函数模型解决问题.例5所给的问题的特点是表中数学的变化是有特定规律的,运用表中的数据规律建立数学模型,注意变化范围和检验结果的合理性,同时使用这种有规律的简单数据实例提供了建立数学模型的方法.例6与例5有所区别,表中数据的变化规律特点不是和明显,需要自己根据对数据的理解选择模型,这反映一个较为完整的建立函数模型解决问题的过程,让学生逐步感受和明确这一点.整节课要求学生分析数据,比较各个函数模型的优劣,选择接近实际的函数模型,并应用函数模型解决实际问题.强化读图、读表能力;优化学生思维,提高学生探究和解决问题的能力;强化学生数学应用意识,感受数学的实用性;锻炼学生的吃苦精神,提高学生的团队合作能力.二、教学目标:知识与技能:1.会分析所给出数据,画出散点图.2.会利用选择或建立的函数模型.3.会运用函数模型解决实际问题.过程与方法:1.通过对给出的数据的分析,抽象出相应的确定性函数模型,并验证函数模型的合理性.2.通过收集到的数据作出散点图,并通过观察图像判断问题所适用的函数模型,在合理选择部分数据或计算机的拟合功能得出具体的满意的函数解析式,并应用模型解决实际问题.情感、态度和价值观:1.经历建立函数模型解决实际问题的过程,领悟数学源自生活,服务生活,体会数学的应用价值.2.培养学生的应用意识、创新意识和探索精神,优化学生的理性思维和求真务实的科学态度.3.提高学生探究学习新知识的兴趣,培养学生,勇于探索的科学态度.三、学生学情分析:1.已掌握了一些基本初等函数的相关知识,有相应的数学基础知识储备.2.在前面的学习中,初步体会了利用给定函数模型解决实际问题的经历,为本节课积累解决问题的经验.3.学生从文字语言向图像语言和符号语言转化较弱;应用意识和应用能力不强;抽象概括和局部处理能力薄弱.四、教学重点、难点重点:根据收集的数据作出散点图,并通过观察图像选择问题所适用的函数模型,利用演算或计算机数据建立具体的函数解析式.难点:怎样合理分析数据选择函数模型和建立具体的函数解析式.五、教学策略分析:基于新课程标准倡导以学生为主体进行探究性学习,教师应成为学生学习的引导者、组织者和合作者的教学理念和最近发展区理论,结合本节课的教学目标,采用如下教学方法:1.问题教学法.在例1的教学中,提出如何能更为直观的发现函数模型,引导学生思考,发现选择函数模型的重要方法,即散点图图像,从而让学生有收获,有成就感.在例2的解决过程中,提出一系列的问题串,学会对问题的剖析,直达问题的核心.使学生的学习过程成为在教师引导下的“再创造”过程,并使学生从中体会学习的兴趣.这样可以充分调动学生学习的主动性、积极性,使课堂气氛更加活跃,同时培养了学生自主学习,动手探究的能力.2.分组讨论法.在例2的教学中,遇到难以选择模型时,通过小组讨论,拓展思维,加强合作,解决问题;在获得函数模型后和课堂总结中,组织小组讨论,相互交流成果,扩大成果影响力.这样不仅能够培养学生对数学知识的探索精神和团队协作精神,更能让学生体验成功的乐趣,培养其学习的主动性.3.多媒体辅助教学法:在教学过程中,采用多媒体教学工具,通过动态演示有利于引起学生的学习兴趣,激发学生的学习热情,增大信息的容量,使内容充实、形象、直观,提高教学效率和教学质量。
基于课程标准的学科教学设计义,能根据所给信息确定一次函数表达式.4.能画一次函数的图象,理解一次函数图象的变化情况,并利用一次函数图象解决简单的实际问题.5.在画一次函数的图象、探索一次函数图象的变化情况、利用一次函数的图象解决实际问题等过程,体会数形结合的思想方法与一次函数中k与b的实际意义.3.单元整体教学思路(教学结构图)课时教学设计课题《一次函数》第一课时课型新授课☑章/单元复习课□专题复习课□习题/试卷讲评课□学科实践活动课□其它1.课程标准分析1.体验从具体情境中抽象出数学符号的过程,理解函数的概念;探索具体问题中的数量关系和变化规律,掌握用函数进行表述的方法.2.通过用函数表述数量关系的过程,体会建模思想,建立符号意识;能独立思考,体会数学的基本思想和思维方式.6.学习活动设计教师活动学生活动环节一:创设情境、导入新课教的活动1播放洋葱数学有关函数的数学史。
学的活动1观看洋葱数学有关函数的数学史。
活动意图说明:承接上一学期变量关系的学习,让学生感受到变量之间关系的是通过多种形式表现出来的,感受研究函数的必要性。
环节二:展现背景,提供概念抽象的素材教的活动1问题 1.你去过游乐园吗?你坐过摩天轮吗?你能描述一下坐摩天轮的感觉吗?当人坐在摩天轮上时,人的高度随时间在变化,那么变化有规律吗?摩天轮上一点的高度h与旋转时间t之间有一定的关系,右图就反映了时间t(分)与摩天轮上一点的高度h(米)之间的关系.你能从上图观察出,有几个变化的量吗?当t分别取3,6,10时,相应的h是多少?给定一个t值,你都能找到相应的h值吗?问题2.在平整的路面上,某型号汽车紧急刹车后仍将滑行S米,一般地有经验公式2300vs ,其中v表示刹车前汽车的速度(单位:千米/时).(1)公式中有几个变化的量?计算当v分别为50,60,100时,相应的滑行距离s是多少?学的活动1畅所欲言,分享体验。
举手回答:摩天轮上一点的高度h与旋转时间t之间的关系。

第五章函数的应用(二)4.5.3 函数模型的应用本节课选自《普通高中课程标准实验教科书数学必修1本(A版)》的第五章的4.5.3函数模型的应用。
函数模型及其应用是中学重要内容之一,又是数学与生活实践相互衔接的枢纽,特别在应用意识日益加深的今天,函数模型的应用实质是揭示了客观世界中量的相互依存有互有制约的关系,因而函数模型的应用举例有着不可替代的重要位置,又有重要的现实意义。
本节课要求学生利用给定的函数模型或建立函数模型解决实际问题,并对给定的函数模型进行简单的分析评价,发展学生数学建模、数学直观、数学抽象、逻辑推理的核心素养。
课程目标学科素养1. 能建立函数模型解决实际问题.2.了解拟合函数模型并解决实际问题.3.通过本节内容的学习,使学生认识函数模型的作用,提高学生数学建模,数据分析的能力.a.数学抽象:由实际问题建立函数模型;b.逻辑推理:选择合适的函数模型;c.数学运算:运用函数模型解决实际问题;d.直观想象:运用函数图像分析问题;e.数学建模:由实际问题建立函模型;f.数据分析:通过数据分析对应的函数模型;教学重点:利用给定的函数模型或建立确定性函数模型解决实际问题.教学难点:利用给定的函数模型或建立确定性函数模型解决实际问题,并对给定的函数模型进行简单的分析评价.多媒体教学过程设计意图核心教学素养目标(一)创设问题情境1.常见函数模型常用函数模型(1)一次函数模型y=kx+b(k,b为常数,k≠0)(2)二次函数模拟y=ax2+bx+c(a,b,c为常数,a≠0)(3)指数函数模型y=ba x+c(a,b,c为常数,b≠0,a>0且a≠1) (4)对数函数模型y=m log a x+n(m,a,n为常数,m≠0,a>0且a≠1)(5)幂函数模型y=ax n+b(a,b为常数,a≠0)2.建立函数模型解决问题的基本过程(二)问题探究我们知道,函数是描述客观世界变化规律的数学模型,不同的变化规律需要用不同的函数模型来刻画.面临一个实际问题,该如何选择恰当的函数模型来刻画它呢?通过对常见函数模型的回顾,提出新的问题,提出运用函数模型分析解决实际问题,培养和发展数据分析、数学建模和数学抽象、直观想象的核心素养。

芯衣州星海市涌泉学校函数模型及其应用教学目的:1.能根据图形、表格等实际问题的情境建立数学模型,并求解;进一步理解函数模型在解决简单的实际问题中的应用,理解函数模型在社会生活中的广泛应用2.在解决实际问题的过程中,培养学生数学地分析问题、探究问题、解决问题的才能,培养学生的应用意识,进步学习数学的兴趣. 教学重点:在解决以图、表等形式作为问题背景的实际问题中,读懂图表并求解. 教学难点: 对图、表的理解. 教学方法: 讲授法,尝试法. 教学过程: 一、情境创设矩形的长为4,宽为3,假设长增加x ,宽减少0.5x ,所得新矩形的面积为S . 〔1〕将S 表示成x 的函数;〔2〕求面积S 的最大值,并求此时x 的值. 二、学生活动 考虑并完成上述问题. 三、例题解析例1有一块半径为R 的半圆形钢板,方案剪裁成等腰梯形ABCD 的形状,它的下底AB 是⊙O 的直径,上底CD 的端点在圆周上,写出这个梯形周长y 和腰长x 间的函数关系式,并求出它的定义域.A BO C DE例2一家旅社有100间一样的客房,经过一段时间是是的经营理论,旅社经理发现每间客房每天的价格与住房率有如下关系:要使每天收入最高,每间客房定价为多少元?例3今年5月,荔枝上.由历年的场行情得知,从5月10日起的60天内,荔枝的场售价与上时间是是的关系大致可用如下列图的折线ABCD表示(场售价的单位为元/500g).请写出场售价S(t)(元)与上时间是是t(天)的函数关系式,并求出6月20日当天的荔枝场售价.练习:1.直角梯形OABC中,AB∥OC,AB=1,OC=BC=2,直线l:x=t截此梯形所得位于l左方图形的f(t)的大致图象为()状可能是()元一个销售,每天可卖200个.假设这种商品每涨价1元,〔2〕假设销售价必须为整数,要使利润最大,应如何定价?5.根据场调查,某商品在最近40天内的价格f(t)与时间是是t满足:l AC DBhH A B C DO 10 40 60f(t)=111(020)241(2040)t t t Nt t t N⎧+<∈⎪⎨⎪-+∈⎩≤,≤≤,,销售量g(t)与时间是是t满足:g(t)=14333t-+(0≤t≤40,t N),求这种商品日销售金额的最大值.四、小结利用图、表建模;分段建模.五、作业。

函数模型及其应用教案一、教学目标1. 理解函数的概念,了解函数模型的产生和应用;2. 学习两种常见函数模型的基本形式和参数,并能解决实际问题应用;3. 认识函数模型在现实生活和工程实践中的重要作用;4. 提高学生分析和解决实际问题的能力。
二、教学重点1. 函数的概念与应用;2. 两种常见函数模型的基本形式与参数;3. 实际问题中函数模型的应用。
三、教学难点1. 函数模型在数学联系与实际应用展示之间的联系;2. 如何将实际问题转化为基本形式的函数模型。
四、教学方法1. 讲授教学法;2. 课堂互动式教学法;3. 问题式教学法。
五、教学准备1. 多媒体教学设备;2. 函数模型案例资料。
六、教学过程1. 引入函数是一种重要的数学概念,也是自然科学、经济学、工程技术等领域的基础。
而函数模型则是在实际问题中应用函数的过程中,通过对数据和经验的分析产生的数学模型,可用于预测、控制、优化等目的。
今天我们将学习两种常见函数模型及其应用。
2. 基础知识讲解(1)函数的概念函数是一个输入输出关系的特殊情况。
数学上定义一个函数是指一组数对,其中第一个数(称为自变量)从一个特定集合中取任意一个值,;第二个数(称为因变量或函数值)则从另一集合中取一个值,这个取值完全由第一个数决定。
(2)线性函数模型线性函数模型可以写为 y=a*x+b 的形式,其中 a 称为斜率,b称为截距。
它的应用非常广泛,比如经济学中的供给函数、消费函数,工程学中的动力学方程等等,都可以通过线性函数模型来描述。
(3)指数函数模型指数函数模型可以用 y=a^x+b 的形式表示,其中 a 称为底数,b 称为位移。
指数函数具有非常广泛的应用,在物理学、天文学、化学、生物学、经济学等领域中都有其用途,比如放射性衰变过程、细胞增殖过程、经济增长过程等等都可以使用指数函数模型来描述。
3. 练习将下列实际问题转化为线性函数模型或指数函数模型,并求出相应的参数或曲线。
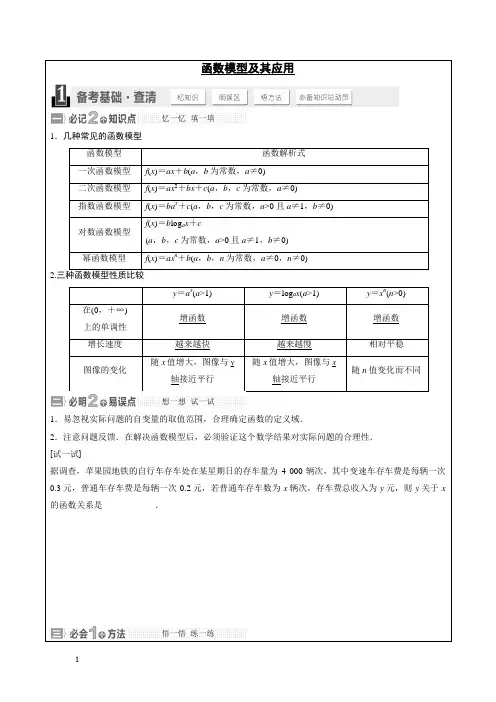
函数模型及其应用1.几种常见的函数模型函数模型函数解析式一次函数模型f(x)=ax+b(a,b为常数,a≠0)二次函数模型f(x)=ax2+bx+c(a,b,c为常数,a≠0)指数函数模型f(x)=ba x+c(a,b,c为常数,a>0且a≠1,b≠0)对数函数模型f(x)=b log a x+c(a,b,c为常数,a>0且a≠1,b≠0)幂函数模型f(x)=ax n+b(a,b,n为常数,a≠0,n≠0)2.三种函数模型性质比较y=a x(a>1)y=log a x(a>1)y=x n(n>0) 在(0,+∞)上的单调性增函数增函数增函数增长速度越来越快越来越慢相对平稳图像的变化随x值增大,图像与y轴接近平行随x值增大,图像与x轴接近平行随n值变化而不同1.易忽视实际问题的自变量的取值范围,合理确定函数的定义域.2.注意问题反馈.在解决函数模型后,必须验证这个数学结果对实际问题的合理性.[试一试]据调查,苹果园地铁的自行车存车处在某星期日的存车量为4 000辆次,其中变速车存车费是每辆一次0.3元,普通车存车费是每辆一次0.2元,若普通车存车数为x辆次,存车费总收入为y元,则y关于x 的函数关系是____________.解决实际应用问题的一般步骤(1)审题:弄清题意,分清条件和结论,理顺数量关系,初步选择数学模型;(2)建模:将自然语言转化为数学语言,将文字语言转化为符号语言,利用数学知识,建立相应的数学模型;(3)求模:求解数学模型,得出数学结论; (4)还原:将数学问题还原为实际问题. 以上过程用框图表示如下:[练一练]如图,已知正方形ABCD 的边长为1,过正方形中心O 的直线MN 分别交正方形的边AB ,CD 于点M ,N ,则当MNBN 取最小值时,CN =________.考点一一次函数与二次函数模型1.某电信公司推出两种手机收费方式:A 种方式是月租20元,B 种方式是月租0元.一个月的本地网内通话时间t (分钟)与电话费s (元)的函数关系如图所示,当通话150分钟时,这两种方式电话费相差________元.2.将进货单价为80元的商品按90元出售时,能卖出400个.若该商品每个涨价1元,其销售量就减(1)二次函数的最值一般利用配方法与函数的单调性解决,但一定要密切注意函数的定义域,否则极易出错;(2)确定一次函数模型时,一般是借助两个点来确定,常用待定系数法;(3)解决函数应用问题时,最后要还原到实际问题.考点二分段函数模型[典例]提高过江大桥的车辆通行能力可改善整个城市的交通状况.在一般情况下,大桥上的车流速度v(单位:千米/小时)是车流密度x(单位:辆/千米)的函数.当桥上的车流密度达到200辆/千米时,造成堵塞,此时车流速度为0千米/小时;当车流密度不超过20辆/千米时,车流速度为60千米/小时.研究表明:当20≤x≤200时,车流速度v是车流密度x的一次函数.(1)当0≤x≤200时,求函数v(x)的表达式.(2)当车流密度x为多大时,车流量(单位时间内通过桥上某观测点的车辆数,单位:辆/小时)f(x)=x·v(x)可以达到最大,并求出最大值(精确到1辆/小时).[类题通法]应用分段函数模型的关注点(1)实际问题中有些变量间的关系不能用同一个关系式给出,而是由几个不同的关系式构成,如出租车票价与路程之间的关系,应构建分段函数模型求解.(2)构造分段函数时,要力求准确、简洁,做到分段合理、不重不漏.(3)分段函数的最值是各段的最大(最小)者的最大者(最小者).[针对训练]某公司研制出了一种新产品,试制了一批样品分别在国内和国外上市销售,并且价格根据销售情况不断进行调整,结果40天内全部销完.公司对销售及销售利润进行了调研,结果如图所示,其中图①(一条折线)、图②(一条抛物线段)分别是国外和国内市场的日销售量与上市时间的关系,图③是每件样品的销售利润与上市时间的关系.(1)分别写出国外市场的日销售量f(t)与上市时间t的关系及国内市场的日销售量g(t)与上市时间t的关系;(2)国外和国内的日销售利润之和有没有可能恰好等于6 300万元?若有,请说明是上市后的第几天;若没有,请说明理由.考点三指数函数模型[典例] 一片森林原来面积为a ,计划每年砍伐一些树,且每年砍伐面积的百分比相等,当砍伐到面积的一半时,所用时间是10年,为保护生态环境,森林面积至少要保留原面积的14,已知到今年为止,森林剩余面积为原来的22. (1)求每年砍伐面积的百分比;(2)到今年为止,该森林已砍伐了多少年? (3)今后最多还能砍伐多少年?[类题通法]应用指数函数模型应注意的问题(1)指数函数模型,常与增长率相结合进行考查,在实际问题中有人口增长、银行利率、细胞分裂等增长问题可以利用指数函数模型来解决.[课堂练通考点]1.(2014·南昌质检)往外埠投寄平信,每封信不超过20 g,付邮费0.80元,超过20 g而不超过40 g,付邮费1.60元,依此类推,每增加20 g需增加邮费0.80元(信的质量在100 g以内).如果某人所寄一封信的质量为72.5 g,则他应付邮费________元.2.(2013·南通调研)甲地与乙地相距250 km.某天小袁从上午7:50由甲地开车前往乙地办事.在上午9:00,10:00,11:00三个时刻,车上的导航仪都提示“如果按出发到现在的平均速度继续行驶,那么还有 1 h到达乙地”.假设导航仪提示语都是正确的,那么在上午11:00时,小袁距乙地还有________km.3.一种产品的成本原为a元,在今后的m年内,计划使成本平均每年比上一年降低p%,成本y是关于经过年数x(0<x≤m)的函数,其关系式y=f(x)可写成_____________________.[课下提升考能]第Ⅰ卷:夯基保分卷1.(2014·苏锡常镇一调)某市出租车收费标准如下:起步价为8元,起步里程为3 km(不超过3 km按起步价付费);超过3 km但不超过8 km时,超过部分按每千米2.15元收费;超过8 km时,超过部分按每千米2.85元收费,另每次乘坐需付燃油附加费1元.现某人乘坐一次出租车付费22.6元,则此次出租车行驶了________ km.2.某大楼共有12层,有11人在第1层上了电梯,他们分别要去第2至第12层,每层1人.因特殊原因,电梯只允许停1次,只可使1人如愿到达,其余10人都要步行到达所去的楼层.假设乘客每向下步行1层的“不满意度”增量为1,每向上步行1层的“不满意度”增量为2,10人的“不满意度”之和记为S.则S最小时,电梯所停的楼层是________层.3.一高为H,满缸水量为V的鱼缸截面如图所示,其底部破了一个小洞,满缸水从洞中流出.若鱼缸水深为h时的水的体积为v,则函数v=f(h)的大致图像可能是图中的________.4.如图,书的一页的面积为600 cm2,设计要求书面上方空出2 cm的边,下、左、右方都空出1 cm的边,为使中间文字部分的面积最大,这页书的长、宽应分别为________.5.某商家一月份至五月份累计销售额达3 860万元,预测六月份销售额为500万元,七月份销售额比六月份递增x%,八月份销售额比七月份递增x%,九、十月份销售总额与七、八月份销售总额相等.若一月份至十月份销售总额至少达7 000万元,则x的最小值是________.6.(2014·连云港模拟)某单位决定对本单位职工实行年医疗费用报销制度,拟制定年医疗总费用在2万元至10万元(包括2万元和10万元)的报销方案,该方案要求同时具备下列三个条件:①报销的医疗费用y(万元)随医疗总费用x(万元)增加而增加;②报销的医疗费用不得低于医疗总费用的50%;③报销的医疗费用不得超过8万元.(1)请你分析该单位能否采用函数模型y=0.05(x2+4x+8)作为报销方案;(2)若该单位决定采用函数模型y=x-2ln x+a(a为常数)作为报销方案,请你确定整数a的值(参考数据:ln 2≈0.69,ln 10≈2.3).2.(2014·苏州一调)如图,有一块边长为1(百米)的正方形区域ABCD.在点A处有一个可转动的探照灯,其照射角∠P AQ始终为45°(其中点P,Q分别在边BC,CD上),设∠P AB=θ,tan θ=t.(1)用t表示出PQ的长度,并探求△CPQ的周长l是否为定值;(2)问探照灯照射在正方形ABCD内部区域的面积S至多为多少平方百米?3.(2013·徐州调研)徐州、苏州两地相距500 km,一辆货车从徐州匀速行驶到苏州,规定速度不得超过在海岸线上建一度假村P,不考虑风向等因素影响,油井对度假村废气污染程度与排出废气的浓度成正比(比例系数都为k1),与距离的平方成反比(比例系数都为k2),又知甲油井排出的废气浓度是乙油井的8倍.(1)设乙油井排出的废气浓度为a(a为常数),度假村P距离甲油井x km,度假村P受到甲、乙两油井的污染程度和记为f(x),求f(x)的解析式并求其定义域;(2)度假村P距离甲油井多少时,甲、乙两油井对度假村的废气污染程度和最小?。
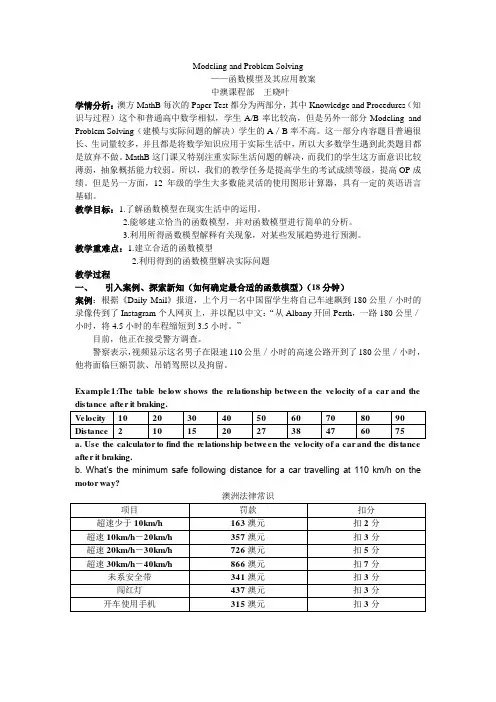
Modeling and Problem Solving——函数模型及其应用教案中澳课程部王晓叶学情分析:澳方MathB每次的Paper Test都分为两部分,其中Knowledge and Procedures(知识与过程)这个和普通高中数学相似,学生A/B率比较高,但是另外一部分Modeling and Problem Solving(建模与实际问题的解决)学生的A/B率不高。
这一部分内容题目普遍很长、生词量较多,并且都是将数学知识应用于实际生活中,所以大多数学生遇到此类题目都是放弃不做。
MathB这门课又特别注重实际生活问题的解决,而我们的学生这方面意识比较薄弱,抽象概括能力较弱。
所以,我们的教学任务是提高学生的考试成绩等级,提高OP成绩。
但是另一方面,12年级的学生大多数能灵活的使用图形计算器,具有一定的英语语言基础。
教学目标:1.了解函数模型在现实生活中的运用。
2.能够建立恰当的函数模型,并对函数模型进行简单的分析。
3.利用所得函数模型解释有关现象,对某些发展趋势进行预测。
教学重难点:1.建立合适的函数模型2.利用得到的函数模型解决实际问题教学过程一、引入案例、探索新知(如何确定最合适的函数模型)(18分钟)案例:根据《Daily Mail》报道,上个月一名中国留学生将自己车速飙到180公里/小时的录像传到了Instagram个人网页上,并以配以中文:“从Albany开回Perth,一路180公里/小时,将4.5小时的车程缩短到3.5小时。
”目前,他正在接受警方调查。
警察表示,视频显示这名男子在限速110公里/小时的高速公路开到了180公里/小时,他将面临巨额罚款、吊销驾照以及拘留。
Example1:The table below shows the relationship between the velocity of a car and the distance after it braking.Velocity 10 20 30 40 50 60 70 80 90 Distance 2 10 15 20 27 38 47 60 75a. Use the calculator to find the relationship between the velocity of a car and the distance after it braking.b. What’s the minimum safe following distance for a car travelling at 110 km/h on the motor way?澳洲法律常识项目罚款扣分超速少于10km/h 163澳元扣2分超速10km/h-20km/h 357澳元扣3分超速20km/h-30km/h 726澳元扣5分超速30km/h-40km/h 866澳元扣7分未系安全带341澳元扣3分闯红灯437澳元扣3分开车使用手机315澳元扣3分(设计意图:从生活案例引入新知,激发学生的学习兴趣。

函数模型及其应用的教学教案教学教案:函数模型及其应用一、教学目标1.了解函数模型的基本概念和特性;2.掌握函数模型在实际问题中的应用;3.培养学生的数学建模能力和问题解决能力。
二、教学重点和难点1.函数模型的基本概念和特性;2.函数模型在实际问题中的应用。
三、教学方法1.讲授与示范相结合;2.小组合作学习;3.课堂实践。
四、教学过程步骤一:导入新知识(10分钟)1.复习函数的基本概念和性质;2.提出问题:“函数模型是什么?它有什么特点?”;3.学生回答问题并进行讨论。
步骤二:讲解函数模型的基本概念(20分钟)1.介绍函数模型的定义和表示方法;2.引导学生理解函数模型的含义:根据已知条件,建立函数模型来描述一个实际问题;3.示范几个常见的函数模型。
步骤三:探究函数模型的特性(20分钟)1.引入函数模型的性质:单调性、奇偶性、周期性等;2.以实例为例,让学生观察并总结函数模型的特性;3.学生合作完成几个练习题。
步骤四:应用函数模型解决实际问题(30分钟)1.通过实例介绍函数模型在实际问题中的应用,如物体自由落体、物种数量增长等;2.让学生进行小组合作,选择一个实际问题,建立相应的函数模型并解决问题;3.学生展示他们的解决方案,进行评价和讨论。
步骤五:巩固与拓展(20分钟)1.让学生复习巩固所学的内容,完成一篇小结;2.引导学生思考:函数模型在其他学科中的应用;3.教师进行点评和总结。
五、教学评估1.课堂表现评价:学生是否积极参与讨论、是否能熟练运用函数模型解决实际问题等;2.书面作业评价:布置相关练习题,检查学生的掌握程度。
六、教学资源1.教材:《数学教材》;2.多媒体教学工具;3.实际问题的资料。
七、教学反思通过本节课的教学,学生能够理解函数模型的基本概念和特性,能够应用函数模型解决实际问题。
在教学过程中,我注重将知识与实际问题相结合,让学生能够在解决问题的过程中感受到函数模型的重要性和应用价值。

教学过程一、课堂导入有一大群喝水、嬉戏的兔子,但是这群兔子曾使澳大利亚伤透了脑筋.1859年,有人从欧洲带进澳洲几只兔子,由于澳洲有茂盛的牧草,而且没有兔子的天敌,兔子数量不断增加,不到100年,兔子们占领了整个澳大利亚,数量达到75亿只.可爱的兔子变得可恶起来,75亿只兔子吃掉了相当于75亿只羊所吃的牧草,草原的载畜率大大降低,而牛羊是澳大利亚的主要牲口.这使澳大利亚头痛不已,他们采用各种方法消灭这些兔子,直至二十世纪五十年代,科学家采用载液瘤病毒杀死了百分之九十的野兔,澳大利亚人才算松了一口气二、复习预习1.方程的根与函数零点有什么关系,函数零点的如何判断?2.用二分法求函数零点时需要注意些什么?3.涵数与方程的关系三、知识讲解考点1 几种常见的函数模型考点2 三种函数模型性质比较[探究] 1.直线上升、指数增长、对数增长的增长特点是什么?提示:直线上升:匀速增长,其增长量固定不变;指数增长:先慢后快,其增长量成倍增加,常用“指数爆炸”来形容;对数增长:先快后慢,其增长速度缓慢.四、例题精析【例题1】【题干】一水池有两个进水口,一个出水口,每个水口的进、出水速度如图甲、乙所示.某天0点到6点,该水池的蓄水量如图丙所示.给出以下3个论断:①0点到3点只进水不出水;②3点到4点不进水只出水;③4点到6点不进水不出水,则一定正确的是()A.①B.①②C.①③D.①②③【答案】A【解析】由甲、乙两图知,进水速度是出水速度的12,所以0点到3点不出水,3点到4点也可能一个进水口进水,一个出水口出水,但总蓄水量降低,4点到6点也可能两个进水口进水,一个出水口出水,一定正确的是①.【例题2】【题干】某商品在近30天内每件的销售价格p (元)与时间t (天)的函数关系式是p =⎩⎨⎧t +20,0<t <25,t ∈N ,-t +100,25≤t ≤30,t ∈N *,且该商品的日销售量Q (件)与时间t (天)的函数关系式是Q =-t +40(0<t ≤30,t ∈N ).求这种商品的日销售金额的最大值,并指出日销售金额最大的一天是30天中的第几天?【解析】设日销售金额为y (元),则y =p ·Q ,即y =⎩⎨⎧-t 2+20t +800,0<t <25,t ∈N ,t 2-140t +4 000,25≤t ≤30,t ∈N ,=⎩⎨⎧-(t -10)2+900,0<t <25,t ∈N , ①(t -70)2-900,25≤t ≤30,t ∈N . ②由①知,当t =10时,y max =900; 由②知,当t =25时,y max =1 125. 由1 125>900,知y max =1 125, 即在第25天日销售额最大,为1 125元.【例题3】【题干】某市居民自来水收费标准如下:每户每月用水不超过4吨时,每吨为1.80元,当用水超过4吨时,超过部分每吨3.00元.某月甲、乙两户共交水费y元,已知甲、乙两户该月用水量分别为5x,3x(吨).(1)求y关于x的函数;(2)若甲、乙两户该月共交水费26.4元,分别求出甲、乙两户该月的用水量和水费.【解析】(1)当甲的用水量不超过4吨时,即5x ≤4,乙的用水量也不超过4吨,y =1.8(5x +3x )=14.4x ;当甲的用水量超过4吨,乙的用水量不超过4吨,即3x ≤4,且5x >4时,y =4×1.8+3x ×1.8+3(5x -4)=20.4x -4.8. 当乙的用水量超过4吨,即3x >4时,y =2×4×1.8+3×[(3x -4)+(5x -4)]=24x -9.6.所以y =⎩⎪⎨⎪⎧ 14.4x , 0≤x ≤45,20.4x -4.8, 45<x ≤43,24x -9.6, x >43.(2)由于y =f (x )在各段区间上均单调递增,当x ∈⎣⎢⎡⎦⎥⎤0,45时,y ≤f ⎝ ⎛⎭⎪⎫45<26.4;当x ∈⎝ ⎛⎦⎥⎤45,43时,y ≤f ⎝ ⎛⎭⎪⎫43<26.4;当x ∈⎝ ⎛⎭⎪⎫43,+∞时,令24x -9.6=26.4,解得x =1.5. 所以甲户用水量为5x =5×1.5=7.5吨,付费S 1=4×1.8+3.5×3=17.70(元);乙户用水量为3x =4.5吨,付费S 2=4×1.8+0.5×3=8.70(元).【例题4】【题干】(2011·山东高考)(本小题满分12分)某企业拟建造如图所示的容器(不计厚度,长度单位:米),其中容器的中间为圆柱形,左右两端均为半球形,按照设计要求容器的容积为80π3立方米,且l≥2r.假设该容器的建造费用仅与其表面积有关.已知圆柱形部分每平方米建造费用为3千元,半球形部分每平方米建造费用为c(c>3)千元.设该容器的建造费用为y千元.(1)写出y关于r的函数表达式,并求该函数的定义域;(2)求该容器的建造费用最小时的r.【解析】(1)设容器的容积为V ,由题意知V =4πr 33+πr 2l ,又V =80π3,⇨(1分) 所以4πr 33+πr 2l =80π3,解得l =803r 2-4r 3,⇨(2分)由于l ≥2r ,因此0<r ≤2.⇨(3分),所以圆柱的侧面积为2πrl =2πr ⎝ ⎛⎭⎪⎫803r 2-4r 3=160π3r -8πr 23, 两端两个半球的表面积之和为4πr 2,所以建造费用y =160πr -8πr 2+4πcr 2,定义域为(0,2].⇨(4分)(2)由(1),得y ′=-160πr 2-16πr +8πcr =c -r 2·⎝ ⎛⎭⎪⎫r 3-20c -2,0<r ≤2,⇨(5分) 由于c >3,所以c -2>0.当r 3-20c -2=0时,r = 320c -2.令 320c -2=m ,则m >0. 所以y ′=8π(c -2)r2 (r -m )(r 2+rm +m 2).⇨(7分) ①当0<m <2,即c >92时,当r =m 时,y ′=0;当r ∈(0,m )时,y ′<0;当r ∈(m,2)时,y ′>0,所以r =m 是函数y 的极小值点,也是最小值点.⇨(9分)②当m≥2,即3<c≤92时,当r∈(0,2)时,y′<0,函数单调递减,所以r=2是函数y的最小值点.⇨(11分)综上,当3<c≤92时,建造费用最小时r=2;当c>92时,建造费最小时r=320c-2.⇨(12分)五、课堂运用【基础】1.如图是张大爷晨练时所走的离家距离(y)与行走时间(x)之间的函数关系图,若用黑点表示张大爷家的位置,则张大爷散步行走的路线可能是()解析:选C由于中间一段时间,张大爷离家的距离不变,故应选C.2.某地2011年底人口为500万,人均住房面积为6 m2,如果该城市人口平均每年增长率为1%.问为使2021年底该城市人均住房面积增加到7 m2,平均每年新增住房面积至少为(1.0110≈1.104 6)()A.90万m2B.87万m2C.85万m2D.80万m2500×(1+1%)10×7-500×610≈86.6(万m 2)≈87(万m2).解析:选B由题意3.如图所示,点P在边长为1的正方形的边上运动,设M是CD边的中点,则当点P沿着A-B-C-M运动时,以点P经过的路程x为自变量,将三角形APM的面积y看作路程x的函数,则其函数图象大致是()解析:选A 当0≤x ≤1时,y =12·x ·1=12x ; 当1<x ≤2时,y =1-12(x -1)-14(2-x )-14=-14x +34; 当2<x ≤2.5时,y =12⎝ ⎛⎭⎪⎫52-x ×1=54-12x . 则y =⎩⎪⎨⎪⎧ 12x ,0≤x ≤1,-14x +34,1<x ≤2,-12x +54,2<x ≤2.5.根据函数可以画出其大致图象,故选A.【巩固】4.一高为H,满缸水量为V的鱼缸截面如图所示,其底部破了一个小洞,满缸水从洞中流出.若鱼缸水深为h时的水的体积为v,则函数v=f(h)的大致图象可能是图中的________.解析:当h=0时,v=0可排除①、③;由于鱼缸中间粗两头细,∴当h在H2附近时,体积变化较快;h小于H2时,增加越来越快;h大于H2时,增加越来越慢.答案:②5.某商场宣传在节假日对顾客购物实行一定的优惠,商场规定:①如一次购物不超过200元,不予以折扣;②如一次购物超过200元,但不超过500元,按标价予以九折优惠;③如一次购物超过500元的,其中500元给予九折优惠,超过500元的给予八五折优惠;某人两次去购物,分别付款176元和432元,如果他只去一次购买同样的商品,则应付款________元.解析:由题意知付款432元,实际标价为432×109=480元,如果一次购买标价176+480=656元的商品应付款500×0.9+156×0.85=582.6元.答案:582.6【拔高】6.A,B两城相距100 km,在两城之间距A城x(km)处建一核电站给A,B两城供电,为保证城市安全,核电站距城市距离不得小于10 km.已知供电费用等于供电距离(km)的平方与供电量(亿度)之积的0.25倍,若A城供电量为每月20亿度,B城为每月10亿度.(1)求x的取值范围;(2)把月供电总费用y表示成x的函数;(3)核电站建在距A城多远,才能使供电总费用y最少?解:(1)x的取值范围为[10,90].(2)y=5x2+52(100-x)2(10≤x≤90).(3)由y=5x2+52(100-x)2=152x2-500x+25 000=152⎝⎛⎭⎪⎫x-10032+50 0003,得x=1003时,y min=50 0003,即核电站建在距A城1003km处,能使供电总费用y最少.7.目前某县有100万人,经过x年后为y万人.如果年平均增长率是1.2%,请回答下列问题:(1)写出y关于x的函数解析式;(2)计算10年后该县的人口总数(精确到0.1万人);(3)计算大约多少年后该县的人口总数将达到120万(精确到1年).解:(1)当x =1时,y =100+100×1.2%=100(1+1.2%);当x =2时,y =100(1+1.2%)+100(1+1.2%)×1.2%=100(1+1.2%)2;当x =3时,y =100(1+1.2%)2+100(1+1.2%)2×1.2%=100(1+1.2%)3;…故y 关于x 的函数解析式为y =100(1+1.2%)x (x ∈N *).(2)当x =10时,y =100×(1+1.2%)10=100×1.01210≈112.7.故10年后该县约有112.7万人.(3)设x 年后该县的人口总数为120万,即100×(1+1.2%)x =120,解得x =log 1.012120100≈15.3故大约16年后该县的人口总数将达到120万.8.据气象中心观察和预测:发生于M地的沙尘暴一直向正南方向移动,其移动速度v(km/h)与时间t(h)的函数图象如图所示,过线段OC上一点T(t,0)作横轴的垂线l,梯形OABC在直线l左侧部分的面积即为t(h)内沙尘暴所经过的路程s(km).(1)当t=4时,求s的值;(2)将s随t变化的规律用数学关系式表示出来;(3)若N城位于M地正南方向,且距M地650 km,试判断这场沙尘暴是否会侵袭到N城.如果会,在沙尘暴发生后多长时间它将侵袭到N城?如果不会,请说明理由.解:(1)由图象可知:当t =4时,v =3×4=12,∴s =12×4×12=24.(2)当0≤t ≤10时,s =12·t ·3t =32t 2;当10<t ≤20时,s =12×10×30+30(t -10)=30t -150;当20<t ≤35时,s =12×10×30+10×30+(t -20)×30-12×(t -20)×2(t -20)=-t 2+70t -550. 综上可知,s =⎩⎪⎨⎪⎧ 32t 2, t ∈[0,10],30t -150, t ∈(10,20],-t 2+70t -550, t ∈(20,35].(3)∵t ∈[0,10]时,s max =32×102=150<650,t ∈(10,20]时,s max =30×20-150=450<650,∴当t ∈(20,35]时,令-t 2+70t -550=650,解得t 1=30,t 2=40.∵20<t ≤35,∴t =30,即沙尘暴发生30 h 后将侵袭到N 城.课程小结常见函数模型的理解(1)直线模型:即一次函数模型,其增长特点是直线上升(x的系数k>0),通过图像可以很直观地认识它.(2)指数函数模型:能用指数型函数表达的函数模型,其增长特点是随着自变量的增大,函数值增大的速度越来越快(a>1),常形象地称为“指数爆炸”.注意:指数函数y=a x(a>1),从图像上看,在开始过程中增长缓慢,但随着x的逐渐增大,当x增加一个非常小的增量Δx,其函数值变化Δy会大得惊人,因此常称之为“指数爆炸”.(3)对数函数模型:能用对数函数表达式表达的函数模型,其增长的特点是开始阶段增长的较快(a>1),但随着x的逐渐增大,其函数值变化越来越慢,常称之为“蜗牛式增长”.(4)幂函数型函数模型:能用幂函数表达的函数模型,其增长情况随x n中n的取值变化而定,常用的有二次函数模型.(5)“对勾”函数模型,形如f(x)=x+ax(a>0,x>0)的函数模型,在现实生活中也有着广泛的应用,常利用“基本不等式”解决,有时利用函数的单调性求解最值.31 / 31。

第十节函数模型及其应用一、复习目标:1.了解指数函数、对数函数以及幂函数的增长特征。
知道直线上升、指数增长、对数增长等不同函数类型增长的含义。
2.了解函数模型(如指数函数、对数函数、幂函数、分段函数等在社会生活中普遍使用的函数模型)的广泛应用。
3.能利用给定的函数模型解决简单的实际问题。
二、重难点:重点:掌握一次函数、二次函数、指数函数、对数函数等基本初等函数模型;培养阅读理解、建立数学模型和分析问题、解决问题的能力掌握解函数应用问题的基本步骤。
难点:建立数学模型和分析问题、解决问题的能力的培养。
三、教学方法:讲练结合,探析归纳。
四、教学过程(一)、谈新课标要求及考纲要求和高考命题考查情况,促使学生积极参与。
新课标要求及考纲要求:1.利用计算工具,比较指数函数、对数函数以及幂函数增长差异;结合实例体会直线上升、指数爆炸、对数增长等不同函数类型增长的含义;2.收集一些社会生活中普遍使用的函数模型(指数函数、对数函数、幂函数、分段函数等)的实例,了解函数模型的广泛应用。
高考命题考查情况及预测:函数应用问题是高考的热点,高考对应用题的考查即考小题又考大题,而且分值呈上升的趋势。
高考中重视对环境保护及数学课外的的综合性应用题等的考查。
出于“立意”和创设情景的需要,函数试题设置问题的角度和方式也不断创新,重视函数思想的考查,加大函数应用题、探索题、开放题和信息题的考查力度,从而使高考考题显得新颖、生动和灵活。
预测2010年的高考,将再现其独特的考查作用,而函数类应用题,是考查的重点,因而要认真准备应用题型、探索型和综合题型,加大训练力度,重视关于函数的数学建模问题,学会用数学和方法寻求规律找出解题策略。
(1)题型多以大题出现,以实际问题为背景,通过解决数学问题的过程,解释问题;(2)题目涉及的函数多以基本初等函数为载体,通过它们的性质(单调性、极值和最值等)来解释生活现象,主要涉计经济、环保、能源、健康等社会现象。
《函数的概念及其表⽰》教案完美版《函数的概念及其表⽰》教案第⼀课时: 1.2.1 函数的概念(⼀)教学要求:通过丰富实例,进⼀步体会函数是描述变量之间的依赖关系的重要数学模型,在此基础上学习⽤集合与对应的语⾔来刻画函数,体会对应关系在刻画函数概念中的作⽤;了解构成函数的要素;能够正确使⽤“区间”的符号表⽰某些集合。
教学重点、难点:理解函数的模型化思想,⽤集合与对应的语⾔来刻画函数。
教学过程:⼀、复习准备:1. 讨论:放学后骑⾃⾏车回家,在此实例中存在哪些变量?变量之间有什么关系?2 .回顾初中函数的定义:在⼀个变化过程中,有两个变量x 和y ,对于x 的每⼀个确定的值,y 都有唯⼀的值与之对应,此时y 是x 的函数,x 是⾃变量,y 是因变量. 表⽰⽅法有:解析法、列表法、图象法.⼆、讲授新课:1.教学函数模型思想及函数概念:①给出三个实例:A .⼀枚炮弹发射,经26秒后落地击中⽬标,射⾼为845⽶,且炮弹距地⾯⾼度h (⽶)与时间t (秒)的变化规律是21305h t t =-.B .近⼏⼗年,⼤⽓层中臭氧迅速减少,因⽽出现臭氧层空洞问题,图中曲线是南极上空臭氧层空洞⾯积的变化情况.(见书P16页图)C .国际上常⽤恩格尔系数(⾷物⽀出⾦额÷总⽀出⾦额)反映⼀个国家⼈民⽣活质量的⾼低。
“⼋五”计划以来我们城镇居民的恩格尔系数如下表. (见书P17页表)②讨论:以上三个实例存在哪些变量?变量的变化范围分别是什么?两个变量之间存在着这样的对应关系?三个实例有什么共同点?归纳:三个实例变量之间的关系都可以描述为,对于数集A 中的每⼀个x ,按照某种对应关系f ,在数集B 中都与唯⼀确定的y 和它对应,记作::f A B →③定义:设A 、B 是⾮空数集,如果按照某种确定的对应关系f ,使对于集合A 中的任意⼀个数x ,在集合B 中都有唯⼀确定的数()f x 和它对应,那么称:f A B →为从集合A 到集合B 的⼀个函数(function ),记作:(),y f x x A =∈.其中,x 叫⾃变量,x 的取值范围A 叫作定义域(domain ),与x 的值对应的y 值叫函数值,函数值的集合{()|}f x x A ∈叫值域(range ).④讨论:值域与B 的关系?构成函数的三要素?⼀次函数(0)y ax b a =+≠、⼆次函数2(0)y ax bx c a =++≠的定义域与值域?⑤练习:2()23f x x x =-+,求f(0)、f(1)、f(2)、f(-1)的值。
博途教育学科教师辅导讲义(一)学员姓名: 年级:高一日期:辅导科目:数学学科教师:刘云丰时间:课题第十一讲:函数模型及其应用授课日期教学目标1、培养学生根据实际问题进行信息综合列出函数解析式;2、会利用函数图象性质对函数解析式进行处理得出数学结论.教学内容函数模型及其应用〖教学重点与难点〗◆教学重点:根据实际问题分析建立数学模型和根据实际问题拟合判断数学模型;◆教学难点:根据数学模型解决实际问题。
〖教学过程〗一、创设情境,导入课题在课本第三章的章头图中,有一大群喝水、嬉戏的兔子,但是这群兔子曾使澳大利亚伤透了脑筋.1859年,有人从欧洲带进澳洲几只兔子,由于澳洲有茂盛的牧草,而且没有兔子的天敌,兔子数量不断增加,不到100年,兔子们占领了整个澳大利亚,数量达到75亿只.可爱的兔子变得可恶起来,75亿只兔子吃掉了相当于75亿只羊所吃的牧草,草原的载畜率大大降低,而牛羊是澳大利亚的主要牲口.这使澳大利亚人头痛不已,他们采用各种方法消灭这些兔子,直至二十世纪五十年代,科学家采用载液瘤病毒杀死了百分之九十的野兔,澳大利亚人才算松了一口气.这段话道出了其中的意蕴:对于一个种群的数量,如果在理想状态(如没有天敌、食物充足等)下,那么它将呈指数增长;但在自然状态下,种群数量一般符合对数增长模型.二、提出问题,探索新知①我市有甲、乙两家乒乓球俱乐部,两家设备和服务都很好,但收费方式不同.甲家每张球台每小时5元;乙家按月计费,一个月中30小时以内(含30小时)每张球台90元,超过30小时的部分每张球台每小时2元.小张准备下个月从这两家中的一家租一张球台开展活动,其活动时间不少于15小时,也不超过40小时.设在甲家租一张球台开展活动x小时的收费为f(x)元(15≤x≤40),在乙家租一张球台开展活动x小时的收费为g(x)元(15≤x≤40),试求f(x)和g(x).②A 、B 两城相距100 km ,在两地之间距A 城x km 处D 地建一核电站给A 、B 两城供电,为保证城市安全.核电站距城市距离不得少于10 km.已知供电费用与供电距离的平方和供电量之积成正比,比例系数λ=0.25.若A 城供电量为20亿度/月,B 城为10亿度/月. 把月供电总费用y 表示成x 的函数,并求定义域.③分析以上实例属于那种函数模型. 讨论结果:①f(x)=5x(15≤x ≤40).g(x)=⎩⎨⎧≤<+≤≤4030,902,3015,90x x x②y=5x 2+25(100—x)2(10≤x ≤90);③分别属于一次函数模型、二次函数模型、分段函数模型.三、应用示例例1 一辆汽车在某段路程中的行驶速率与时间的关系如图所示. (1)求图3-2-2-1中阴影部分的面积,并说明所求面积的实际含义;(2)假设这辆汽车的里程表在汽车行驶这段路程前的读数为2004km,试建立行驶这段路程时汽车里程表读数s km 与时间t h 的函数解析式,并作出相应的图象.图3-2-2-1活动:学生先思考或讨论,再回答.教师根据实际,可以提示引导:图中横轴表示时间,纵轴表示速度,面积为路程;由于每个时间段速度不断变化,汽车里程表读数s km 与时间t h 的函数为分段函数.解:(1)阴影部分的面积为50×1+80×1+90×1+75×1+65×1=360. 阴影部分的面积表示汽车在这5小时内行驶的路程为360 km.(2)根据图,有s=⎪⎪⎪⎩⎪⎪⎪⎨⎧≤≤+-<≤+-<≤+-<≤+-<≤+.54,2299)4(65,43,2224)3(75,32.2134)2(90,21,2054)1(80,10,200450t t t t t t t t t t这个函数的图象如图3-2-2-2所示.图3-2-2-2变式训练电信局为了满足客户不同需要,设有A 、B 两种优惠方案,这两种方案应付话费(元)与通话时间(分钟)之间关系如下图(图3-2-2-3)所示(其中MN ∥CD).(1)分别求出方案A 、B 应付话费(元)与通话时间x(分钟)的函数表达式f(x)和g(x);(2)假如你是一位电信局推销人员,你是如何帮助客户选择A 、B 两种优惠方案?并说明理由.图3-2-2-3解:(1)先列出两种优惠方案所对应的函数解析式:f(x)=⎪⎩⎪⎨⎧>-≤≤,100,10103,1000,20x x x g(x)=⎪⎩⎪⎨⎧>-≤≤.500,100103,5000,50x x x(2)当f(x)=g(x)时,103x-10=50, ∴x=200.∴当客户通话时间为200分钟时,两种方案均可;当客户通话时间为0≤x <200分钟,g(x)>f(x),故选择方案A ; 当客户通话时间为x>200分钟时,g(x)<f(x),故选方案B.点评:在解决实际问题过程中,函数图象能够发挥很好的作用,因此,我们应当注意提高读图的能力.另外,本例题用到了分段函数,分段函数是刻画现实问题的重要模型.例 2 人口问题是当今世界各国普遍关注的问题.认识人口数量的变化规律,可以为有效控制人口增长提供依据.早在1798年,英国经济学家马尔萨斯(T.R.Malthus,1766~1834)就提出了自然状态下的人口增长模型: y=y 0e rt ,其中t 表示经过的时间,y 0表示t=0时的人口数,r 表示人口的年平均增长率. 下表是1950~1959年我国的人口数据资料:年份 1950 1951 1952 1953 1954 1955 1956 1957 1958 1959 人数/万人 55196 56300 57482 58796 60266 61456 62828 64563 65994 67207(1)如果以各年人口增长率的平均值作为我国这一时期的人口增长率(精确到0.000 1),用马尔萨斯人口增长模型建立我国在这一时期的具体人口增长模型,并检验所得模型与实际人口数据是否相符;(2)如果按表的增长趋势,大约在哪一年我国的人口达到13亿?解:(1)设1951~1959年的人口增长率分别为r1,r2,r3,…,r9.由55196(1+r1)=56300,可得1951年的人口增长率为r1≈0.020 0.同理,可得r2≈0.0210,r3≈0.0229,r4≈0.0250,r5≈0.0197,r6≈0.0223,r7≈0.0276,r 8≈0.0222,r9≈0.0184.于是,1950~1959年期间,我国人口的年平均增长率为r=(r1+r2+…+r9)÷9≈0.0221.令y=55 196,则我国在1951~1959年期间的人口增长模型为y=55 196e0.0221t,t∈N.根据表中的数据作出散点图,并作出函数y=55 196e0.0221t(t∈N)的图象(图3-2-2-4).图3-2-2-4由图可以看出,所得模型与1950~1959年的实际人口数据基本吻合.(2)将y=130000代入y=55 196e0.0221t,由计算器可得t≈38.76.所以,如果按表的增长趋势,那么大约在1950年后的第39年(即1989年)我国的人口就已达到13亿.由此可以看到,如果不实行计划生育,而是让人口自然增长,今天我国将面临难以承受的人口压力.变式训练一种放射性元素,最初的质量为500 g,按每年10%衰减.(1)求t年后,这种放射性元素质量ω的表达式;(2)由求出的函数表达式,求这种放射性元素的半衰期(剩留量为原来的一半所需的时间叫做半衰期).(精确到0.1.已知lg2=0.301 0,lg3=0.477 1)解:(1)最初的质量为500 g.经过1年后,ω=500(1-10%)=500×0.91;经过2年后,ω=500×0.9(1-10%)=500×0.92;由此推知,t年后,ω=500×0.9t.(2)解方程500×0.9t=250,则0.9t=0.5,所以t=9.0lg 5.0lg =13lg 22lg --≈6.6(年), 即这种放射性元素的半衰期约为6.6年.知能训练某电器公司生产A 型电脑.1993年这种电脑每台平均生产成本为5 000元,并以纯利润20%确定出厂价.从1994年开始,公司通过更新设备和加强管理,使生产成本逐年降低.到1997年,尽管A 型电脑出厂价仅是1993年出厂价的80%,但却实现了50%纯利润的高效益. (1)求1997年每台A 型电脑的生产成本;(2)以1993年的生产成本为基数,求1993年至1997年生产成本平均每年降低的百分数.(精确到0.01,以下数据可供参考:5=2.236,6=2.449)活动:学生先思考或讨论,再回答.教师根据实际,可以提示引导. 出厂价=单位商品的成本+单位商品的利润.解:(1)设1997年每台电脑的生产成本为x 元,依题意,得 x(1+50%)=5000×(1+20%)×80%,解得x=3200(元).(2)设1993年至1997年间每年平均生产成本降低的百分率为y,则依题意,得5000(1-y)4=3200, 解得y 1=1-552,y 2=1+552(舍去). 所以y=1-552≈0.11=11%, 即1997年每台电脑的生产成本为3 200元,1993年至1997年生产成本平均每年降低11%. 点评:函数与方程的应用是本章的重点,请同学们体会它们的关系. 拓展提升某家电企业根据市场调查分析,决定调整产品生产方案,准备每周(按120个工时计算)生产空调、彩电、冰箱共360台,且冰箱至少生产60台.已知生产这些家电产品每台所需工时和每台产值如下表:家电名称 空调 彩电 冰箱每台所需工时21 31 41 每台产值(千元) 4 32 问每周应生产空调、彩电、冰箱各多少台,才能使周产值最高?最高产值是多少?(以千元为单位) 解:设每周生产空调、彩电、冰箱分别为x 台、y 台、z 台,每周产值为f 千元, 则f=4x+3y+2z ,其中⎪⎩⎪⎨⎧≥≥≥=++=++)3(,60,0,0)2(,120413121)1(,360z y x z y x z y x由①②可得y=360-3x,z=2x,代入③得⎪⎩⎪⎨⎧≥≥-≥,602,03360,0x x x 则有30≤x ≤120.故f=4x+3(360-3x)+2·2x=1080-x, 当x=30时,f max =1 080-30=1050. 此时y=360-3x=270,z=2x=60.答:每周应生产空调30台,彩电270台,冰箱60台,才能使每周产值最高,最高产值为1 050千元.点评:函数方程不等式有着密切的关系,它们相互转化组成一个有机的整体,请同学们借助上面的实例细心体会.四、课堂小结本节重点学习了函数模型的实例应用,包括一次函数模型、二次函数模型、分段函数模型等;另外还应关注函数方程不等式之间的相互关系.五、课后练习1.按复利计算利率的储蓄,银行整存一年,年息8%,零存每月利息2%,现把2万元存入银行3年半,取出后本利和应为人民币( ) A . 3.52(18%)+万元 B .362(18%)(12%)++万元 C .32(18%)22%5++⨯⨯万元D .3362(18%)2(18%)(12%)++⨯++万元解析:3年半本利和的计算问题,应转为3年按年息8%计算,而半年按6个月(月息2%)计算,又由于是复利问题,故只有选B .2.某学生离家去学校,为了锻炼身体,一开始跑步前进,跑累了再走余下的路,下图中,纵轴表示离学校的距离,横轴表示出发后的时间,则下列四个图形中较符合该生走法的是( )解析:由于d表示学生的家与学校的距离,因而首先排除A、C选项,又因为图中线段的斜率的绝对值表示前进速度的大小,因而排除B,故只能选择D。
三角函数的定义及应用教学教案【优秀4篇】(经典版)编制人:__________________审核人:__________________审批人:__________________编制单位:__________________编制时间:____年____月____日序言下载提示:该文档是本店铺精心编制而成的,希望大家下载后,能够帮助大家解决实际问题。
文档下载后可定制修改,请根据实际需要进行调整和使用,谢谢!并且,本店铺为大家提供各种类型的经典范文,如总结报告、心得体会、应急预案、演讲致辞、合同协议、规章制度、条据文书、教学资料、作文大全、其他范文等等,想了解不同范文格式和写法,敬请关注!Download tips: This document is carefully compiled by this editor. I hope that after you download it, it can help you solve practical problems. The document can be customized and modified after downloading, please adjust and use it according to actual needs, thank you!Moreover, our store provides various types of classic sample essays, such as summary reports, insights, emergency plans, speeches, contract agreements, rules and regulations, documents, teaching materials, complete essays, and other sample essays. If you would like to learn about different sample formats and writing methods, please pay attention!三角函数的定义及应用教学教案【优秀4篇】EXcel中经常需要使用到三角函数进行计算,三角函数具体该如何使用呢?它山之石可以攻玉,以下内容是本店铺为您带来的4篇《三角函数的定义及应用教学教案》,如果对您有一些参考与帮助,请分享给最好的朋友。
函数的实际应用教案一、教学目标通过本教案的学习,学生应能够:1.了解函数的概念及其在数学和实际生活中的应用;2.掌握函数的定义和表示方法;3.学会解决实际问题时使用函数进行建模和求解。
二、教学重点1.函数的定义和表示方法;2.函数在实际问题中的应用。
三、教学难点1.函数的实际应用;2.使用函数进行建模和求解实际问题。
四、教学过程Step 1 引入1.引导学生回顾函数的定义:函数是一种对应关系,它将一个集合的每个元素与另一个集合的唯一元素相对应。
2.通过几个简单的例子,让学生了解函数的基本概念,并引发学生对函数在实际生活中的应用的思考。
Step 2 函数的表示方法1.介绍函数的表示方法:函数可以用方程、表格和图像来表示。
2.通过具体的例子,让学生了解不同表示方法之间的转换关系,并掌握如何将方程、表格和图像互相转换。
Step 3 函数在实际问题中的应用1.引导学生思考函数在实际问题中的应用,比如数学建模、物理问题、经济问题等。
2.通过一些实际问题的例子,让学生体会到函数在实际生活中的重要性,并了解如何将实际问题转化为函数的形式进行求解。
Step 4 使用函数进行建模和求解问题1.讲解如何使用函数进行建模:根据实际问题中的条件和要求,选择适当的变量和函数形式来建立数学模型。
2.通过一些综合性的例子,让学生掌握使用函数进行建模的方法和技巧,并学会通过求解函数来解决实际问题。
Step 5 练习与拓展1.设计一些练习题,让学生运用所学知识解决实际问题;2.引导学生思考更多的实际问题,并尝试用函数进行建模和求解。
五、教学评价1.观察学生在课堂中的表现,包括参与讨论的积极性、解决问题的能力等;2.布置作业,检查学生对函数实际应用的理解和运用能力。
六、教学反思通过本节课的教学,学生对函数的实际应用有了更深入的了解。
在教学过程中可以通过实际问题的引入,让学生深入体验函数在解决实际问题中的作用,培养学生的数学思维和建模能力。
精心整理高一数学必修一教案《函数模型及其应用》【篇一】【内容】建立函数模型刻画现实问题量的原始数据的处理,这可能会用到电脑和计算器以及图形工具,而我们的教学应更加关注的是通过实际问题的分析过程来选择适当的函数模型和函数模型的构建过程。
在这个过程中,要使学生着重体会的是模型的建立,同时体会模型建立的可操作性、有效性等特点,学习模型的建立以解决实际问题,培养发展有条理的思维和表达能力,提高逻辑思维能力。
【教学目标】(1)体现建立函数模型刻画现实问题的基本过程.态度了解函处理生的小组合作探究来突破本节课的难点,这样,在小组合作学习与探究过程中实现教学目标中对知识和能力的要求(目标1,2,3)在如何用函数建模刻画现实问题的基本过程中让学生亲身体验函数应用的广泛性,同时提高学生探究学习新知识的兴趣,培养学生主动参与、自主学习、勇于探索的科学态度,从而实现教学目标中的德育目标(目标4)【学生学习中预期的问题及解决方案预设】①描点的规范性;②实际操作的速度;③解析式的计算速度④计算结束后不进行检验这样以.教学前言:函数模型是应用最广泛的数学模型之一,许多实际问题一旦认定是函数关系,就可以通过研究函数的性质把握问题,使问题得到解决.教学内容师生活动设计意图探究新知引入:教师:大家觉得我胖吗?学生回答教师:我们在街上见到一个人总是会判断这个人的胖瘦,我们衡来衡量BMI 大于学生说,教师把相关数据填在用PPT展示的一张表格上教师:好,有了这些数据我们就可以来研究了,那接下来我们怎么来处理刚收集到的这些数据呢?学生回答(预期:画散点图——连线——找函数)教师:好,大家按小组先画图连线然后讨论一下你们小组认为哪个函数的图像符合学生活动并回答教师:好,那大家分一下工,你们几个小组来计算这个函数解析呢?教师:那大家来检验一下哪个模型更符合数据情况学生分小组进行检验教师:好了,我们利用刚才收集的数据通过我们的努力得出了一个式子,它也就是符合大家的情况的一个胖瘦的标准,既是我们班的一个标准,能用来衡量其它班的同学吗?那我们来计算一下老师的结果是什么样的.教师:可见用世界肥胖标准对老师的体重进行的评价和所建立的数学模型计算的结果是基本一致的。
Modeling and Problem Solving——函数模型及其应用教案中澳课程部王晓叶学情分析:澳方MathB每次的Paper Test都分为两部分,其中Knowledge and Procedures(知识与过程)这个和普通高中数学相似,学生A/B率比较高,但是另外一部分Modeling and Problem Solving(建模与实际问题的解决)学生的A/B率不高。
这一部分内容题目普遍很长、生词量较多,并且都是将数学知识应用于实际生活中,所以大多数学生遇到此类题目都是放弃不做。
MathB这门课又特别注重实际生活问题的解决,而我们的学生这方面意识比较薄弱,抽象概括能力较弱。
所以,我们的教学任务是提高学生的考试成绩等级,提高OP成绩。
但是另一方面,12年级的学生大多数能灵活的使用图形计算器,具有一定的英语语言基础。
教学目标:1.了解函数模型在现实生活中的运用。
2.能够建立恰当的函数模型,并对函数模型进行简单的分析。
3.利用所得函数模型解释有关现象,对某些发展趋势进行预测。
教学重难点:1.建立合适的函数模型2.利用得到的函数模型解决实际问题教学过程一、引入案例、探索新知(如何确定最合适的函数模型)(18分钟)案例:根据《Daily Mail》报道,上个月一名中国留学生将自己车速飙到180公里/小时的录像传到了Instagram个人网页上,并以配以中文:“从Albany开回Perth,一路180公里/小时,将4.5小时的车程缩短到3.5小时。
”目前,他正在接受警方调查。
警察表示,视频显示这名男子在限速110公里/小时的高速公路开到了180公里/小时,他将面临巨额罚款、吊销驾照以及拘留。
Example1:The table below shows the relationship between the velocity of a car and theVelocity 10 20 30 40 50 60 70 80 90 Distance 2 10 15 20 27 38 47 60 75a. Use the calculator to find the relationship between the velocity of a car and the distance after it braking.b. What’s the minimum safe following distance for a car travelling at 110 km/h on the motor way?项目罚款扣分超速少于10km/h 163澳元扣2分超速10km/h-20km/h 357澳元扣3分超速20km/h-30km/h 726澳元扣5分超速30km/h-40km/h 866澳元扣7分未系安全带341澳元扣3分闯红灯437澳元扣3分开车使用手机315澳元扣3分(设计意图:从生活案例引入新知,激发学生的学习兴趣。
从简单题目入手,目的是让学生掌握图形计算器的使用,能够利用图形计算器建立合适的函数模型,为解决函数模型的应用做铺垫。
同时在课堂中渗透德育内容,让学生知法懂法守法。
)小结:如何建立合适的函数模型?•Solve the practical problemExercise.Some Chemistry students measured the concentration of chlorine remaining in a swimming pool over a period of 8 hours on a hot summer day. Chlorine had been placed in theMorning AfternoonTime 9 10 11 12 1 2 3 4 Chlorine concentration(ppm) 5.0 3.8 2.9 2.2 1.6 1.2 0.9 0.7a. Develop a model for the data。
b. What’s the concentration of chlorine at 8 am?(设计意图:通过练习,巩固加强掌握图形计算器的使用,为下一个例题的讲解做好铺垫。
)二、例题精讲(函数模型的应用)(12分钟)A/B Standard-Modeling and Problem SolvingExample2.Some Chemistry students measured the concentration of chlorine remaining in a swimming pool over a period of 8 hours on a hot summer day. Chlorine had been placed in theMorning AfternoonTime 9 10 11 12 1 2 3 4 Chlorine concentration(ppm) 5.0 3.8 2.9 2.2 1.6 1.2 0.9 0.7e the calculator to find the relationship between the chlorine concentration and the timeelapsed since the chlorine was placed in the pool.e the results to find the concentration that would be needed at 8 am on a similar day toensure that the chlorine concentration did not fall below 1.5ppm(parts per million) before 3 pm.3.If two chlorine doses were used , one at 8 am and another at 12 noon, what concentrationswould be needed at these times to ensure that the concentration did not fall below 1.5 ppm before 3 pm.4.What are the implication of your answers for the effective chlorination of poolsat lowest cost?(设计意图:例题为11年级MathB一次大考中的一道原题,是一道A/B等级的题目,大多数学生遇到此类型的题目都是放弃不做,原因是题目太长,生词太多,难度较大。
针对这些问题,所以通过前面的练习让学生看懂题目,拟合出合适的函数模型。
精解函数模型的应用。
首先是引导学生审题,找关键词,读懂题目。
又将题目分解成多个小题,层层递进,由易到难,引导学生学会解决此类问题的方法。
遇到大的困难时,先一个一个小困难的解决。
)三、课堂检测(14分钟)1. An oscilloscope is used by students to measure the voltage across a capacitor as it discharges through a large resistance. Observations made by the students were as follows.Time(s) 4 8 12 20 30 40V oltage 49 40 33 22 13 8b. Use yours results to predict the voltage after 2 min.2.The removal of some substances from the blood by the kidneys depends on the concentration in the blood. The following measurements of the concentration of an antibiotic were taken after theTime (hours) 0.5 1 1.5 2 2.5 3 Concentration(ppm) 18 16 14 13 11 10a. Develop a model for the data。
b. For the drug to be effective, it is known that the concentration must be between 5 and 20ppm. How often must a follow-up dose be given?(设计意图:由于澳方核心能力考试强调学科间的结合,所以例题涉及化学,课堂练习两道题目涉及分别涉及物理、生物医药。
目的是为了检测本节课学习成果,检测学生的掌握程度。
题目难度比例题要低一些,确保大多数同学都能完成,增强信心,第二题的第二个小问稍微有些难度,题目由易到难。
)四:总结(1分钟)五、课后练习:1.People from the country say that the pace of city is too fast. They claim that people in the bigcities walk faster than those in smaller towns. Marc and Helen Bornstern collected data on theLocation Population Speed(m/s)Brooklyn,USA 2602000 1.54Munich,Germany 1340000 1.91Prague Czechoslovakia 1092759 1.79Jerusalem,Israel 304500 1.35New Haven,USA 138000 1.34Iraklion,Greece 78200 1.17Netanya,Israel 70700 1.31Bastia,France 49375 1.49Dimona,Israel 23700 1.00Safed,Israel 14000 1.13Corte,Corsica 5491 1.01Itea,Greece 2500 0.69Psychro,Crete 365 0.84Athens,Greece 867023 1.59Brno,Czechoslovakia 341948 1.47a. Develop a model for the datab. Use your results to predict the speed of walkers in Toowoomba, which has a population of about100000.2. In 1798,Thomas Malthus suggested that exponential growth of the human population musteventually outstrip the possible food supply and lead to wars and disease.a.Investigate the records of word population given above to find whether you agree with theYear 1000 1200 1400 1500 1600 1700 1750 1800 1850 1900 1950 2000Population 350 435 465 475 490 630 800 900 1300 1600 2500 6000 idea that it does grow exponentially.b.Malthus originally suggested that the world’s food supply would have been outstripped longbefore the present day. Suggest some reasons to explain the fact that his dire predictions haveat least been delayed.。